Comparison of Unlike Fractions
In comparison of unlike fractions, we change the unlike fractions to like fractions and then compare.
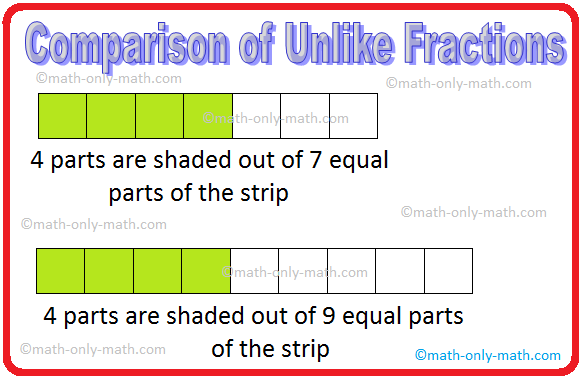
Let us compare two fractions \(\frac{4}{7}\) and \(\frac{4}{9}\) which have same numerator.
Since 4 shaded parts of 7 is bigger than the 4 shaded parts of 9 therefore \(\frac{4}{7}\) > \(\frac{4}{9}\).
To compare
two fractions with different numerators and different denominators, we multiply
by a number to convert them to like fractions.
Let us consider some of the examples on comparing fractions
(i.e. unlike fractions).
1. Which one is greater, \(\frac{4}{7}\) or \(\frac{3}{5}\)?
First we convert these fractions into like fractions. To convert unlike fraction into like fraction first of all find the L.C.M. of their denominators.
L.C.M. of 7 and 5 = 35
Now, divide this L.C.M. by the denominator of both the fractions.
35 ÷ 7 = 5
35 ÷ 5 = 7
Multiply both the numerator and denominator with the number you get after dividing.
i.e., \(\frac{4 × 5}{7 × 5}\) = \(\frac{20}{35}\)
\(\frac{3 × 7}{5 × 7}\) = \(\frac{21}{35}\)
because \(\frac{21}{35}\) > \(\frac{20}{35}\)
So, \(\frac{3}{5}\) > \(\frac{4}{7}\)
We can compare two fractions by cross multiplication also.
Let us solve the above example by cross multiplication. Here, we cross multiply as follows.
4 × 5 = 20
3 × 7 = 21
Since, 21 > 20
Therefore, \(\frac{3}{5}\) > \(\frac{4}{7}\)
2. Compare 3\(\frac{2}{5}\) and 2\(\frac{3}{4}\).
First we convert these mixed numbers into improper
fractions.
2\(\frac{3}{4}\) = \(\frac{4 × 2 + 3}{4}\) = \(\frac{11}{4}\)
3\(\frac{2}{5}\) = \(\frac{5 × 3 + 2}{5}\) = \(\frac{17}{5}\)
Now, we compare \(\frac{11}{4}\) and \(\frac{17}{5}\) by cross multiplication.
11 × 5 = 55 and 17 × 4 = 68
We see that 68 > 55.
Therefore, \(\frac{17}{5}\) > \(\frac{11}{4}\) or, 3\(\frac{2}{5}\) > 2\(\frac{3}{4}\)
3. Let us
compare \(\frac{5}{7}\) and \(\frac{3}{5}\).
\(\frac{5}{7}\)
= \(\frac{5 × 5}{7 × 5}\) = \(\frac{25}{35}\)
Multiply
the numerator and denominator by 5.
\(\frac{3}{5}\)
= \(\frac{3 × 7}{5 × 7}\) = \(\frac{21}{35}\)
Multiply
the numerator and denominator by 7.
Hence, \(\frac{25}{35}\)
> \(\frac{21}{35}\)
Therefore, \(\frac{5}{7}\)
> \(\frac{3}{5}\)
We will
learn an alternative method i.e. cross multiply to compare the given fractions.
4. Let us
compare \(\frac{2}{3}\) and \(\frac{4}{5}\).
2 × 5 = 10
and 3 × 4 = 12
Since, 12
> 10, hence \(\frac{4}{5}\) > \(\frac{2}{3}\)
You might like these
-
To add two or more like fractions we simplify add their numerators. The denominator remains same.
-
In worksheet on addition of fractions having the same denominator, all grade students can practice the questions on adding fractions. This exercise sheet on fractions can be practiced by the students to get more ideas how to add fractions with the same denominators.
-
In worksheet on subtraction of fractions having the same denominator, all grade students can practice the questions on subtracting fractions. This exercise sheet on fractions can be practiced by the students to get more ideas how to subtract fractions with the same
-
Addition and subtraction of like fractions. Addition of Like Fractions: To add two or more like fractions we simplify add their numerators. The denominator remains same. To subtract two or more like fractions we simply subtract their numerators and keep the same denominator.
-
Recall the topic carefully and practice the questions given in the math worksheet on add and subtract fractions. The question mainly covers addition with the help of a fraction number line, subtraction with the help of a fraction number line, add the fractions with the same
-
In 4th grade fractions worksheet we will circle the like fractions, circle the greatest fraction, arrange the fractions in descending order, arrange the fractions in ascending order, addition of like fractions and subtraction of like fractions.
-
We will discuss here how to arrange the fractions in ascending order. Solved examples for arranging in ascending order: 1. Arrange the following fractions 5/6, 8/9, 2/3 in ascending order. First we find the L.C.M. of the denominators of the fractions to make the denominators
-
Any two like fractions can be compared by comparing their numerators. The fraction with larger numerator is greater than the fraction with smaller numerator, for example \(\frac{7}{13}\) > \(\frac{2}{13}\) because 7 > 2. In comparison of like fractions here are some
-
Like and unlike fractions are the two groups of fractions: (i) 1/5, 3/5, 2/5, 4/5, 6/5 (ii) 3/4, 5/6, 1/3, 4/7, 9/9 In group (i) the denominator of each fraction is 5, i.e., the denominators of the fractions are equal. The fractions with the same denominators are called
-
In worksheet on equivalent fractions, all grade students can practice the questions on equivalent fractions. This exercise sheet on equivalent fractions can be practiced by the students to get more ideas to change the fractions into equivalent fractions.
-
We will discuss here about verification of equivalent fractions. To verify that two fractions are equivalent or not, we multiply the numerator of one fraction by the denominator of the other fraction. Similarly, we multiply the denominator of one fraction by the numerator
-
Equivalent fractions are the fractions having the same value. An equivalent fraction of a given fraction can be obtained by multiplying its numerator and denominator by the same number
-
In 5th Grade Fractions Worksheets we will solve how to compare two fractions, comparing mixed fractions, addition of like fractions, addition of unlike fractions, addition of mixed fractions, word problems on addition of fractions, subtraction of like fractions
-
Here we will learn Reciprocal of a fraction. What is 1/4 of 4? We know that 1/4 of 4 means 1/4 × 4, let us use the rule of repeated addition to find 1/4× 4. We can say that \(\frac{1}{4}\) is the reciprocal of 4 or 4 is the reciprocal or multiplicative inverse of 1/4
-
To divide a fraction or a whole number by a fraction or a whole number, we multiply the reciprocal of the divisor. We know that the reciprocal or the multiplicative inverse of 2 is \(\frac{1}{2}\).
Related Concept
● Fraction
of a Whole Numbers
● Representation
of a Fraction
● Equivalent
Fractions
● Properties
of Equivalent Fractions
● Like and
Unlike Fractions
● Comparison
of Like Fractions
● Comparison
of Fractions having the same Numerator
● Types of
Fractions
● Changing Fractions
● Conversion
of Fractions into Fractions having Same Denominator
● Conversion
of a Fraction into its Smallest and Simplest Form
● Addition
of Fractions having the Same Denominator
● Subtraction
of Fractions having the Same Denominator
● Addition
and Subtraction of Fractions on the Fraction Number Line
4th Grade Math Activities
From Comparison of Unlike Fractions to HOME PAGE
Didn't find what you were looking for? Or want to know more information
about Math Only Math.
Use this Google Search to find what you need.
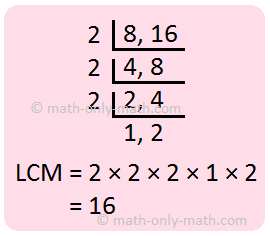
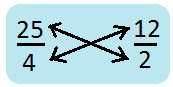
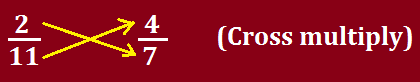
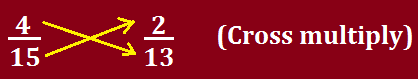
New! Comments
Have your say about what you just read! Leave me a comment in the box below. Ask a Question or Answer a Question.