Verification of Equivalent Fractions

We will discuss here about verification of equivalent fractions. To verify that two fractions are equivalent or not, we multiply the numerator of one fraction by the denominator of the other fraction. Similarly, we multiply the denominator of one fraction by the numerator of the other fraction. If the products obtained, are the same, the fractions are equivalent.

Checking for Equivalence of Two Fractions:

We can check whether the two fractions are equivalent or not by cross multiplication.

If two fractions are equivalent, then

Numerator of the first × Denominator of the second = Denominator of the first Numerator of the second.

In other words, if fractions $\frac{a}{b}$ and $\frac{c}{d}$ are equivalent,

i.e., $\frac{a}{b}$ = $\frac{c}{d}$, then ad = cb

Consider the following examples.

1: Check whether the given fractions are equivalent or not:

(i) $\frac{3}{5}$, $\frac{6}{10}$

(ii) $\frac{5}{11}$, $\frac{20}{33}$

Solution:

(i) By cross multiplication, we have 3 × 10 = 30 and 5 × 6 = 30

Since two products are the same, the given fractions are equivalent.

(ii) By cross multiplication, we have 5 × 33 = 165 and 11 × 20 = 220

Since two products are not the same, the given fractions are not equivalent.

2. Test whether $\frac{4}{9}$ and $\frac{8}{18}$ are equivalent or not.

Here, 4 × 18 = 72              

(The product of the numerator of the first fraction and the denominator of the other)

9 × 8 = 72                        

(The product of the denominator of the first fraction and the numerator of the other)

Thus, $\frac{4}{9}$ and $\frac{8}{18}$ are equivalent fractions.

We can also verify equivalent fractions by reducing them to their lowest terms.

3. Verifying equivalent fractions:

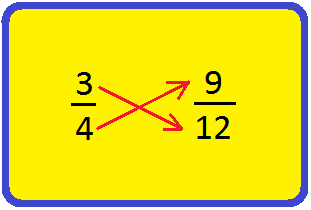
Consider two fractions $\frac{3}{4}$ and $\frac{9}{12}$.

Find the cross product as shown below.

3 × 12 Multiply the numerator of $\frac{3}{4}$ by the denominator of $\frac{9}{12}$

4 × 9 Multiply the denominator of $\frac{3}{4}$ by the numerator of $\frac{9}{12}$

We get 3 × 12 = 4 × 9

              36    =    36

Hence, the two fractions are equivalent if their cross products are equal.

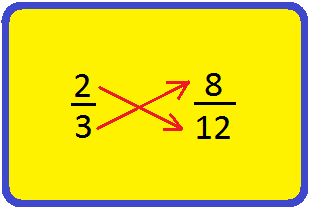
4. Verify if $\frac{2}{3}$ and $\frac{8}{12}$ are equivalent.

Multiplying numbers across fractions. 2 × 12 = 24 and 3 × 8 = 24 both the products are equal. Hence, $\frac{2}{3}$ and $\frac{8}{12}$ are equivalent fractions.

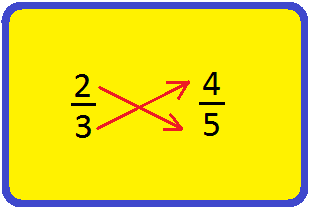
5. Verify if $\frac{2}{3}$ and $\frac{4}{5}$ are equivalent.

Multiplying numbers across fractions. 2 × 5 = 10 and 3 × 4 = 12 Cross products are not equal. Hence, $\frac{2}{3}$ and $\frac{4}{5}$ are not equivalent fractions.

6. Test whether $\frac{2}{3}$, $\frac{10}{15}$ and $\frac{22}{33}$ are equivalent or not.

We express the above fractions to their lowest terms.

$\frac{2}{3}$ is itself in its lowest terms.   (The H.C.F. of 2 and 3 is 1)

$\frac{10}{15}$ = $\frac{10 ÷ 5}{15 ÷ 5}$ = $\frac{2}{3}$ and $\frac{22}{33}$ = $\frac{22 ÷ 11}{33 ÷ 11}$ = $\frac{2}{3}$

Because $\frac{2}{3}$, $\frac{10}{15}$ and $\frac{22}{33}$ have the same value. So, they are equivalent fractions.

Related Concept

● Fraction of a Whole Numbers

● Representation of a Fraction

● Equivalent Fractions

● Properties of Equivalent Fractions

● Like and Unlike Fractions

● Comparison of Like Fractions

● Comparison of Fractions having the same Numerator

● Types of Fractions

● Changing Fractions

● Conversion of Fractions into Fractions having Same Denominator

● Conversion of a Fraction into its Smallest and Simplest Form

● Addition of Fractions having the Same Denominator

● Subtraction of Fractions having the Same Denominator

● Addition and Subtraction of Fractions on the Fraction Number Line

4th Grade Math Activities

From Verification of Equivalent Fractions to HOME PAGE