Fractions in Descending Order
We will discuss here how to arrange the fractions in descending order.
Solved examples for arranging in descending order:
1. Arrange the following fractions 56, 710, 1120 in descending order.
First we find the L.C.M. of the denominators of the fractions to make the denominators same.
L.C.M. of 6, 10 and 20 = 2 × 5 × 3 × 1 × 2 = 60
56 = 5×106×10 = 5060 (because 60 ÷ 6 = 10)
710 = 7×610×6 = 4260 (because 60 ÷ 10 = 6)
1120 = 11×320×3 = 3360 (because 60 ÷ 20 = 3)
Now we compare the like fractions 5060, 4260 and 3360
Comparing numerators, we find that 50 > 42 > 33.
Therefore, 5060 > 4260 > 3360 or 56 > 710 > 1120
The descending order of the fractions is 56, 710, 1120.
2. Arrange the following fractions 12, 34, 78, 512 in
descending order.
First we find the L.C.M. of the denominators of the fractions to make the denominators same.
L.C.M. of 2, 4, 8 and 12 = 24
12 = 1×122×12 = 1224 (because 24 ÷ 2 = 12)
34 = 3×64×6 = 1824 (because 24 ÷ 10 = 6)
78 = 7×38×3 = 2124 (because 24 ÷ 20 = 3)
512 = 5×212×2 = 1024 (because 24 ÷ 20 = 3)
Now we compare the like fractions 1224, 1824, 2124 and 1024.
Comparing numerators, we find that 21 > 18 > 12 > 10.
Therefore, 2124 > 1824 > 1224 > 1024 or 78 > 34 > 12 > 512
The descending order of the fractions is 78 > 34 > 12 > 512.
3. Arrange the following fractions in descending order of magnitude.
|
3×184×18 = 5472 Therefore, 34 = 5472 |
5×98×9 = 4572 Therefore, 58 = 4572 |
|
4×126×12 = 4872 Therefore, 46 = 4872 |
2×89×8 = 1672 Therefore, 29 = 1672 |
Descending order: 5472, 4872, 4572, 1672
i.e., 34, 46, 58, 29
4. Arrange the following fractions in descending order of magnitude.
412, 312, 514, 116, 214
Observe the whole numbers.
4, 3, 5, 1, 2
1 < 2 < 3 < 4 < 5
Therefore, descending order: 514, 412, 312, 214, 116
5. Arrange the following fractions in descending order of magnitude.
314, 312, 216, 414, 819
Observe the whole numbers.
3, 3, 2, 4, 8
Since the whole number part of 314 and 312 are same, compare them.
Which is bigger? 314 or 312? 14 or 12?
L.C.M. of 4, 2 = 4
1×14×1 = 14 1×22×2 = 24
Therefore, 314 = 314 312 = 324
Therefore, 324 > 314 i.e., 312 > 314
Therefore, descending order: 819, 434, 312, 314, 216
Worksheet on Fractions in Descending Order:
Comparison of Like Fractions:
1. Arrange the given fractions in descending order:
(i) 727, 1027, 1827, 2127
(ii) 1539, 739, 1039, 2639
Answers:
1. (i) 2127, 1827, 1027, 727
(ii) 2639, 1539, 1039, 739
2. Arrange the following fractions in descending order of magnitude:
(i) 523, 1223, 423, 1723, 4523, 3623
(ii) 1317, 1217, 1117, 1617
Answers:
2. (i) 4523, 3623, 1723, 1223, 523
(ii) 1617 > 1317 > 1217 > 1117
Comparison of Unlike Fractions:
3. Arrange the following fractions in descending order:
(i) 16, 512, 23, 518
(ii) 34, 23, 43, 64, 12, 14
(iⅲ) 36, 34, 35, 38
(iv) 47, 67, 314, 521
Answers:
3. (1) 23 > 512 > 518 > 16
(ii) 64 > 43 > 34 > 23 > 12 > 14
(iⅲ) 34 > 35 > 36 > 38
(iv) 67 > 47 > 521 > 314
Related Concept
● Representation of a Fraction
● Properties of Equivalent Fractions
● Comparison of Like Fractions
● Comparison of Fractions having the same Numerator
● Conversion of Fractions into Fractions having Same Denominator
● Conversion of a Fraction into its Smallest and Simplest Form
● Addition of Fractions having the Same Denominator
● Subtraction of Fractions having the Same Denominator
● Addition and Subtraction of Fractions on the Fraction Number Line
4th Grade Math Activities
From Fractions in Descending Order to HOME PAGE
Didn't find what you were looking for? Or want to know more information about Math Only Math. Use this Google Search to find what you need.
Recent Articles
-
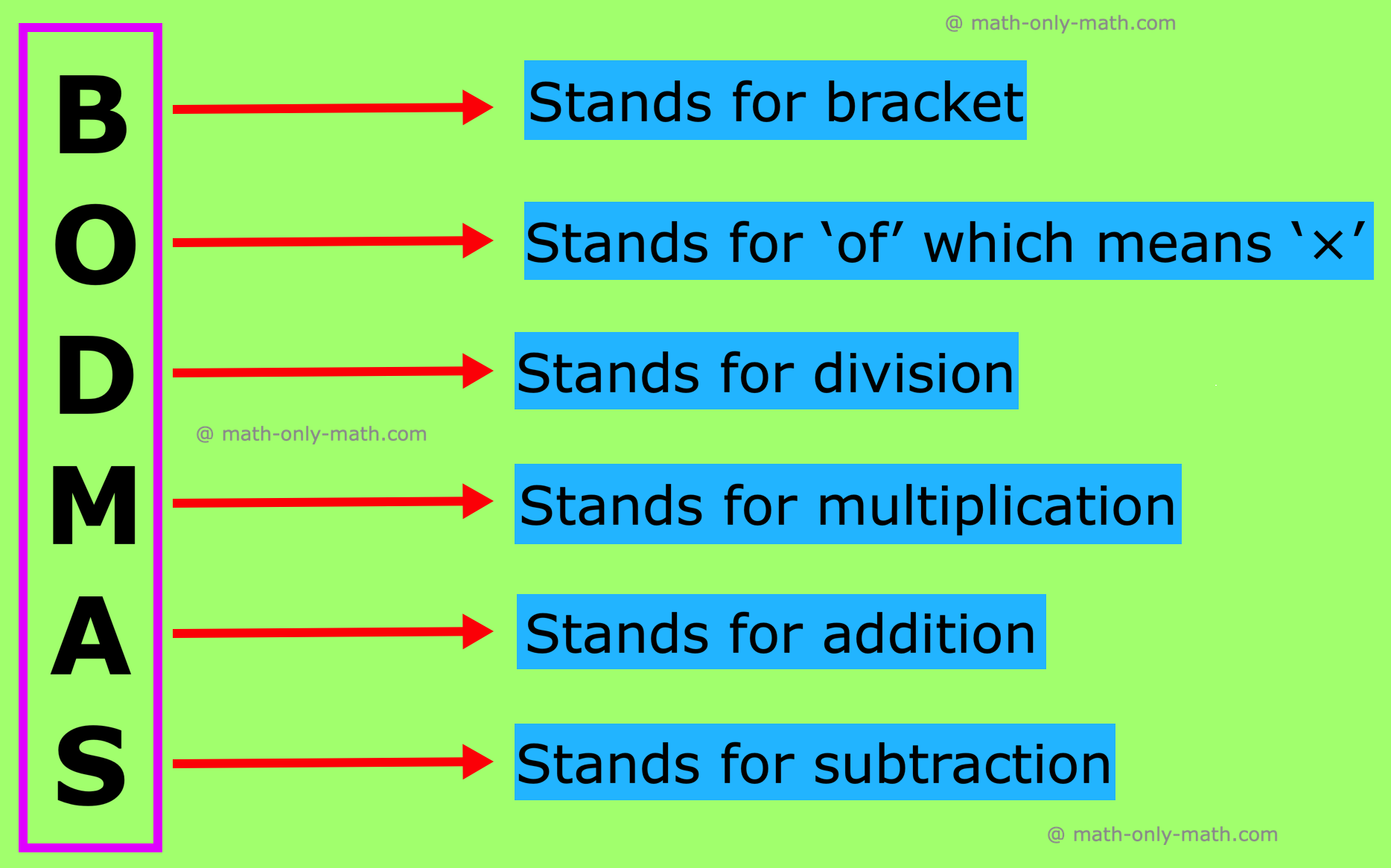
BODMAS Rule | Order of Operation | Definition, Examples, Problems
Mar 28, 25 02:17 AM
Easy and simple way to remember BODMAS rule!! B → Brackets first (parentheses) O → Of (orders i.e. Powers and Square Roots, Cube Roots, etc.) DM → Division and Multiplication -
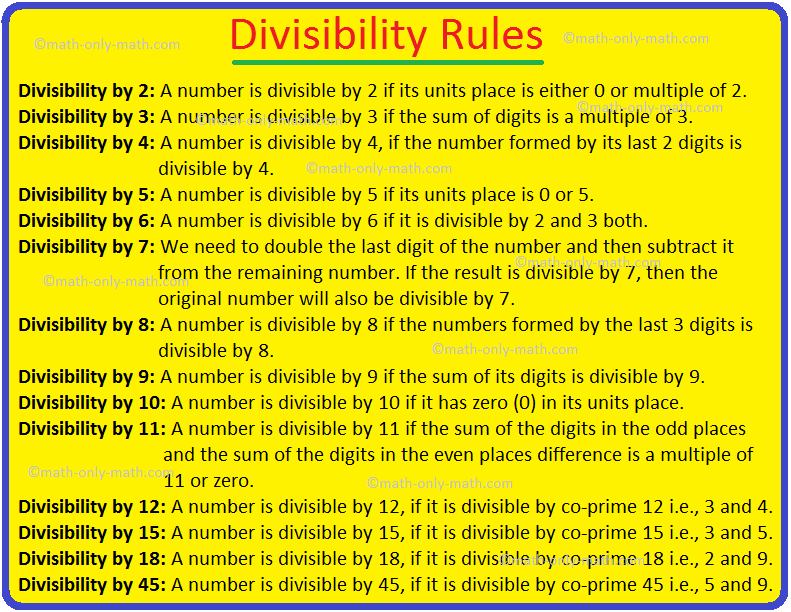
Divisibility Rules From 2 to 18 | Math Divisibility Test | Videos |
Mar 27, 25 01:04 PM
To find out factors of larger numbers quickly, we perform divisibility test. There are certain rules to check divisibility of numbers. Divisibility tests of a given number by any of the number 2, 3, 4… -
Divisible by 2 Video |Test of Divisibility by 2 Trick| Rules| Examples
Mar 27, 25 11:10 AM
A number is divisible by 2 if the digit at unit place is either 0 or multiple of 2. So a number is divisible by 2 if digit at its units place is 0, 2, 4, 6 or 8. -
Divisible by 3 | Test of Divisibility by 3 Trick | Rules | Video
Mar 27, 25 11:01 AM
A number is divisible by 3, if the sum of its all digits is a multiple of 3 or divisibility by 3. Consider the following numbers to find whether the numbers are divisible or not divisible by 3: (i) 54… -
Divisible by 4 | Test of Divisibility by 4 Trick | Rules | Video
Mar 27, 25 11:00 AM
A number is divisible by 4 if the number is formed by its digits in ten’s place and unit’s place (i.e. the last two digits on its extreme right side) is divisible by 4. Consider the following numbers…



















New! Comments
Have your say about what you just read! Leave me a comment in the box below. Ask a Question or Answer a Question.