Equivalent Ratios
Learn about equivalent ratios to get the clear concept on ratio. We know that we use ratios to compare numbers.
How do we find two equivalent ratios by using multiplication and division?
To get a ratio equivalent to a given ratio we multiply or divide both the terms of the given ratio by the same non-zero number. We will learn how to find the equivalent ratios of a given ratio by writing the ratio as a fraction and then compare by using multiplication and division.
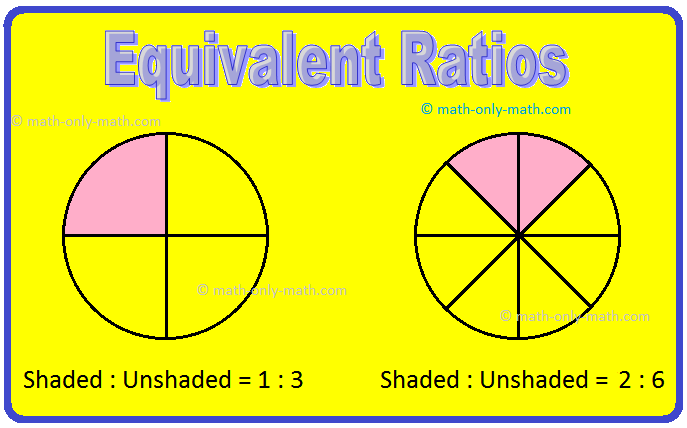
Look at the following figures
Also, 2 : 6 = 26 = 13 = 1 : 3
Hence, 1 : 3 and 2 : 6 are same ratio.
These are called equivalent ratios.
Solved Examples to Find Two Equivalent Ratios:
1. Give two equivalent ratios of 8 : 18.
Solution:
We will find the first equivalent ratio of 8 : 18 by using multiplication.
So, first we need to write the given
ratio as fraction,
= 818
= 8×218×2
= 1636
= 16 : 36 (one equivalent ratio),
So, 16 : 36 is an equivalent ratio of 8 : 18.
Now we will find another equivalent ratio of 8 : 18 by using division.
Similarly, first we need to write the given ratio as fraction,
= 818
= 8÷218÷2
= 49
= 4 : 9 (another equivalent ratio)
So, 4 : 9 is an equivalent ratio of 8 : 18.
Therefore, the two equivalent ratios of 8 : 18 are 16 : 36 and 4 : 9.
2. Frame two equivalent ratios of 4 : 5.
Solution:
To find two equivalent ratios of 4 : 5 we need to apply multiplication method only to get the answer in integer form.
First we need to write the given ratio as fraction,
= 45
= 4×25×2
= 810
= 8 : 10 is one equivalent ratio,
Similarly again, we need to write the given ratio 4 : 5 as fraction to get another equivalent ratio;
= 45
= 4×35×3
= 1215 is another equivalent ratio
Therefore, the two equivalent ratios of 4 : 5 are 8 : 10 and 12 : 15.
Note: In this question we can’t apply division method to get the answer in integer form because the G.C.F. of 4 and 5 is 1. That means, 4 and 5 cannot be divisible by any other number except 1.
3. For the following ratio find the two equivalent ratios of 11 : 13.
Solution:
To find two equivalent ratios of 11 : 13 first we need to write the given ratio as fraction,
1113
= 11×213×2
= 2226
= 22 : 26 is one equivalent ratio
Similarly again, to get another equivalent ratio of 11 : 13;
1113
= 11×413×4
= 4452
= 44 : 52 is another equivalent ratio
Therefore, the two equivalent ratios of 11 : 13 are 22 : 26 and 44 : 52.
Note: If a : b and x : y are two equivalent ratios, we write ab = xy.
Solved Examples to Find Three Equivalent Ratios:
1. Find three equivalent ratios of 3 : 8.
Solution:
3 : 8 = 38 = 3×28×2, [Multiply both numerator and denominator by 2]
= 616
= 6 : 16 is the first equivalent ratio.
3 : 8 = 38 = 3×48×4, [Multiply both numerator and denominator by 4]
= 1232
= 12 : 32 is the second equivalent ratio.
3 : 8 = 38 = 3×68×6, [Multiply both numerator and denominator by 6]
= 1848
= 18 : 48 is the third equivalent ratio.
Therefore, the three equivalent ratios of 3 : 8 are 6 : 16, 12 : 32 and 18 : 48.
2. Find any three equivalent ratios of 3 : 2.
Solution:
3 : 2 = 32 = 3×22×2 = 64 = 6 : 4
3 : 2 = 32 = 3×32×3 = 96 = 9 : 6
3 : 2 = 32 = 3×42×4 = 128 = 12 : 8
Hence, 6 : 4, 9 : 6 and 12 : 8 are three equivalent ratios of 3 : 2.
Worksheet on Equivalent Ratios:
1. Find two equivalent ratios of:
(i) 1 : 2
(ii) 5 : 3
(iii) 2 : 7
(iv) 3 : 8
Answer:
1. Find two equivalent ratios of:
(i) 2 : 4; 3 : 6
(ii) 10 : 6; 15 : 9
(iii) 4 : 14; 6 : 21
(iv) 6 : 16; 9 : 24
2. Fill in the blanks:
(i) 37 = 9⯀
(ii) 2 : 3 = 8 : ⯀
(iii) 25 = ⯀30
Answer:
2. (i) 21
(ii) 12
(iii) 12
From Equivalent Ratios to HOME PAGE
Didn't find what you were looking for? Or want to know more information about Math Only Math. Use this Google Search to find what you need.
Recent Articles
-
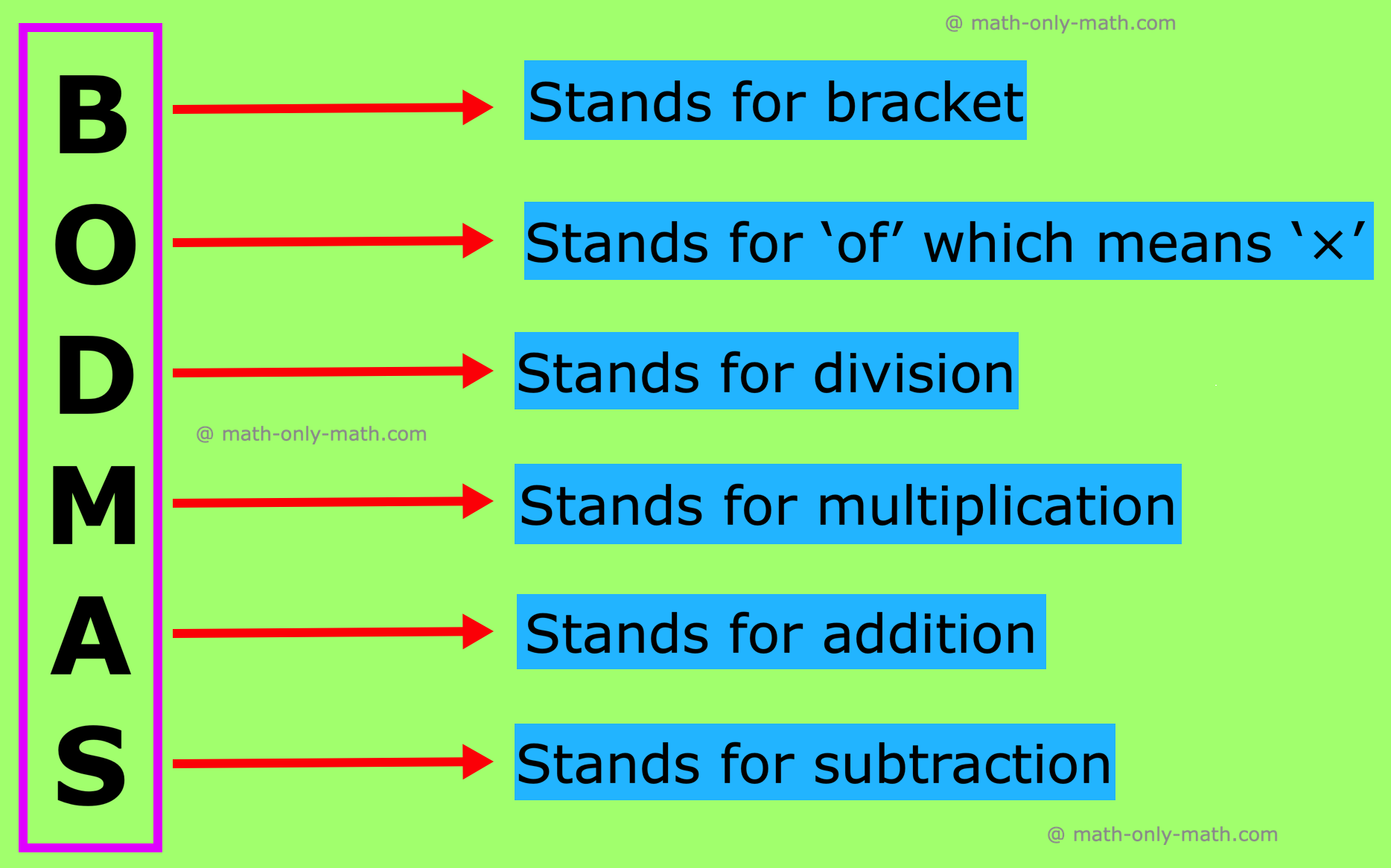
BODMAS Rule | Order of Operation | Definition, Examples, Problems
Mar 30, 25 01:11 PM
Easy and simple way to remember BODMAS rule!! B → Brackets first (parentheses) O → Of (orders i.e. Powers and Square Roots, Cube Roots, etc.) DM → Division and Multiplication -
Subtracting 1-Digit Number | Subtract Two One-Digit Number | Video
Mar 30, 25 10:16 AM
In subtracting 1-digit number we will subtract or minus one-digit number from one-digit number or one-digit number from 2-digit number and find the difference between them. We know that subtraction me… -
Divisible by 10 | Test of Divisibility by 10 Video | Rules | Examples
Mar 29, 25 03:06 PM
Divisible by 10 is discussed below. A number is divisible by 10 if it has zero (0) in its units place. Consider the following numbers which are divisible by 10, using the test of divisibility by 10: -
Divisible by 9 | Test of Divisibility by 9 | Rules | Video | Examples
Mar 29, 25 02:55 PM
A number is divisible by 9, if the sum is a multiple of 9 or if the sum of its digits is divisible by 9. Consider the following numbers which are divisible by 9, using the test of divisibility by 9: -
Divisible by 6 | Rules for Test of Divisibility by 6 Video | Examples
Mar 29, 25 02:48 PM
Divisible by 6 is discussed below: A number is divisible by 6 if it is divisible by 2 and 3 both. Consider the following numbers which are divisible by 6, using the test of divisibility by 6: 42




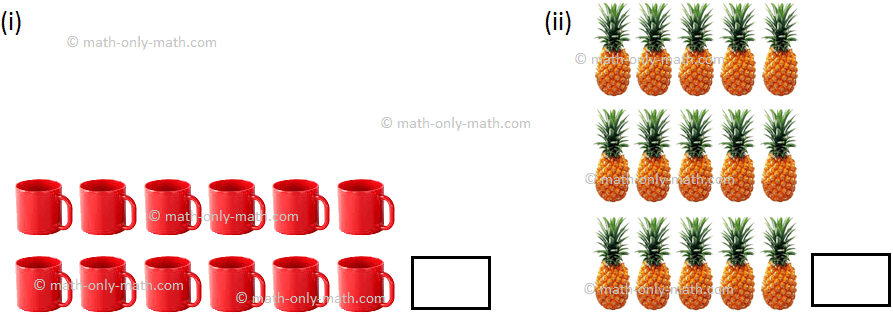



New! Comments
Have your say about what you just read! Leave me a comment in the box below. Ask a Question or Answer a Question.