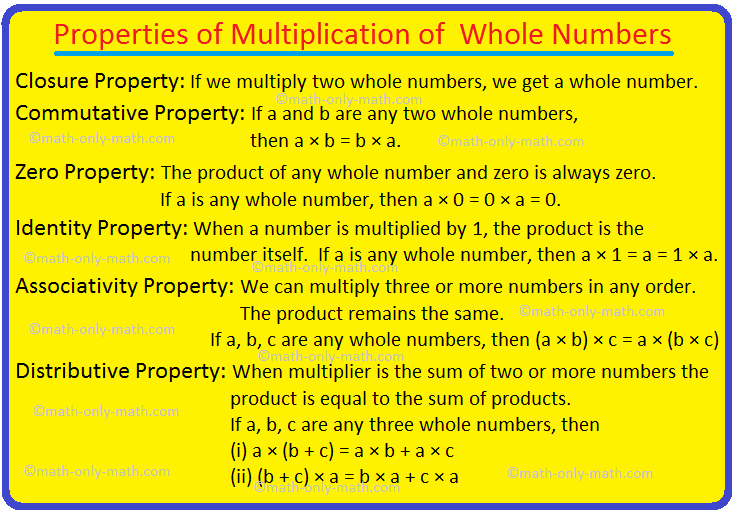
Properties of Multiplication

There are six properties of multiplication of whole numbers that will help to solve the problems easily.

The six properties of multiplication are Closure Property, Commutative Property, Zero Property,  Identity Property, Associativity Property and Distributive Property.

The properties of multiplication on whole numbers are discussed below; these properties will help us in finding the product of even very large numbers conveniently.

I: Closure Property of  Whole Numbers:

If a and b are two numbers, then their product a × b is also a whole number. 

In other words, if we multiply two whole numbers, we get a whole number.

Verification:

In order to verify this property, let us take a few pairs of whole numbers and multiply them;

For example:

(i) 8 × 9 = 72

(ii) 0 × 16 = 0

(iii) 11 × 15 = 165

(iv) 20 × 1 = 20

We find that the product is always a whole numbers.

II: Commutativity of  Whole Numbers / Order Property of  Whole Numbers:

The multiplication of whole numbers is commutative.

In other words, if a and b are any two whole numbers, then a × b = b × a.

We can multiply numbers in any order. The product does not change when the order of numbers is changed.

When multiplying any two numbers, the product remains same regardless of the order of multiplicands. We can multiply numbers in any order, the product remains the same.

For Example:

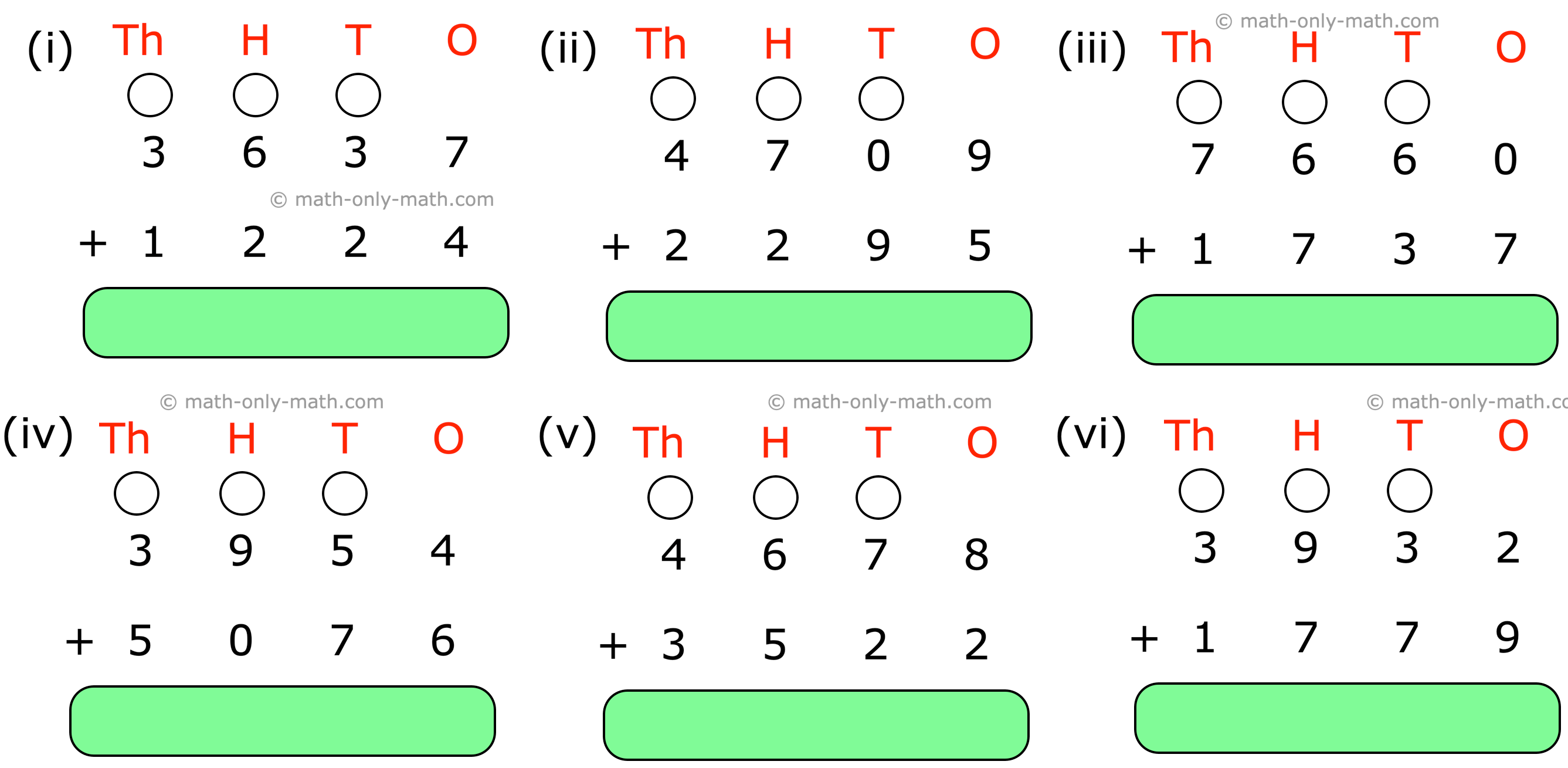
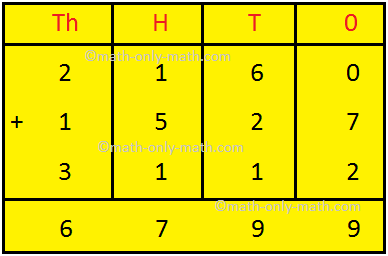
(i) 7 × 4 = 28

    4 × 7 = 28

Verification:

In order to verify this property, let us take a few pairs of whole numbers and multiply these numbers in different orders as shown below;

For Example:

(i) 7 × 6 = 42 and 6 × 7 = 42

Therefore, 7 × 6 = 6 × 7

(ii) 20 × 10 = 200 and 10 × 20 = 200

Therefore, 20 × 10 = 10 × 20

(iii) 15 × 12 = 180 and 12 × 15 = 180

Therefore, 15 × 12 = 12 × 15

(iv) 12 × 13 = 156 and 13 × 12

Therefore, 12 × 13 = 13 × 12

(V) 1122 × 324 = 324 × 1122

(vi) 21892 × 1582 = 1582 × 21892

We find that in whatever order we multiply two whole numbers, the product remains the same.

III: Multiplication By Zero/Zero Property of Multiplication of Whole Numbers:

When a number is multiplied by 0, the product is always 0.

If a is any whole number, then a × 0 = 0 × a = 0.

In other words, the product of any whole number and zero is always zero.

When 0 is multiplied by any number the product is always zero.

For example:

(i) 3 × 0 = 0 + 0 + 0 = 0

(ii) 9 × 0 = 0 + 0 + 0 = 0

Verification:

In order to verify this property, we take some whole numbers and multiply them by zero as shown below;

For example:

(i) 20 × 0 = 0 × 20 = 0

(ii) 1 × 0 = 0 × 1 = 0

(iii) 115 × 0 = 0 × 115 = 0

(iv) 0 × 0 = 0 × 0 = 0

(v) 136 × 0 = 0 × 136 = 0

(vi) 78160 × 0 = 0 × 78160 = 0

(vii) 51999 × 0 = 0 × 51999 = 0

We observe that the product of any whole number and zero is zero.



IV: Multiplicative Identity of Whole Numbers / Identity Property of  Whole Numbers:

When a number is multiplied by 1, the product is the number itself.

If a is any whole number, then a × 1 = a = 1 × a.

In other words, the product of any whole number and 1 is the number itself.

When 1 is multiplied by any number the product is always the number itself.

For example:

(i) 1 × 2 = 1 + 1 = 2

(ii) 1 × 6 = 1 + 1 + 1 + 1 + 1 + 1 = 6

Verification:

In order to verify this property, we find the product of different whole numbers with 1 as shown below:

For example:

(i) 13 × 1 = 13 = 1 × 13

(ii) 1 × 1 = 1 = 1 × 1

(iii) 25 × 1 = 25 = 1 × 25

(iv) 117 × 1 = 117 = 1 × 117

(v) 4295620 × 1 = 4295620

(vi) 108519 × 1 = 108519

We see that in each case a × 1 = a = 1 × a.

The number 1 is called the multiplication identity or the identity element for multiplication of whole numbers because it does not change the identity (value) of the numbers during the operation of multiplication.

V. Associativity Property of Multiplication of Whole Numbers:

We can multiply three or more numbers in any order. The product remains the same.

If a, b, c are any whole numbers, then 

(a × b) × c = a × (b × c)

In other words, the multiplication of whole numbers is associative, that is, the product of three whole numbers does not change by changing their arrangements.

When three or more numbers are multiplied, the product remains the same regardless of their group or place. We can multiply three or more numbers in any order, the product remains the same.

For example:

(i) (6 × 5) × 3 = 90

(ii) 6 × (5 × 3) = 90

(iii) (6 × 3) × 5 = 90

Verification:

In order to verify this property, we take three whole numbers say a, b, c and find the values of the expression (a × b) × c and a × (b × c) as shown below :

For example:

(i) (2 × 3) × 5 = 6 × 5 = 30 and 2 × (3 × 5) = 2 × 15 = 30

Therefore, (2 × 3) × 5 = 2 × (3 × 5)

(ii) (1 × 5) × 2 = 5 × 2 = 10 and 1 × (5 × 2) = 1 × 10 = 10

Therefore, (1 × 5) × 2 = 1 × (5 × 2)

(iii) (2 × 11) × 3 = 22 × 3 = 66 and 2 × (11 × 3) = 2 × 33 = 66

Therefore, (2 × 11) × 3 = 2 × (11 × 3).

(iv) (4 × 1) × 3 = 4 × 3 = 12 and 4 × (1 × 3) = 4 × 3 = 12

Therefore, (4 × 1) × 3 = 4 × (1 × 3).

(v) (1462 × 1250) × 421 = 1462 × (1250 × 421) = (1462 × 421) × 1250

(vi) (7902 × 810) × 1725 = 7902 × (810 × 1725) = (7902 × 1725) × 810

We find that in each case (a × b) × c = a × (b × c).

Thus, the multiplication of whole numbers is associative.

VI. Distributive Property of Multiplication of Whole Numbers / Distributivity of Multiplication over Addition of Whole Numbers:

When multiplier is the sum of two or more numbers the product is equal to the sum of products.

If a, b, c are any three whole numbers, then

(i) a × (b + c) = a × b + a × c

(ii) (b + c) × a = b × a + c × a

In other words, the multiplication of whole numbers distributes over their addition.

Verification:

In order to verify this property, we take any three whole numbers a, b, c and find the values of the expressions a × (b + c) and a × b + a × c as shown below :

For example:

(i) 3 × (2 + 5) = 3 × 7 = 21 and 3 × 2 + 3 × 5 = 6 + 15 =21

Therefore, 3 × (2 + 5) = 3 × 2 + 3 × 5

(ii) 1 × (5 + 9) = 1 × 14 = 15 and 1 × 5 + 1 × 9 = 5 + 9 = 14

Therefore, 1 × (5 + 9) = 1 × 5 + 1 × 9.

(iii) 2 × (7 + 15) = 2 × 22 = 44 and 2 × 7 + 2 × 15 = 14 + 30 = 44.

Therefore, 2 × (7 + 15) = 2 × 7 + 2 × 15.

(vi) 50 × (325 + 175) = 50 × 3250 + 50 × 175

(v) 1007 × (310 + 798) = 1007 × 310 + 1007 × 798

These are the important properties of multiplication of whole numbers.

Let us first review the properties of multiplication learnt earlier:

1. The product of two numbers does not change when we change the order of the numbers.

Example: 25 × 13 = 13 × 25

2. The product of three numbers does not change when we change the groupings of the numbers.

Example: 20 × (8 × 4) = (20 × 8) × 4

                                   = (20 × 4) × 8

3. The product of a number by 1 is the number itself.

Example: 143 × 1 = 143 and 2535 × 1 = 2535

4. The product of a number by zero is always zero

Example: 563 × 0 = 0 and 6984 × 0 = 0

5. The product of a number by the sum of two numbers is the same as the sum of the products of that number by the two numbers separately

Example: 15 × (45 + 38) = 15 × 45 + 5 × 38

REMEMBER: In a multiplication question, the number to be multiplied is called multiplicand, the number try which we multiply is called multiplier and the result of multiplication is called product.

For example,

                  15          ×          10          =          150

           Multiplicand            Multiplier               Product

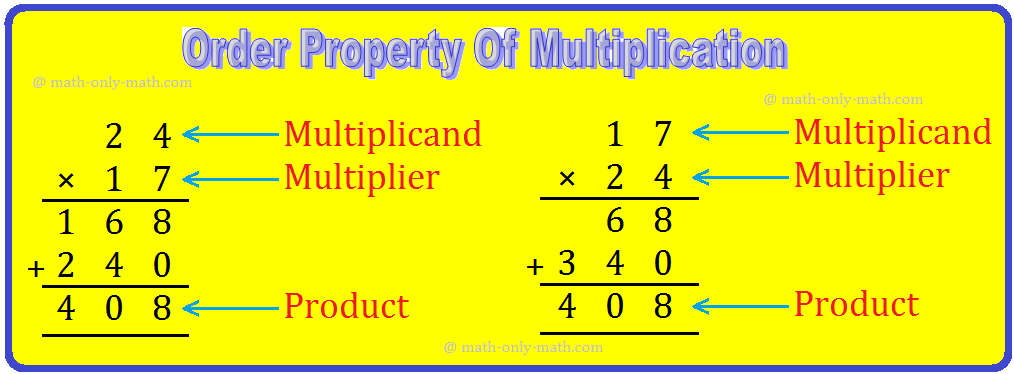
A. Order Property of Multiplication:

A change in the order of the factors does not change the product.

7 × 2 = 7 + 7          = 14	two groups of 7 leaves
2 × 7 = 2 + 2 + 2 + 2 + 2 + 2 + 2          = 14	seven groups of 2 leaves

So, 2 × 7 = 7 × 2 = 14

Observe the following:

What do we serve?

We see that the product in both the cases is the same.

Hence, the product does not change when the order of numbers as changed This property is known as order property of multiplication.

B. Multiplicative Property of 1:

When we multiply a number by 1, the product is the number itself.

(i) 1 + 1 + 1 + 1 = 4 So, 1 × 4 = 4
(ii) 1 + 1 + 1 + 1 + 1 + 1 + 1 = 7 So, 1 × 7 = 7

Observe the following:

70 × 1 = 7

98 × 1 = 98

204 × 1 = 204

What do we observe?

We see that the product of a number and 1 is he number itself This property is known as multiplicative property of 1.

C. Multiplicative Property of 0:

When we multiply any number by 0, the product is always 0.

(i) 0 + 0 = 0

So, 2 × 0 = 0

(ii) 0 + 0 + 0 + 0 + 0 = 0

So, 5 × 0 = 0

Observe the following:

5 × 0 = 0

25 × 0 = 0

292 × 0 = 0

What do we observe?

We see that the product of any number and is 0 This property is known as multiplicative property of 0.

Remember:

The product of two given numbers is always greater than the numbers unless one of the numbers is 1 or 0.

D. Multiplying by 10 and 100:

When we multiply a number by 10, we just add a '0' at the end of the number to get the product.

For example,

6 × 10 = 60

41 ×10 = 410

90 × 10 = 900

When we multiply any number by 100, we just add two '0s' at the end of the number to get the product.

For example,

7 × 100 = 700

8 × 100 = 800

9 × 100 = 900

Worksheet on Properties of Multiplication:

1. Fill in the Blanks.

(i) Number × 0 = __________

(ii) 54 × __________ = 54000

(iii) Number × __________ = Number itself

(iv) 8 × (5 × 7) = (8 × 5) × __________

(v) 7 × _________ = 9 × 7

(vi) 5 × 6 × 12 = 12 × __________

(vii) 62 × 10 = __________

(viii) 6 × 32 × 100 = 6 × 100 × __________

Answers:

(i) 0

(ii) 1000

(iii) 1

(iv) 7

(v) 79

(vi) 5 × 6

(vii) 620

(viii) 32

2. Fill in the blanks using Properties of Multiplication:

(i) 62 × ………… = 5 × 62

(ii) 31 × ………… = 0

(iii) ………… × 9 = 332 × 9

(iv) 134 × 1 = …………

(v) 26 × 16 × 78 = 26 × ………… × 16

(vi) 43 × 34 = 34 × …………

(vii) 540 × 0 = …………

(viii) 29 × 4 × ………… = 4 × 15 × 29

(ix) 47 × ………… = 47

Answer:

2. (i) 5

(ii) 0

(iii) 332

(iv) 134

(v) 78

(vi) 43

(vii) 0

(viii) 15

(ix) 1

3. Fill in the blanks using properties of multiplication:

(i) 629 × 9 = 9 × _____ 

(ii) 999 × _____ = 38 × 999

(iii) 99,125 × _____ = 99,125

(iv) 4,875 × 69 = 69 × _____

(v) _____ × 242 = 242

(vi) 24,210 × 0 = _____

(vii) 12,982 × _____ = 0

(viii) 43,104 × _____ = 43,104

(ix)  _____ × 48,452 = 0

(x) 35 × 43,194 = _____ × 35

(xi) 4,589 ×_____ = 4,589

(xii) 57,130 × _____ = 1,576 × 57,130

(xiii) 700 × 516 = 516 × _____

(xiv) 2,942 x _____ = 0

Answer:

3. (i) 629

(ii) 38

(iii) 1

(iv) 4,875

(v) 1

(vi) 0

(vii) 0

(viii) 1

(ix)  0

(x) 43,194

(xi) 1

(xii) 1,576

(xiii) 700

(xiv) 0

Order in Multiplication:

4. Multiply and fill in the blanks as shown.

(i) 6 × 5 =   30  and 5 × 6 =  30 .  So, 6 × 5 =  5  ×  6 

(ii) 9 × 7 = ___ and 7 × 9 = ___.  So, 9 × 7 = __ × __

(iii) 2 × 10 = ___ and 10 × 2 = ___.  So, 2 × 10 = __ × __

(iv) 3 × 8 = ___ and 8 × 3 = ___.  So, 3 × 8 = __ × __

(v) 4 × 7 = ___ and 7 × 4 = ___.  So, 4 × 7 = __ × __

Answer:

4. (ii) 63; 63; 7; 9

(iii) 20; 20; 10; 2 

(iv) 24; 24; 8; 3

(v) 28; 28; 7; 4

Multiplying by 0 and 1:

5. Write the missing number as shown:

(i) _1_ × 9 = 9

(ii) ___ × 1 = 46

(iii) ___ × 99 = 99

(iv) 41 × ___ = 0

(v) 19 × ___ = 19

(vi) 341 × ___ = 0

(vii) 6 × 0 = ___

(viii) 1 × 8 = ___

(ix) 456 × 0 = ___

Answer:

5. (ii) 46

(iii) 1

(iv) 0

(v) 1

(vi) 0

(vii) 0

(viii) 8

(ix) 0

Multiply by 10 and 100:

6. Multiply the following numbers as shown.

(i) 7 × 10 = _____

(ii) 40 × 10 = _____

(iii) 4 × 100 = _____

(iv) 1 × 100 = _____

(v) 10 × 10 = _____

(vi) 8 × 10 = _____

(vii) 61 × 10 = _____

(viii) 5 × 100 = _____

(ix) 3 × 100 = _____

(x) 2 × 100 = _____

(xi) 9 × 10 = _____

(xii) 72 × 10 = _____

(xiii) 7 × 100 = _____

(xiv) 6 × 100 = _____

(xv) 6 × 10 = _____

Answer:

6. (i) 70

(ii) 400

(iii) 400

(iv) 100

(v) 100

(vi) 80

(vii) 610

(viii) 500

(ix) 300

(x) 200

(xi) 90

(xii) 720

(xiii) 700

(xiv) 600

(xv) 60

● Whole Numbers

• The Number Zero
• Properties of Whole Numbers
• Successor and Predecessor
• Representation of Whole Numbers on Number Line
• Properties of Addition
• Properties of Subtraction
• Properties of Multiplication
• Properties of Division
• Division as The Inverse of Multiplication

Numbers Page 

6th Grade Page 

From Properties of Multiplication to HOME PAGE