Properties of Whole Numbers

The properties of whole numbers are as follows:

• The number 0 is the first and the smallest whole numbers.

• All natural numbers along with zero are called whole numbers. 

• There is no last or greatest whole number.

• There is no largest whole number since they are infinite.

• There is infinitely many or uncountable number of whole numbers.

• All natural numbers are whole numbers.

• Each number is 1 more than its previous number. 

• All whole numbers are not natural numbers.

For example: 0 is a whole number but it is not a natural number.

• Whole numbers are denoted by 'W' normally.

Note:

The system has infinite numbers. 

Thus, W = {0, 1, 2, 3, 4, ……….}

Even Whole Numbers (E): 

A system of whole numbers, which are divisible by 2 or are multiples of 2, is called a set of even numbers. It is denoted by 'E'. 

Thus, E = {2, 4, 6, 8, 10, 12, .....} 

There are infinite even numbers. 

Odd Whole Numbers (O): 

A system of whole numbers, which are not divisible by 2 or are not multiples of 2, is called a set of odd numbers. It is denoted by 'O'.

Thus, O = {1, 3, 5, 7, 9, 11, .....} 

There are infinite odd numbers. 

Note:

‘0’ (Zero) is neither a negative number nor a positive number, it’s a natural number.

Having learnt about four basic operations are addition, subtraction, multiplication and division on whole numbers. We shall now study the properties of these operations on whole numbers. Set of whole numbers: w = {0, 1, 2, 3 ,............}

I: Properties of Addition of Whole Numbers:

A: Closure Property of Addition of Whole Numbers:

The sum of any two whole numbers is a whole number.
Hence, whole numbers are closed under addition. If x and y are any two whole numbers, then x + y is also a whole number.

For Example:

(i) 3 + 8 = 11 (a whole number)

(ii) 25 + 42 = 67 (a whole number)

B: Commutative Property of Addition of Whole Numbers:

Two whole numbers can be added in any order.

Hence, addition is commutative for whole numbers.

If a and b are any whole numbers, then

x + y = y + x

For Example:

(i) 0 + 7 = 7 and 7 + 0 = 7

(ii) 85 + 73 = 158 and 73 + 85 = 158

REMEMBER

In whatever order two whole numbers are added, their sum always remains the same.

C: Additive Property Zero of Whole Numbers:

The sum of a whole number and 0 is the whole number itself. Zero is called the identity element under addition or additive identity for whole numbers.

If x is any whole number, then

x + 0 = 0 + x = x

For Example:

(i) 752 + 0 = 0 + 752 = 752

(ii) 2565 + 0 = 0 + 2565 = 2565

D: Associative Property Zero of Whole Numbers:

Three or more whole numbers can be grouped in any order to find their sum.

Hence, addition is associative for whole numbers.

If x, y and z are any three whole numbers, then

(x + y) + z = x + (y + z)

For Example:

(i) (645 + 707) + 883 = 1,352 + 883 = 2,235

and 645 + (707 + 883) = 645 + 1590 = 2,235

Therefore, (645 + 707) + 883 = 645 + (707 + 883)

(ii) (888 + 2,330) + 755 = 3218 + 755 = 3,973

and 888 + (2,330 + 755) = 888 + 3,085 = 3,973

Therefore, (888 + 2,330) + 755 = 888 + (2,330 + 755)

By using the commutative and associative properties of addition of whole numbers, often it becomes convenient to add two or more whole numbers as shown in the following examples.

Solved Examples Properties of Whole Numbers

1. Find the sum by suitable rearrangement.

(i) 943 + 508 + 557

(ii) 2920 + 532 + 2580 + 468

Solution:

(i) 943 + 508 + 557

(943 + 557) + 508

1500 + 508 = 2008

(ii) 2920 + 532 + 2580 + 468

(2920 + 2580) + (532 + 468)

= 5500 + 1000

= 6500

2. Find the sum of the following.

(i) 12873 + 9999

(ii) 99999 + 289326

Solution:

(i) 12873 + 9999

= 12873 + (10000 - 1)

= (12873 + 10000) - 1

= 22873 - 1

= 22872

(ii) 99999 + 289326

= (100000 - 1) + 289326

= (289326 + 100000) - 1

= 389326 - 1

= 389325

II: Properties of Subtraction of Whole Numbers:

A: Closure Property of Subtraction of Whole Numbers:

The difference of two whole numbers is not always a whole number. Subtraction results in a whole number only when the number from which the other is subtracted is greater than or equal to the other number.

Hence, whole numbers are not closed under subtraction.

If x and y are two whole numbers such that x > y or x = y, then x - y is whole number but if x < y, then subtraction x - y is not possible in whole numbers.

For Example:

(i) Subtraction of two equal whole numbers results in whole number 0.

For Example:

           8 - 8 = 0,

       57 - 57 = 0,

      549 - 549 = 0, etc.

(ii) Subtraction of a smaller whole number from a larger whole number results in a whole number,

For Example:

            48 - 15 = 33,

           249 - 64 = 185,

        100000 - 1 = 99,999, etc.

(iii) Subtraction of a larger whole number from a smaller one does not result in a whole number.

For Example:

            45 - 84 is not equal to a whole number.

            235 - 460 is not equal to a whole number.

B: Commutative Property of Subtraction of Whole Numbers:

Two whole numbers cannot be subtracted in any order. Hence, subtraction is not commutative for whole numbers. If x and y are any two whole numbers, then

x - y ≠ y - x

For Example:

(i) 42 - 12 = 30, a whole number but 12 - 42 is not defined in whole numbers.

(ii) 850 - 170 = 680, a whole number but 170 - 850 is not defined in whole numbers.

C: Property of Zero of Subtraction of Whole Numbers:

If zero is subtracted from any whole number, the result is the number itself. Thus, the identity property of a zero holds good when 0 is subtracted from a whole number. When a whole number other than 0 is subtracted from 0, the result is not a whole number.

If x is any whole number, then x - 0 = x but 0 - x is not defined in whole numbers.

For Example:

(i) 39 - 0 = 39, a whole number but 0 - 39 is not defined in whole numbers.

(ii) 559 - 0 = 559, a whole number but 0 - 559 is not defined in whole numbers.

C: Associative Property of Subtraction of Whole Numbers:

Subtraction is not associative for whole numbers. If x, y, z are any three whole numbers, then

(x - y)- z ≠ x - (y - z)

For Example:

                            (8 - 4) - 2 = 4 - 2 = 2

                      and 8 - (4 - 2) = 8 - 2 = 6

            Therefore, (8 - 4) - 2 ≠ 8 - (4 - 2)

D: Inverse operations of Subtraction of Whole Numbers:

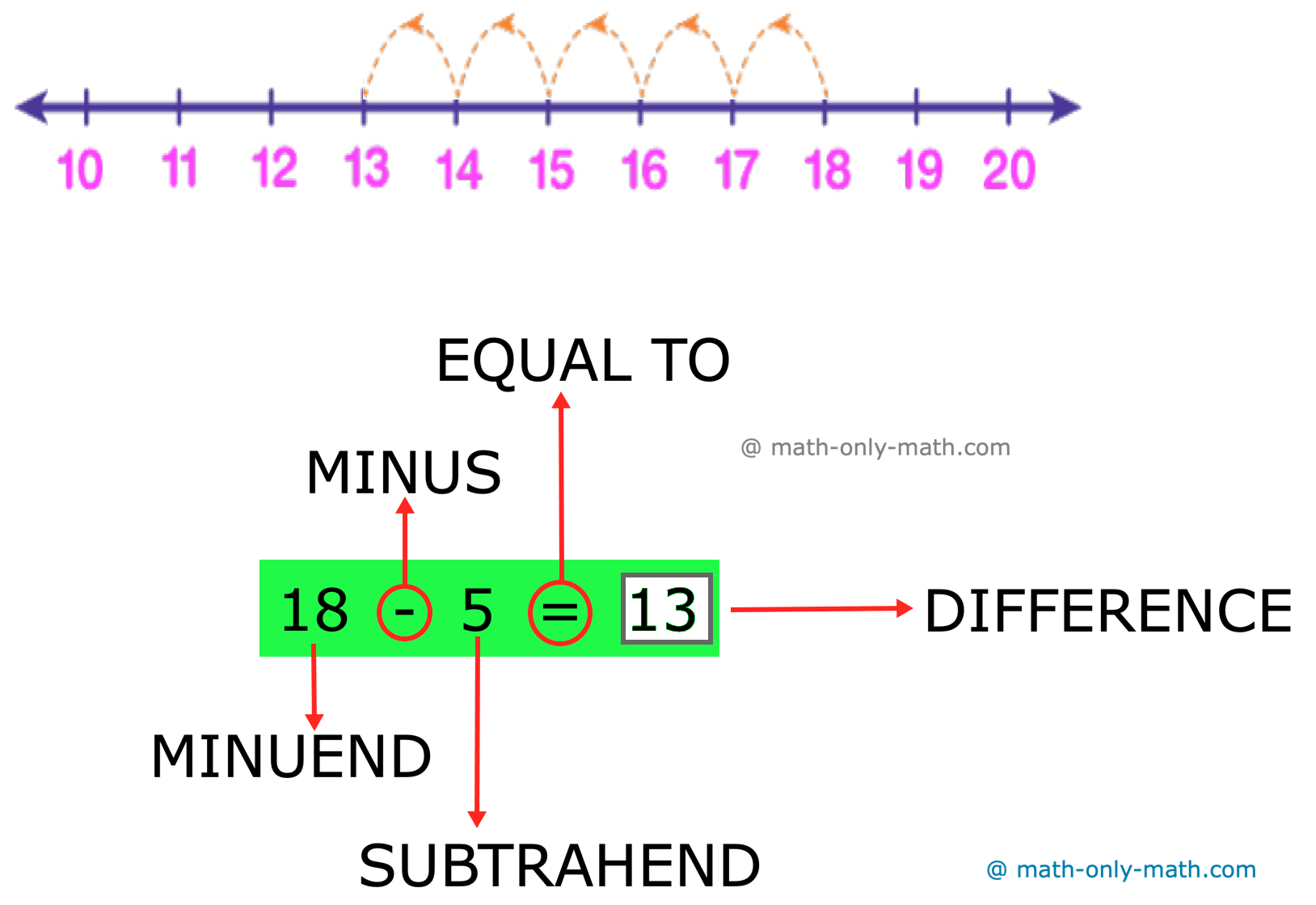
The operations of addition and subtraction are inverse of each other. Thus, subtracting a whole number, say, 18 from the whole number 60 can be considered as finding a whole number which when added to the whole number 18 results in 60.

60 - 18 = 42 ⟹ 18 + 42 = 60

If x, y, z are any three whole numbers such that

x - y = z, then y + z = x.

For Example:

(i) 30 - 17 = 13 ⟹ 17 + 13 = 30

(ii) 23 - 8 = 15 ⟹ 8 + 15 = 23

II: Properties of Multiplication of Whole Numbers:

A: Closure Property of Multiplication of Whole Numbers:

The product of two whole numbers is always a whole number.

Hence, whole numbers are closed under multiplication.

If m and n are any two whole numbers, then their product m × n is also a whole number.

For Example:

(i) 8 × 6 = 54 a whole number

(ii) 42 × 4 = 168 a whole number

B: Commutative Property of Multiplication of Whole Numbers:

Two whole numbers can be multiplied in any order. Hence, multiplication is commutative for whole numbers.

If y and z are any two whole numbers, then

y × z = z × y

For Example:

(i) 8 × 4 = 32 and 4 × 8 = 32

Therefore, 8 × 4 = 4 × 8

(ii) 15 × 20 = 300 and 20 × 15 = 300

Therefore, 15 × 20 = 20 × 15

C: Multiplicative Property of Zero of Whole Numbers:

The product of any whole number and zero is always zero.

If z is any whole number, then z × 0 = 0 × z = 0

For Example:

(i) 6 × 0 = 0 × 6 = 0

(ii) 365 × 0 = 0 × 365 = 0

D: Existence of Multiplicative Identity Property of Whole Numbers:

The product of any whole number and 1 is the number itself.

1 is called the multiplicative identity or identity element for whole numbers under multiplication.

If z is any whole number, then

z × 1 = 1 × z = z

For Example:

(i) 72 × 1 = 1 × 72 = 72

(ii) 245 × 1 = 1 × 245 = 245

E: Associative Property Multiplication of Whole Numbers:

Three or more whole numbers can be grouped in any order to find their product. Hence, multiplication is associative for whole numbers.

If a, b, c are any three whole numbers, then

(a × b) × c = a × (b × c)

For Example:

                     (3 × 5) × 4 = 15 × 4 = 60

               and 3 × (5 × 4) = 3 × 20 = 60

     Therefore, (3 × 5) × 4 = 3 × (5 × 4)

F: Distributive Property Multiplication Over Addition of Whole Numbers:

The multiplication of whole numbers distributes over their addition.

If a, b, c are any three whole numbers, then

a × (b + c) = a × b + a × c

For Example:

                    3 × (5 + 7) = 3 x (12) = 36

              and 3 × 5 + 3 × 7 = 15 + 21 = 36

                 Therefore, 3 × (5 + 7) = 3 × 5 + 3 × 7

F: Distributive Property Multiplication Over Subtraction of Whole Numbers:

The multiplication of whole numbers distributes over their subtraction.

If a, b, c are whole numbers such that b > c, then

a × (b - c) = a × b - a × c

For Example:

                18 × (8 - 5) = 18 × 3 = 54

    and 18 × 8 - 18 × 5 = 144 - 90 = 54

Therefore, 18 × (8 - 5) = 18 × 8 - 18 × 5

Solved Examples on Properties of Multiplication of Whole Numbers:

1. Determine each of the following products by suitable rearrangement.

(i) 4 × 6798 × 250

(ii) 2388 × 25 × 2 × 40

Solution:

(i) 4 × 6798 × 250

= 6798 × (4 × 250)

= 6798 x 1000

= 6798000

(ii) 2388 × 25 × 2 × 40

= (2388 × 2) × (25 × 40)

= 4776 × 10000 

= 4776000

2. Find the value of each of the following using various properties.

(i) 638 × 7 + 638 × 3

(ii) 997 × 10 × 982 - 882 × 9970

Solution:

(i) 638 × 7 + 638 × 3

= 638 × (7 + 3)

= 638 × 10

= 6380

(ii) 997 × 10 × 982 - 882 × 9970

= (997 × 10 × 982) - (9970 × 882)

= (9970 × 982) - (9970 × 882)

= 9970 × (982 - 882)

= 9970 × 100

= 997000

3. Using distributive property of multiplication over addition/subtraction in whole numbers, find the product of each of the following.

(i) 498 × 102

(ii) 736 ×1003

(iii) 482 × 64

Solution:

(i) 498 × 102

= 498 × (100 + 2)

= 498 × 100 + 498 × 2

= 49800 + 996

= 50796

(ii) 736 × 1003

= 736 × (1000 + 3)

= 736 × 1000 + 736 × 3

= 736000 + 2208

= 738208

(iii) 482 × 64

= (400 + 80 + 2) × 64

= 400 × 64 + 80 × 64 + 2 × 64

= 25600 + 5120 + 128

= 30848

4. Find the product of the greatest number of five digits and the greatest number of four digits.

Solution:

Greatest five digit number = 99999

Greatest four digit number = 9999

Now find the product of greatest 5-digit and greatest 4-digit numbers

Product = 99999 × 9999

            = 99999 × (10000 - 1)

            = 99999 x 10000 - 99999 × 1 [Using distributive property]

            = 999990000 - 99999

            = 999990000 - (100000 - 1)

            = 999990000 - 100000 + 1 

            = 999890000 + 1

            = 999890001

5. 29 laptops and 29 tables were purchased for a new office. If each laptop costs $2,632 and each table costs $368, find the total amount spend.

Solution:

Cost of 29 laptops = $2,632 × 29

Cost of 29 chairs = $368 × 29

Total cost = $(2,632 × 29 + 368 × 29)

               = $ 29 (2,632 + 368); [Using Distributive Property]

               = $ 29 (3,000)

               = $ (30 - 1) (3,000)

               = $ (30 × 3,000 - 1 × 3,000); [Using Distributive Property]

               = $ (90,000 - 3,000)

               = $ 87,000

Hence, the total amount spend = $ 87,000

II: Properties of Division of Whole Numbers:

A: Closure Property of Division of Whole Numbers:

The quotient of two whole numbers when one is divided by the other is not always a whole number.

Hence, whole numbers are not closed under division. If x and y (y ≠ 0) are whole numbers, then x ÷ y not always a whole number.

For Example:

(i) 18 ÷ 3 = 6, a whole number

(ii) 23 ÷ 7, is not a whole number

B: Commutative Property of Division of Whole Numbers:

Division is not commutative for (non-zero) whole numbers.

If x and y are whole numbers, then

x ÷ y ≠ y ÷ x (x ≠ y, x ≠ 0, y ≠ 0)

For Example:

24 ÷ 6 = 4, a whole number and 6 ÷ 24 = $\frac{6}{24}$ = 1/4 not a whole number.

Clearly, 24 ÷ 6 ≠ 6 ÷ 24

C: Associative Property of Division of Whole Numbers:

Division is not associative for whole numbers.

If x, y, z are whole numbers, then

(x ÷ y) ÷ z ≠ x ÷ (y ÷ z); (y ≠ 0, z ≠ 0)

For Example:

                (30 ÷ 5) ÷ 6 = 6 ÷ 6 = 1

          and 30 ÷ (5 ÷ 6) = 30 ÷ $\frac{5}{6}$ = 30 × $\frac{6}{5}$ = 36

Therefore, (30 ÷ 5) ÷ 6 ≠ 30 ÷ (5 ÷ 6)

D: Property of Division by 1 of Whole Numbers:

Any whole number divided by 1 gives the quotient as the number itself.

If x is any whole number, then x ÷ 1 = x.

For Example:

(i) Since,    1 × 5 =  5;      Therefore, 5 ÷ 1   =  5

(ii) Since, 85 × 1 = 85;      Therefore, 85 ÷ 1 = 85

(iii) Since,  0 × 1 =  0;       Therefore, 0 ÷ 1   =  0

(iv) Since,  1 × 1 =  1;       Therefore, 1 ÷ 1   =  1

E: Property of Division by a Number itself of Whole Numbers:

Any whole number (other than zero) divided by itself gives 1 as the quotient.

If x is a whole number (other than zero), then x ÷ x = 1.

For Example:

(i) Since,    6 =   6 × 1;        Therefore,     6 ÷ 6  =  1

(ii) Since,  43 = 43 × 1;        Therefore,  43 ÷ 43 = 1

(iii) Since, 85 = 85 × 1;        Therefore, 85 ÷ 85  =  85

(iv) Since,  1 =   1 × 1;        Therefore,    1 ÷ 1   =  1

F: Property of Zero Divided by a Whole Numbers:

Zero divided by any whole number (other than 0) gives the quotient as 0.

If x is a whole number other than 0, then 0 ÷ x = 0.

For Example:

(i) Since,       0 × 8 = 0;       Therefore, 0 ÷ 8    = 0

(ii) Since,      0 × 5 = 0;       Therefore,  0 ÷ 5   = 0

(iii) Since,    0 × 49 = 0;      Therefore, 0 ÷ 49  = 0

(iv) Since,  0 × 845 = 0;      Therefore, 0 ÷ 845 = 0

G: Property of Divided by Zero of a Whole Numbers:

Division by zero is not defined. To evaluate 4 ÷ 0, we need to find a whole number which when multiplied by zero results in 4. No such number can be obtained as the product of any whole number and zero is always zero.

Hence, division of a whole number by zero is meaningless.

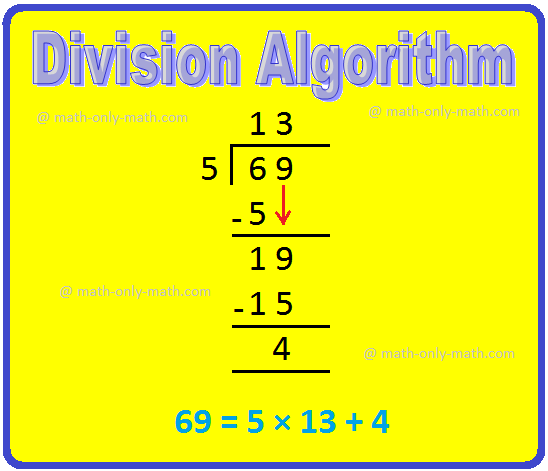
H. Division Algorithm of a Whole Numbers:

Dividend = Divisor × Quotient + Remainder,

where dividend is the number to be divided, divisor is the number by which the dividend is divided, quotient is the number which is left over after division.

In general, if a and b are whole numbers such that a > b and b ≠ 0 and on dividing a by b the quotient and the remainder obtained are q and r respectively, then a = bq + r, where 0 ≤ r < b.

For Example:

Divide 69 by 5 and verify the division algorithm.

Clearly, 69 = 5 × 13 + 4

I. Even and Odd Whole Numbers:

Even whole numbers are the whole numbers which are divisible by 2.

For example, 0, 2, 4, 6, 8, 10, 12, etc. are all even numbers.

Odd whole numbers are the whole numbers which are not divisible by 2.

For example, 1, 3, 5, 7, 9, 11, 13, etc. are all odd numbers.

I. Worksheet on Properties of Addition of Whole Numbers:

1. Fill in the blanks.

(i) If two whole numbers are added, the sum is always a _____ number.

(ii) Whole numbers are _____ under addition.

(iii) _____ is called the identity element for whole numbers under addition.

(iv) In whatever order two whole numbers are added, their sum always remains _____.

(v) If a is a whole number such that a + a = a, then a = _____. 

Answer:

1. (i) whole number

(ii) Identity element

(iii) zero

(iv) the same

(v) 0

2. Fill in the blanks to make each of the following a true statement.

(i) 6728 + 0 = __________

(ii) __________ + 125831 = 125831

(iii) 5239 + 928 = 928 +

(iv) 9325 + __________ = 998 + 9325

(ν) 337 + (892 + 617) = (337 + 892) + __________

Answer:

2. (i) 6728

(ii) 0

(iii) 5239

(iv) 998 

(ν) 617

3. Find the sum by using a short method.

(i) 312875 + 9999

(ii) 1289345 + 99999

Answer:

3. (i) 312875 + 9999

= 312875 + (10000 - 1)

= (312875 + 10000) - 1

= 322875 - 1

= 322874

(ii) 1289345 + 99999

= 1289345 + (100000 - 1)

= (1289345 + 100000) -1

= 1389345 -1

= 1389344

4. Determine each of the following sums using suitable rearrangement.

(i) 647 + 142 + 858 + 253

(ii) 211 + 684 + 389 + 5816

(iii) 15209 + 378 + 791 + 122

(iv) 496 + 497 + 498 + 499 + 1 + 2 + 3 + 4

Answer:

4. (i) 647 + 142 + 858 + 253

 = (647 + 858) + (253 + 142)

= 1505 + 395

= 1900

(ii) 211 + 684 + 389 + 5816

= (211 + 389) + (684 + 5816)

= 600 - 6500

= 7100

(iii) 15209 + 378 + 791 + 122

= (15209 + 791) + (378 + 122)

= 16000 + 500

= 16500

(iv) 496 + 497 + 498 + 499 + 1 + 2 + 3 + 4

= (496 + 4) + (497 + 3) + (497 + 2) + (499 + 1)

= 500 + 500 + 500 + 500

= 2000

II. Worksheet on Properties of Subtraction of Whole Numbers:

1. For each of the following addition sentence write two subtraction sentences.

(i) 23 + 12 = 35

(ii) 326 + 422 = 748

(iii) 135 + 92 = 227

Answer:

1. (i) 23 + 12 = 35 

⟹ 23 = 35 - 12 

⟹ 12 = 35 - 23

(ii) 326 + 422 = 748

⟹ 326 = 748 - 422

⟹ 422 = 748 - 326

(iii) 135 + 92 = 227

⟹ 135 = 227 - 92

⟹ 92 = 227 - 135

2. Perform the following subtractions and check your results by corresponding additions.

(i) 9,328 - 427

(ii) 41,000 - 10,999

(iii) 100,000 - 75,652

(iv) 6,050,501 - 787,879

Answer:

2. (i) 9328 - 427 = 8901

Check: 8901 + 427 = 9328

(ii) 41000 - 10999 = 30,001

Check: 30,001+ 10,999=41000

(iii) 100,000 - 75,652 = 24,348

Check: 24,348 + 75,652 = 100,000

(iv) 6,050,501 - 787,879 = 5,262,622

Check: 5,262,622 + 787,879 = 6,050,501

3. Fill in the blanks.

(i) 100,000 - __________ = 8,019

(ii) 759,999 - 83,599 = __________

Answer:

3. (i) 91,981

(ii) 676,400

4. Find the difference between the smallest number of 5 digits and the largest number of 4 digits.

Answer:

4. 1

5. Find the difference between the smallest number of 7 digits and the largest number of 4 digits.

Answer:

5. 990,001

6. Find the whole number x when

(i) x + 7 = 20

(ii) x + 43 = 215

(iii) x - 17 = 41

(iv) x - 30,298 = 32,307

Answer:

6. (i) x = 13

(ii) x = 172

(iii) x = 58

(iv) x = 62,605

7. The population of a town was 150800. In one year it increased by 4290 due to new births. In the increased population if the number of men is 61296, determine the number of women.

Answer:

7. 93,794

III. Worksheet on Properties of Multiplication of Whole Numbers:

1. Fill in the blanks.

(i) 992 × 0 = __________

(ii) 6675 × 1 = __________

(ii) 2198 × 557 = 557 × __________

(iv) 88 × (125 × 45) = (88 × 125) × __________

(v) 90 × 100 × __________ = 900000

(vi) 125 × (86 + 95) = 125 × 86 + __________ × 95

Answer:

1. (i) 0

(ii) 6675

(ii) 2198

(iv) 45

(v) 100

(vi) 125

2. Determine each of the following products by suitable rearrangements.

(i) 25 × 928 × 4

(ii) 50 × 6793 × 2

(iii) 25 × 8 × 5432

(iv) 874 × 625 × 16

(v) 40 × 1345 × 25 × 2

(vi) 20 × 625 × 8 × 50

Answer:

2. (i) 25 × 928 × 4

= 928 (25 × 4)

= 928 × 100

= 92800

(ii) 50 × 6793 × 2

= 6793 (50 × 2)

= 6793 × 100

= 679300

(iii) 25 × 8 × 5432

= (25 × 8) × 5437

= 200 × 5432

= 1,086,400

(iv) 874 × 625 × 16

= 874(625 × 16)

= 874 × 10000

= 8,740,000

(v) 40 × 1345 × 25 × 2

= (1345 × 2)(40 × 25)

= 2690 × 1000

= 2,690,000

(vi) 20 × 625 × 8 × 50

= (625 × 8)(50 × 20)

= 5000 * 1000

= 5,000,000

3. Find the value of each of the following using various properties.

(i) 199 × 29 + 199 × 71

(ii) 1679 × 999 + 1679

(iii) 389 × 17 + 389 × 23 + 389 × 60

(iv) 683 × 36 + 683 × 17 - (683 × 48) - (5 × 683)

(v) 2398 × 761 - 2398 × 661

(vi) 12345 × 12345 - 12345 × 2345

Answer:

3. (i) 199 × 29 + 199 × 71

= 199 (29 + 71)

= 199 × 100

= 19,900

(ii) 1679 × 999 + 1679

= 1679 × (999 + 1)

= 1679 × 1000

= 1,679,000

(iii) 389 × 17 + 389 × 23 + 389 × 60

= 389 (17 + 23 + 60)

= 389 × 100

= 38,900

(iv) 683 × 36 + 683 × 17 - (683 × 48) - (5 × 683)

= 683 (36 + 17) - 683 × 48 - 5 × 683

= 683 (53) - 683 (48 + 5)

= 683 (53) - 683 (53)

= 683(53 - 53)

= 683 × 0

= 0

(v) 2398 × 761 - 2398 × 661

= 2,398 (761 - 661)

= 2,398 × 100

= 239,800

(vi) 12345 × 12345 - 12345 × 12345

= 12345 (12345 - 12345)

= 12345 (0)

= 0

4. Use the distributive property of multiplication over addition, subtraction to find the following.

(i) 932 × 103

(ii) 345 × 1008

(iii) 3847 × 97

(iv) 350 × 64

Answer:

4. (i) 932 × 103

= 932 (100 + 3)

= 932 × 100 + 932 × 3

= 93200 + 2796

= 95,996

(ii) 345 × 1,008

= 345 (1,000 + 8)

= 345 × 1,000 + 345 × 8 

= 345,000 + 2,760

= 347,760

(iii) 3847 × 97

= 3847 (100 - 3)

= 3847 × 100 - 3847 × 3

= 384,700 - 11,541

= 373,159

(iv) 350 × 64

= (300 + 50) × 64

= 300 × 64 + 50 × 64

= 19,200 + 3,200

= 22,400

Worksheet on Properties of Division of Whole Numbers:

1. Find the value of the following

(i) 923287 ÷ 1

(ii) 0 ÷ 6993

(iii) 990 ÷ (640 ÷ 64)

(iv) 7896 ÷ (2347 ÷ 2347)

(v) 999 + (2975 ÷ 2975)

(vi) (6208 ÷ 6208) - (2358 ÷ 2358)

Answer:

1. (i) 923287

(ii) 0

(iii) 99

(iv) 7896

(v) 1000

(vi) 0

2. Divide and check the result by division algorithm in each of the following.

(i) 2873 ÷ 35

(ii) 93875 ÷ 651

(iii) 121878 ÷ 88

(iv) 254254 ÷ 675

Answer:

2. (i) 2873 = 35 × 82 + 3

(ii) 93875 = 651 × 128 + 447

(iii) 121878 = 88 × 1384 + 86

(iv) 254254 = 675 × 376 + 454

3. Divide and find out the quotient and remainder. Check your answer.

(i) 94335 ÷ 93

(ii) 10000 ÷ 125

(ⅲ) 66087 ÷ 285

(iv) 99999 ÷ 423

Answer:

3. (i) Quotient = 1014; Remainder = 33

        94335 = 93 × 1014 + 33

(ii) Quotient = 80; Remainder = 0

        10000 = 125 × 80 + 0

(ⅲ) Quotient = 231; Remainder = 252

       66087 = 285 × 231 + 252

(iν) Quotient = 263; Remainder = 171

       99999 = 423 × 263 + 171

● Whole Numbers

The Number Zero

Properties of Whole Numbers

Successor and Predecessor

Representation of Whole Numbers on Number Line

Properties of Addition

Properties of Subtraction

Properties of Multiplication

Properties of Division

Division as The Inverse of Multiplication

Numbers Page 

6th Grade Page 

From Properties of Whole Numbers to HOME PAGE