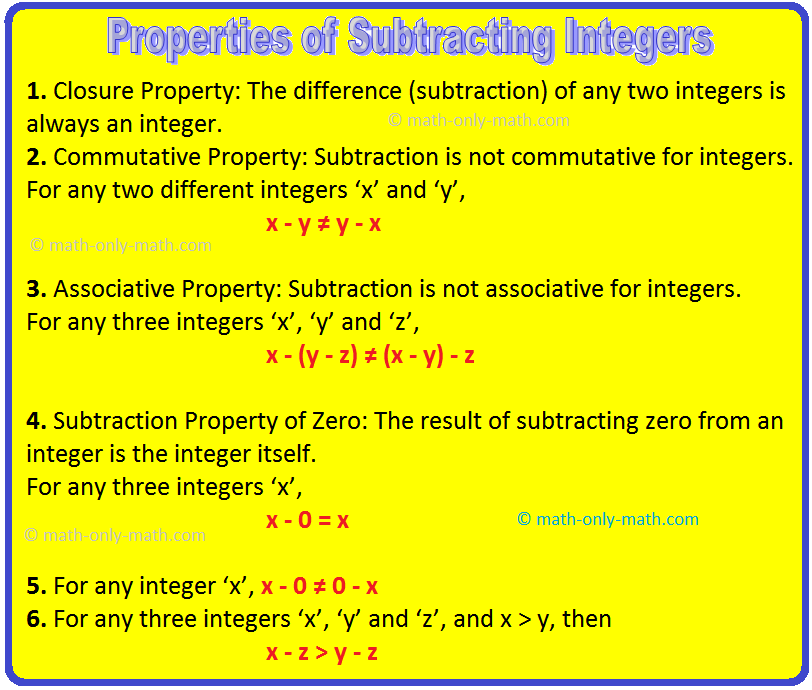
Properties of Subtraction
Some properties of subtraction of whole numbers are:
Property I:
If a and b are two whole numbers such that a > b or a = b, then a – b is a whole number. If a < b, then subtraction a – b is not possible in whole numbers.
For example:
9 - 5 = 4
87 - 36 = 51
130 - 60 = 70
119 - 59 = 60
28 - 0 = 28
Property II:
The subtraction of whole numbers is not commutative, that is, if a and b are two whole numbers, then in general a – b is not equal to (b – a).
Verification:
We know that 9 – 5 = 4 but 5 – 9 is not possible. Also, 125 – 75 = 50 but 75 – 125 is not possible. Thus, for two whole numbers a and b if a > b, then a – b is a whole number but b – a is not possible and if b > a, then b – a is a whole number but a – b is not possible.
Hence, in general (a – b) is not equal to (b – a)
Property III:
If a is any whole number other than zero, then a – 0 = a but 0 – a is not defined.
Verification:
We know that 15 – 0 = 15, but 0 – 15 is not possible.
Similarly, 39 – 0 = 39, but 0 – 39 is not possible.
Again, 42 – 0 = 42, but 0 – 42 is not possible.
Property IV:
The subtraction of whole numbers is not associative. That is, if a, b, c are three whole numbers, then in general a – (b – c) is not equal to (a – b) – c.
Verification:
We have,
20 – (15 – 3) = 20 – 12 = 8,
and, (20 – 15) – 3 = 5 – 3 = 2
Therefore, 20 – (15 – 3) ≠ (20 – 15) – 3.
Similarly, 18 – (7 – 5) = 18 – 2 = 16,
and, (18 – 7) – 5 = 11 – 5 = 6.
Therefore, 18 – (7 – 5) ≠ (18 – 7) – 5.
Property V:
If a, b and c are whole numbers such that a – b = c, then b + c = a.
Verification:
We know that 25 – 8 = 17. Also, 8 + 17 = 25
Therefore, 25 – 8 = 17 or, 8 + 17 = 25
Similarly 89 – 74 = 15 because 74 + 15 = 89.
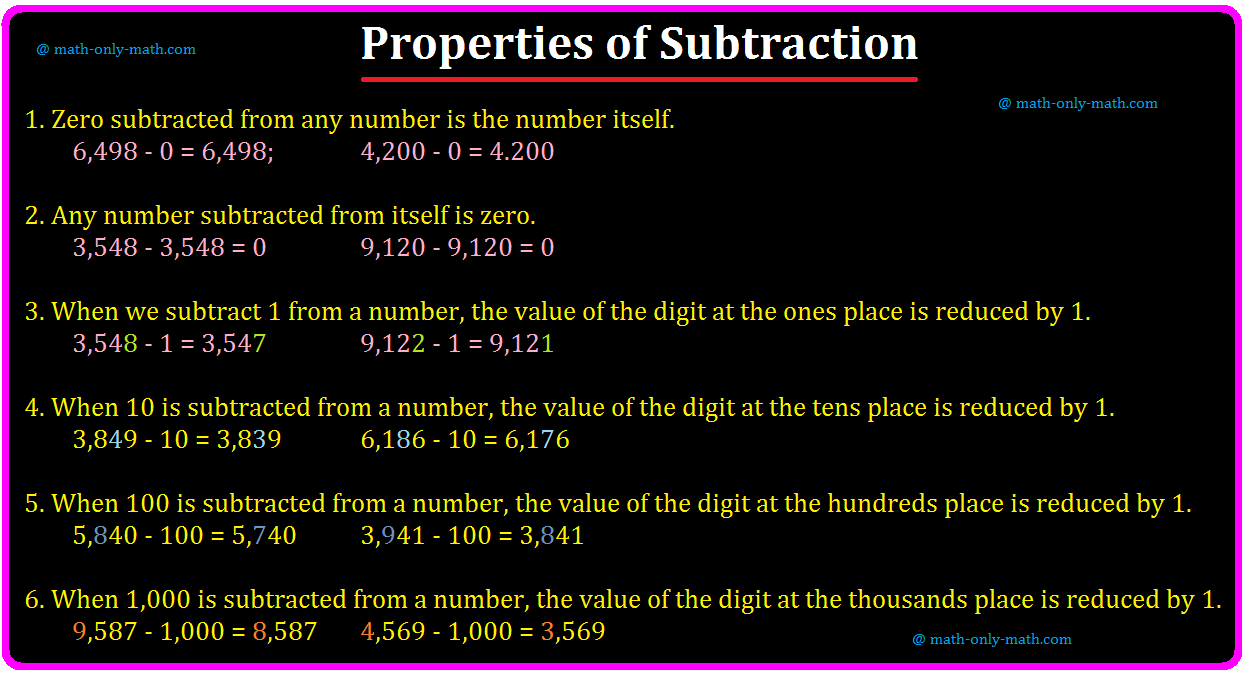
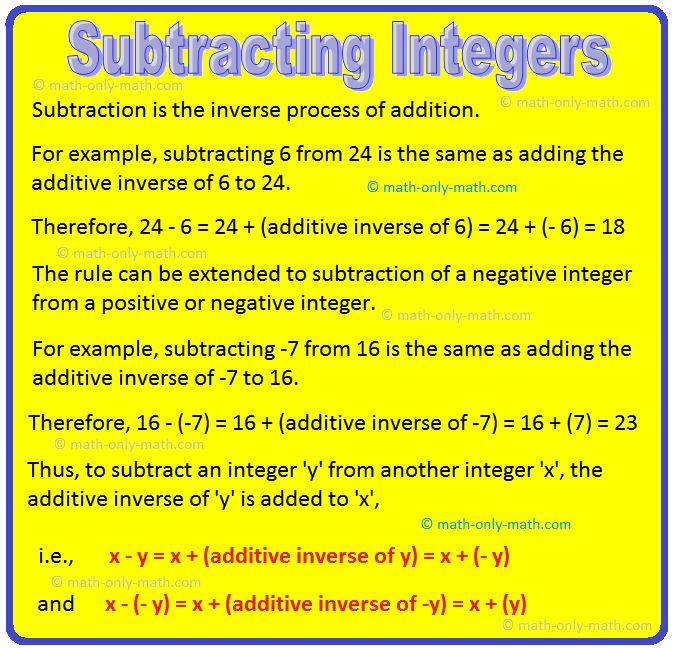
● Zero Property of Subtraction - When zero is subtracted from the number, the difference is the number itself.
For example,
(i) 8931 – 0 = 8931;
(ii) 5649 – 0 = 5649;
(iii) 245 – 0 = 245
(iv) 197 – 0 = 197
● Properties of Subtraction of a Number from itself: When a number is subtracted from itself the difference is zero.
For example,
(i) 5485 – 5485 = 0
(ii) 345 – 345 = 0
(iii) 279 – 279 = 0
● Predecessor – On subtracting 1 from any number, we get the number just before it. When 1 is subtracted from a number, we get its predecessor.
For example,
(i) 6001 – 1 = 6000
(ii) 6000 – 1 = 5999
(iii) 163 – 1 = 162
(iv) 171 – 1 = 170
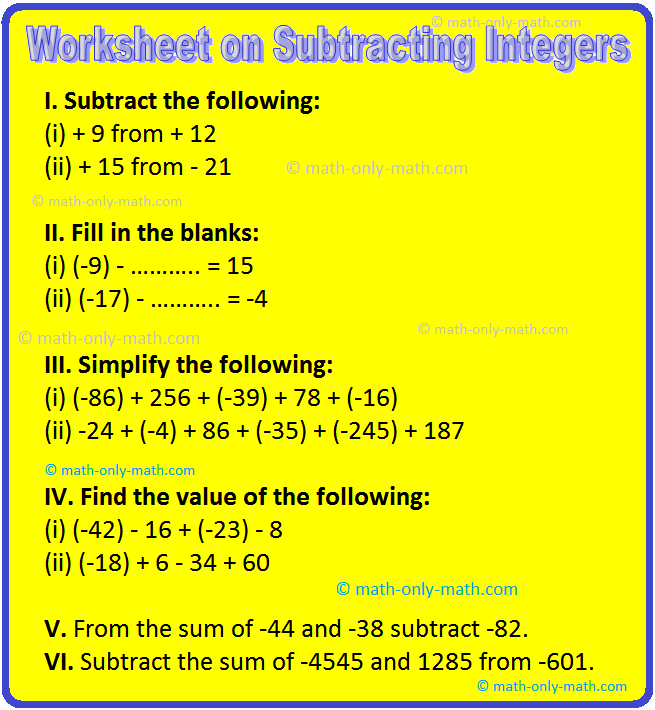
Worksheet on Properties of Subtraction:
I. Fill in the blanks:
(i) 568 – 0 = …………….
(ii) 7530 – 4530 = …………….
(iii) 7790 – 1 = …………….
(iv) 65894 – 65893 = …………….
(v) 54172 - ……………. = 0
(vi) 8688 – 8288 = …………….
(vii) 7721 – 5620 = …………….
(viii) 17281 – 1 = …………….
(ix) ……………. – 1 = 29999
(x) 29080 - ……………. = 29079
(xi) 548 - ………….. = 0
(xii) ………….. – 0 = 274
(xiii) 367 - ………….. = 367
(xiv) 765 – 765 = …………..
(xv) 212 – 0 = …………..
(xvi) 167 - ………….. = 0
(xvii) 647 – 647 = …………..
(xviii) 326 – 326 = …………..
(xix) ………….. – 0 = 876
(xx) 429 – 0 = …………..
(xxi) 999 – 999 = …………..
(xxii) 412 - ………….. = 412
(xxiii) 700 - 100 = …………..
(xxiv) 100 - 10 = …………..
(xxv) 9429 - 100 = …………..
(xxvi) 4583 - 1000 = …………..
(xxvii) 9498 - 1000 = …………..
(xxviii) 1000 - 1000 = …………..
Answers:
(i) 568
(ii) 3000
(iii) 7789
(iv) 1
(v) 54172
(vi) 400
(vii) 2101
(viii) 17280
(ix) 30000
(x) 1
(xi) 54
(xii) 274
(xiii) 0
(xiv) 0
(xv) 212
(xvi) 167
(xvii) 0
(xviii) 0
(xix) 876
(xx) 429
(xxi) 0
(xxii) 0
(xxiii) 600
(xxiv) 90
(xxv) 9329
(xxvi) 3583
(xxvii) 8498
(xxviii) 0
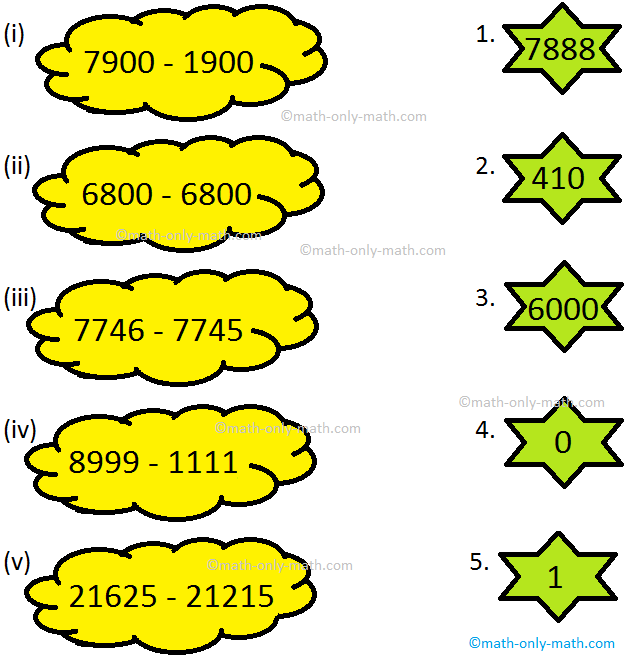
II. Match the given difference to its solution by coloring the cloud and the shape with same color.
Answer:
(i) → 3
(ii) → 4
(iii) → 5
(iv) → 1
(v) → 2
III. Write the predecessor of the following numbers:
(i) 259 …………..
(ii) 608 …………..
(iii) 450 …………..
(iv) 374 …………..
(v) 900 …………..
(vi) 529 …………..
(vii) 201 …………..
(viii) 598 …………..
Answers:
III. (i) 258
(ii) 607
(iii) 449
(iv) 373
(v) 899
(vi) 528
(vii) 200
(viii) 597
IV: Fill in the blanks on the basis of subtraction facts:
(i) 43 - 0 = _____
(ii) 18 - 1 = _____
(iii) 77 - 77 = _____
(iv) 54 - 0 = _____
(v) 33 - 1 = _____
(vi) 62 - 0 = _____
(vii) 98 - 1 = _____
(viii) 56 - 56 = _____
(ix) 34 - 0 = _____
Answer:
IV: (i) 43
(ii) 17
(iii) 0
(iv) 54
(v) 32
(vi) 62
(vii) 97
(viii) 0
(ix) 34
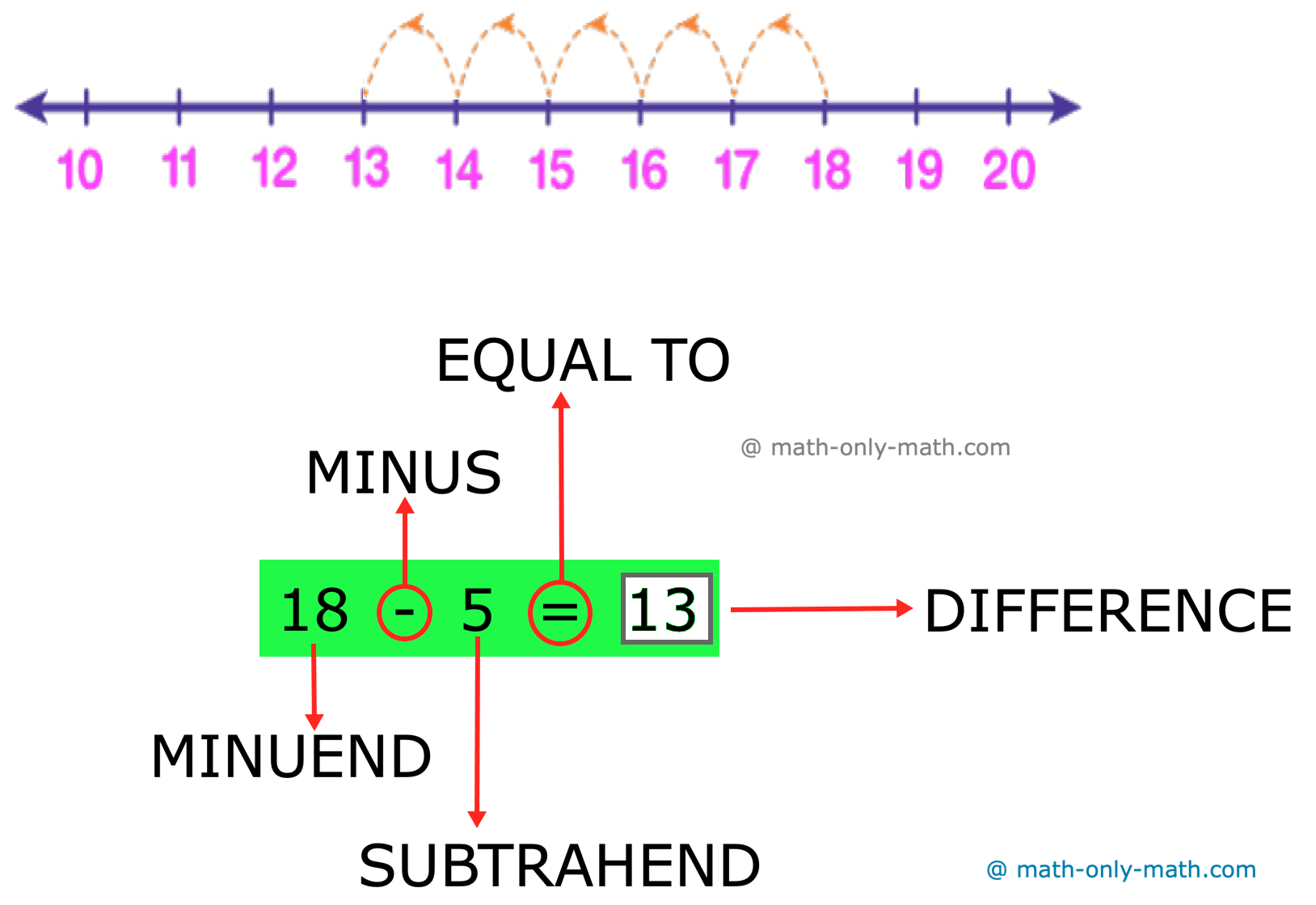
1. When do you find the difference is the number itself?
1. When do you find the difference is the number itself?
Answer: Subtracting zero from a number means no subtraction. The difference is the number itself.
Examples: 11 - 0 = 11
19 - 0 = 19
93 - 0 = 93
2. How do we get the predecessor of a number?
2. How do we get the predecessor of a number?
Answer: By subtracting 1 from a number, we get the previous number or predecessor of that number.
Examples: 18 - 1 = 17
29 - 1 = 28
101 - 1 = 100
3. What the difference between two numbers is zero (0)?
3. What the difference between two numbers is zero (0)?
Answer: When a number is subtracted from itself the difference is zero.
Examples: 10 - 10 = 0
75 - 75 = 0
98 - 98 = 0
Math Only Math is based on the premise that children do not make a distinction between play and work and learn best when learning becomes play and play becomes learning.
However, suggestions for further improvement, from all quarters would be greatly appreciated.
Numbers Page
6th Grade Page
From Properties of Subtraction to HOME PAGE
Didn't find what you were looking for? Or want to know more information about Math Only Math. Use this Google Search to find what you need.
Recent Articles
-
Counting Numbers from 1 to 50 | Match the Number | Missing Numbers
Apr 04, 25 03:46 PM
In counting numbers from 1 to 50, recognize the numbers, count and then join the numbers in the correct number order. Here we mainly need eye-hand coordination to draw the picture and maintain the num -
Counting Eleven to Twenty with Numbers and Words |Numbers from 11 - 20
Apr 04, 25 03:21 PM
Counting eleven to twenty with numbers and words are explained below. One ten and one more is eleven. Eleven comes after ten. One ten and two more is twelve. Twelve comes after eleven. -
5th Grade BODMAS Rule Worksheet | PEMDAS | Order of operations|Answers
Apr 03, 25 03:11 PM
In 5th Grade BODMAS Rule Worksheet you will get different types of problems on mathematical expressions involving different operations, mathematical expression with 'brackets' and 'of' and simplifying… -
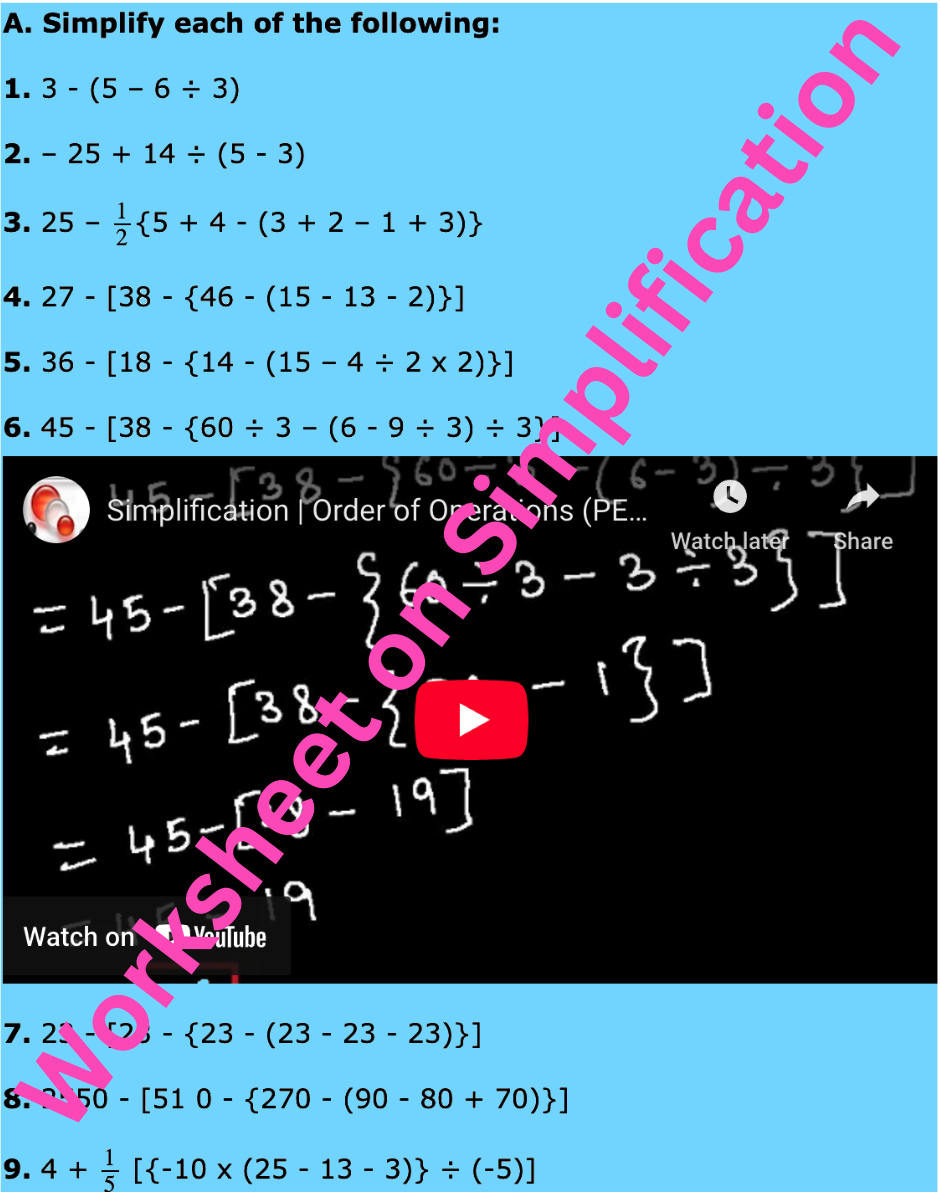
Worksheet on Simplification | Simplify Expressions | BODMAS Questions
Apr 03, 25 02:58 PM
In worksheet on simplification, the questions are based in order to simplify expressions involving more than one bracket by using the steps of removal of brackets. This exercise sheet -
Divisible by 2 Video |Test of Divisibility by 2 Trick| Rules| Examples
Apr 03, 25 10:25 AM
A number is divisible by 2 if the digit at unit place is either 0 or multiple of 2. So a number is divisible by 2 if digit at its units place is 0, 2, 4, 6 or 8.







New! Comments
Have your say about what you just read! Leave me a comment in the box below. Ask a Question or Answer a Question.