Finding the Median of Grouped Data

To find the median of arrayed (grouped) data we need to follow the following steps:

Step I: Arrange the grouped data in ascending or descending order, and form a frequency table.

Step II: Prepare a cumulative-frequency table of the data.

Step III: Select the cumulative frequency that is just greater than $\frac{N}{2}$, where N is the total number of observations (variates). Then find the median as follows.

The variate whose cumulative frequency is the selected cumulative frequency, is the median of the data.

If $\frac{N}{2}$ is equal to the cumulative frequency of a variate then

median = mean of this variate and the variate just greater than it.

Solved Examples on Find the Median of Grouped Data /Arrayed Data:

1. Find the median of the following distribution.

Solution:

Here, the frequency distribution is given.

The cumulative-frequency table of the distribution is

Here, $\frac{N}{2}$ = $\frac{16}{2}$ = 8.

The cumulative frequency just greater than 8 is 10.

The variate whose cumulative frequency is 10 is 6.

Therefore, the median = 6.

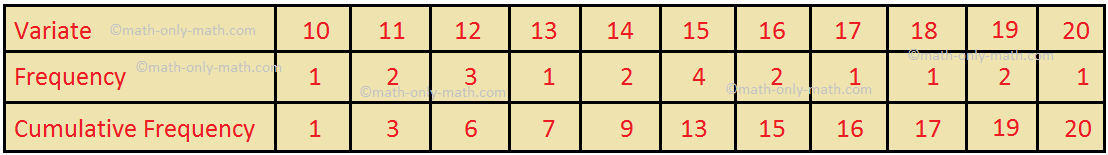
2. Find the median of the arrayed data given below.

10, 11, 11, 12, 12, 12, 13, 14, 14, 15, 15, 15, 15, 16, 16, 17, 18, 19, 19, 20.

Solution:

Putting the data in a frequency table, we have the cumulative frequencies as below.

Here, the total frequency N = 20.

So, $\frac{N}{2}$ = $\frac{20}{2}$ = 10.

The cumulative frequency just greater than 10 is 13 and the corresponding variates is 15. So, the median = 15.

9th Grade Math

From Finding the Median of Grouped Data to HOME PAGE