Dividing a Quantity in Three given Ratios
Rules of dividing a quantity in three given ratios is explained below along with the different types of examples.
If a quantity K is divided into three parts in the ratio X : Y : Z, then
First part = X/(X + Y + Z) × K,
Second part = Y/(X + Y + Z) × K,
Third part = Z/(X + Y + Z) × K.
For example, suppose, we have to divide $ 1200 among X, Y, Z in the ratio 2 : 3 : 7. This means that if X gets 2 portions, then Y will get 3 portions and Z will get 7 portions. Thus, total portions = 2 + 3 + 7 = 12. So, we have to divide $ 1200 into 12 portions and then distribute the portions among X, Y, Z according to their share.
Thus, X will get 2/12 of $ 1200, i.e., 2/12 × 1200 = $ 200
Y will get 3/12 of $ 1200, i.e., 3/12 × 1200 = $ 300
Z will get 7/12 of $ 1200, i.e., 7/12 × 1200 = $ 700
Solved examples:
1. If $ 135 is divided among three boys in the ratio 2 : 3 : 4, find the share of each boy.
Solution:
The sum of the terms of the ratio = 2 + 3 + 4 = 9
Share of first boy = 2/9 × 135 = $ 30.
Share of second boy = 3/9 × 315 = $ 45.
Share of first boy = 4/9 × 315 = $ 60.
Thus, the required shares are $ 30, $ 45 and $ 60 respectively.
2. Divide 99 into three parts in the ratio 2 : 4 : 5.
Solution:
Since, 2 + 4 + 5 = 11.
Therefore, first part = 2/11 × 99 = 18.
Second part = 4/11 × 99 = 36.
And, third part = 5/11 × 99 = 45.
3. 420 articles are divided among A, B and C, such that A gets three-times of B and B gets five-times of C. Find the number of articles received by B.
Solution:
Let the number of articles C gets = 1
The number of article that B gets = five times of C = 5 × 1 = 5.
And, the number of articles that A gets = three times of B = 3 × 5 = 15.
Therefore, A : B : C = 15 : 5 : 1
And, A + B + C = 15 + 5 + 1 = 21
The number of articles received by B = 5/21 × 420 = 100
The above examples on dividing a quantity in three given ratios will help us to solve different types of problems on ratios.
6th Grade Page
From Dividing a Quantity in Three given Ratios to HOME PAGE
Didn't find what you were looking for? Or want to know more information about Math Only Math. Use this Google Search to find what you need.
Recent Articles
-
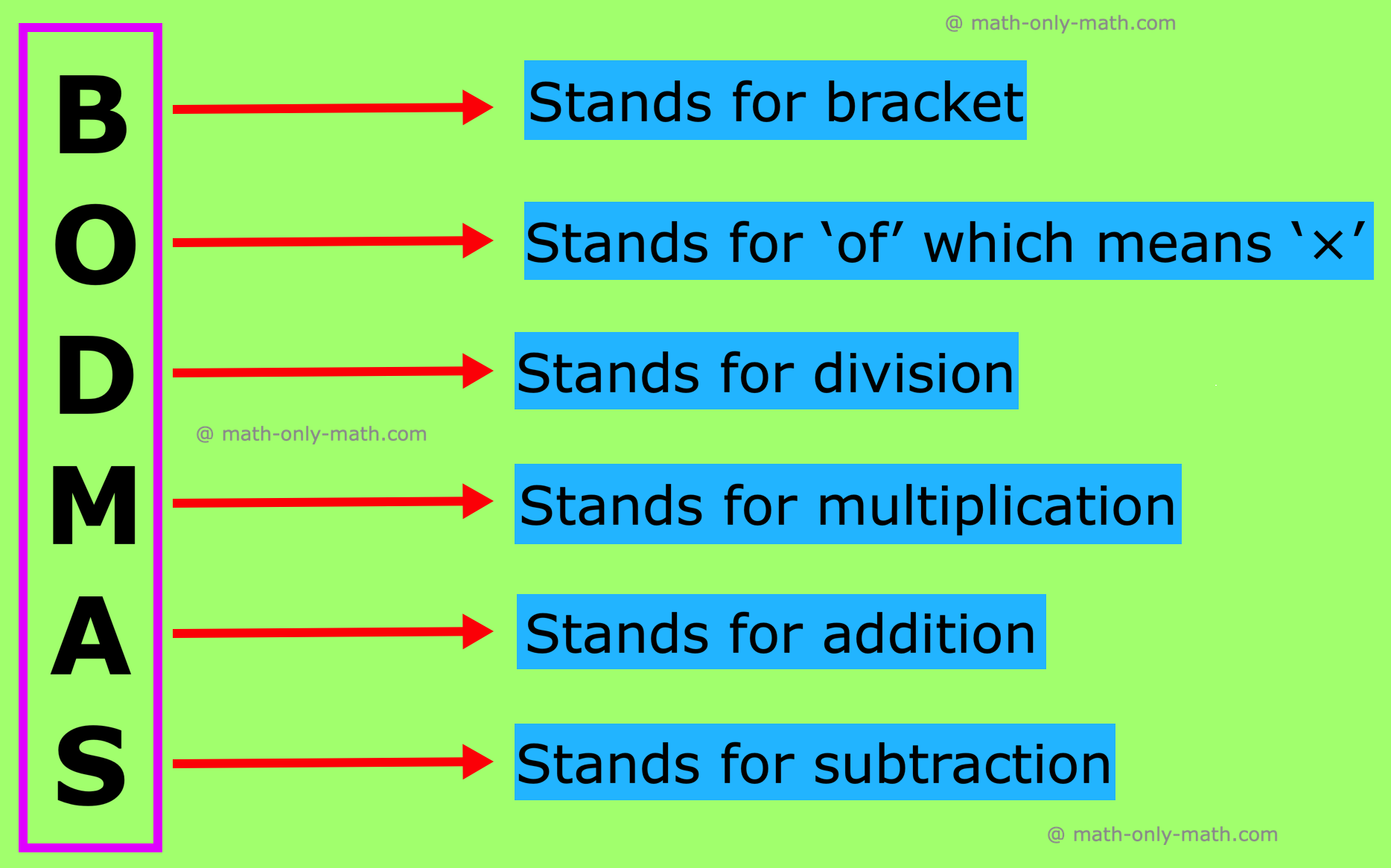
BODMAS Rule | Order of Operation | Definition, Examples, Problems
Mar 30, 25 01:11 PM
Easy and simple way to remember BODMAS rule!! B → Brackets first (parentheses) O → Of (orders i.e. Powers and Square Roots, Cube Roots, etc.) DM → Division and Multiplication -
Subtracting 1-Digit Number | Subtract Two One-Digit Number | Video
Mar 30, 25 10:16 AM
In subtracting 1-digit number we will subtract or minus one-digit number from one-digit number or one-digit number from 2-digit number and find the difference between them. We know that subtraction me… -
Divisible by 10 | Test of Divisibility by 10 Video | Rules | Examples
Mar 29, 25 03:06 PM
Divisible by 10 is discussed below. A number is divisible by 10 if it has zero (0) in its units place. Consider the following numbers which are divisible by 10, using the test of divisibility by 10: -
Divisible by 9 | Test of Divisibility by 9 | Rules | Video | Examples
Mar 29, 25 02:55 PM
A number is divisible by 9, if the sum is a multiple of 9 or if the sum of its digits is divisible by 9. Consider the following numbers which are divisible by 9, using the test of divisibility by 9: -
Divisible by 6 | Rules for Test of Divisibility by 6 Video | Examples
Mar 29, 25 02:48 PM
Divisible by 6 is discussed below: A number is divisible by 6 if it is divisible by 2 and 3 both. Consider the following numbers which are divisible by 6, using the test of divisibility by 6: 42




New! Comments
Have your say about what you just read! Leave me a comment in the box below. Ask a Question or Answer a Question.