Conversion of Numbers

Conversion of numbers from one system to another becomes necessary to understand the process and the logic of the operations of a computer system. It is not very difficult to convert numbers from one base to another. We will first discuss about the conversion of binary numbers to their decimal equivalents.

(i) Expansion Method:

In expansion method the conversion of binary numbers to their decimal equivalents are shown with the help of the examples.

1. Convert the decimal numbers to their binary equivalents:

(a) 256

Solution:

256

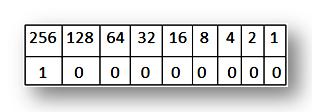
Since the given number 256 appears in the first row, we put 1 in the slot below 256 and fill all the other slots to the right of this slot with zeros.

Thus, 256₁₀ = 100000000₂

(b) 77

Solution:

77

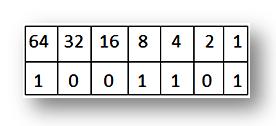
The given number is less than 128 but greater than 64. We therefore put 1 in the slot corresponding to 64 in the first row. Next, we subtract 64 from 77 and get 13 as remainder.

This remainder is less than 16 and greater than 8. So we put 1 in the slot corresponding to 8 and subtract 8 from 13. This gives 13 - 8 = 5. This remainder is greater than 4 and less than 8.

Hence we put 1 in the slot corresponding to 4 and subtracting 4 from 5 we get 1. Now, 1 is present in the right hand most slot of the first row. We, therefore, put 1 in the corresponding slot and fill all other slots with zeros.

Thus, 77₁₀ = 1001101₂.

Conversion of decimal fractions to binary fractions may also be accomplished by using similar method. Let us observe the procedure with the help of the following example:

2. Convert 0.675₁₀ to its binary equivalent.

Solution:

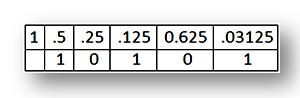
Subtract .5 from the given number to get .675 - .5 = .175 and place 1 in the slot corresponding to .5 of the first row.

Now the number .175 is less than .25 and greater than .125. So, we put 1 in the slot corresponding to the number .125 of the first row and subtract .125 from .175 to get .175 - .125 = .05. The remainder .05 is less than .0625 but greater than .03125.

Hence we put 1 in the slot corresponding to 0.3125 and the subtraction given .05 - .03125 = .01875 and continue the process. The other slots are then filled with zeros.

Thus, .675₁₀ = (.10101…)₂

Note:

It should be noted that the conversion of decimal fractions to binary fractions may not be exact and the process is to be continued until there is no remainder or the remainder is less than the order of accuracy desired.

(ii) Multiplication and Division Method:

We explain conversion of numbers using multiplication and division method with the help of following example.

1. Convert 4215₁₀ to its binary equivalent

Solution:
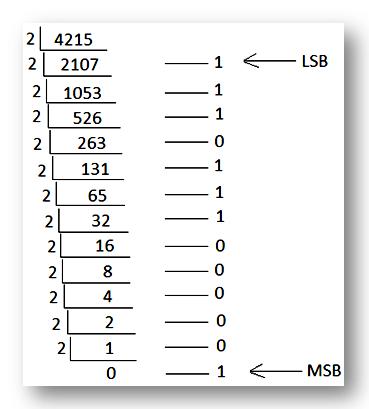
Therefore, 4215₁₀ =1000001110111₂

The conversion of decimal fractions to binary fractions is accomplished by multiplying repeatedly the decimal fraction by the base 2 of the binary number. The integral part after each multiplication is either 0 or 1. The equivalent binary fraction is obtained by writing the integral parts of each product to the right of the binary point in the same sequence. If the fractional part of the product becomes exactly zero at a certain stage, then the binary fraction is finite, otherwise, the fraction is non-terminating and then we find the binary fraction upto the desired degree of accuracy. We explain the process with the help of the following examples.

2. Convert the following decimal numbers to their binary equivalents:

(a) 0.375

Solution:

Decimal Numbers to Binary Number Conversion Table
Multiplication	Integer	Fraction
0.375 × 2 = 0.75	0	.75
0.75 × 2 = 1.5	1	.5
.5 × 2 = 1.0	1	0

Therefore, 0.375₁₀ = 0.011₂


(b) 0.435

Solution:

Decimal Numbers to Binary Number Conversion Table
Multiplication	Integer	Fraction
0.435 × 2 = 0.87	0	.87
0.87 × 2 = 1.74	1	.74
.74 × 2 = 1.48	1	.48
.48 × 2 = 0.96	0	.96
.96 × 2 = 1.92	1	.92

Therefore, 0.435₁₀ = (0.01101…)₂

Fox mixed number, we will have to separate the number into its integral and fractional parts and find the binary equivalent of each part independently.

Finally, we add the two parts to get the binary equivalent of the given number.

3. Convert (56.75)₁₀ to its binary equivalent.

Solution:

At first we find the binary equivalent of 56.
Therefore, 56₁₀ = 111000₂

The binary equivalent of 0.75 is obtained below:

Decimal Numbers to Binary Number Conversion Table
Multiplication	Integer	Fraction
0.75 × 2 = 1.5	1	.5
0.5 × 2 = 1.0	1	0

Therefore, 0.75₁₀ = 0.11₁₀

Hence 56.75₁₀ = 111000.11₁₀

● Binary Numbers

• Data and Information

• Number System

• Decimal Number System

• Binary Number System

• Why Binary Numbers are Used

• Binary to Decimal Conversion

• Conversion of Numbers

• Octal Number System

• Hexa-decimal Number System

• Conversion of Binary Numbers to Octal or Hexa-decimal Numbers

• Octal and Hexa-Decimal Numbers

• Signed-magnitude Representation

• Radix Complement

• Diminished Radix Complement

• Arithmetic Operations of Binary Numbers

• Binary Addition

• Binary Subtraction

• Subtraction by 2’s Complement

• Subtraction by 1’s Complement

• Addition and Subtraction of Binary Numbers

• Binary Addition using 1’s Complement

• Binary Addition using 2’s Complement

• Binary Multiplication

• Binary Division

• Addition and Subtraction of Octal Numbers

• Multiplication of Octal Numbers

• Hexadecimal Addition and Subtraction

From Conversion of Numbers to HOME PAGE