Trigonometrical Ratios of (- θ)

What is the relation among all the trigonometrical ratios of (– θ)?

In trigonometrical ratios of angles (- θ) we will find the relation between all six trigonometrical ratios.

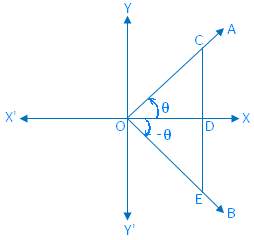
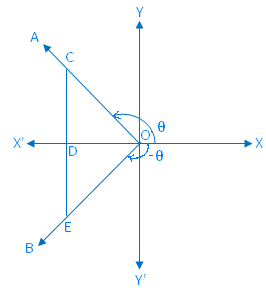
Let a rotating line OA rotates about O in the anti-clockwise direction. From initial position to ending position OA make an angle ∠XOA = θ.

Again a rotating line OA rotates about O in the clockwise direction and makes an angle ∠XOB having magnitude equal to ∠XOA.

Then we get, ∠XOB = - θ. Observe the diagram 1 and 4 to take a point C on OA and draw CD perpendicular to OX. Or we can also observe the diagram 2 and 3 where CD perpendicular to OX'. Let produce CD to intersect OB at E. Now, from the ∆ COD and ∆ EOD we get ∠COD = ∠EOD (same magnitude), ∠ODC = ∠ODE and OD is common.

Therefore, ∆ COD ≅ ∆ EOD (congruent)

Therefore,  according to the rules of trigonometric sign we get,

ED = - CD and OE = OC.

Again according to the definition of trigonometric ratios,

sin (- θ) = \(\frac{ED}{OE}\)

sin (- θ) = \(\frac{- CD}{OC}\), [ED = CD and OE = OC since, ∆ COD ≅ ∆ EOD]

sin (- θ) = - sin θ

again, cos (- θ) = \(\frac{OD}{OE}\)

cos (- θ) = \(\frac{OD}{OC}\), [OE = OC since, ∆ COD ≅ ∆ EOD]

cos (- θ) = cos θ

again, tan (- θ) = \(\frac{ED}{OD}\)

tan (- θ) = \(\frac{- CD}{OD}\), [ED = CD since, ∆ COD ≅ ∆ EOD]

tan (- θ) = -  tan θ.

similarly, csc (- θ) = \(\frac{1}{sin (- \Theta)}\)

csc (- θ) = \(\frac{1}{-  sin \Theta}\)

csc (- θ) = - csc θ.

again, sec (- θ) = \(\frac{1}{cos (- \Theta)}\)

sec (- θ) = \(\frac{1}{cos \Theta}\) 

sec (- θ) = sec θ.

And again, cot (- θ) = \(\frac{1}{tan (- \Theta)}\)

cot (- θ) = \(\frac{1}{-  tan \Theta}\)

cot (- θ) = - cot θ.

Solved example:

1. Find the value of sin (- 45)°.

Solution:

sin (- 45)° = - sin 45°; since we know sin (- θ) = - sin θ

               = \(\frac{-1}{√2}\)

2. Find the value of sec (- 60)°.

Solution:

sec (- 60)° = sec 60°; since we know sec (- θ) = sec θ

                = 2   

3. Find the value of cot (- 90)°.

Solution:

cot (- 90)° = - tan 90°; since we know cot (- θ) = - tan θ

                = 0

● Trigonometric Functions

• Basic Trigonometric Ratios and Their Names
• Restrictions of Trigonometrical Ratios
• Reciprocal Relations of Trigonometric Ratios
• Quotient Relations of Trigonometric Ratios
  
• Limit of Trigonometric Ratios
  
• Trigonometrical Identity
• Problems on Trigonometric Identities
• Elimination of Trigonometric Ratios
• Eliminate Theta between the equations
• Problems on Eliminate Theta
• Trig Ratio Problems
• Proving Trigonometric Ratios
• Trig Ratios Proving Problems
• Verify Trigonometric Identities
• Trigonometrical Ratios of 0°
• Trigonometrical Ratios of 30°
• Trigonometrical Ratios of 45°
• Trigonometrical Ratios of 60°
• Trigonometrical Ratios of 90°
• Trigonometrical Ratios Table
• Problems on Trigonometric Ratio of Standard Angle
  
• Trigonometrical Ratios of Complementary Angles
• Rules of Trigonometric Signs
• Signs of Trigonometrical Ratios
  
• All Sin Tan Cos Rule
• Trigonometrical Ratios of (- θ)
• Trigonometrical Ratios of (90° + θ)
• Trigonometrical Ratios of (90° - θ)
• Trigonometrical Ratios of (180° + θ)
• Trigonometrical Ratios of (180° - θ)
• Trigonometrical Ratios of (270° + θ)
• Trigonometrical Ratios of (270° - θ)
• Trigonometrical Ratios of (360° + θ)
• Trigonometrical Ratios of (360° - θ)
• Trigonometrical Ratios of any Angle
• Trigonometrical Ratios of some Particular Angles
• Trigonometric Ratios of an Angle
• Trigonometric Functions of any Angles
• Problems on Trigonometric Ratios of an Angle
• Problems on Signs of Trigonometrical Ratios

11 and 12 Grade Math

From Trigonometrical Ratios of (- θ) to HOME PAGE