The Cube Roots of Unity
We will discuss here about the cube roots of unity and their properties.
Suppose let us assume that the cube root of 1 is z i.e., ∛1 = z.
Then, cubing both sides we get, z\(^{3}\) = 1
or, z\(^{3}\) - 1 = 0
or, (z - 1)(z\(^{2}\) + z + 1) = 0
Therefore, either z - 1 = 0 i.e., z = 1 or, z\(^{2}\) + z + 1 = 0
Therefore, z = \(\frac{-1\pm \sqrt{1^{2} - 4\cdot 1\cdot 1}}{2\cdot 1}\) = \(\frac{-1\pm \sqrt{- 3}}{2}\) = -\(\frac{1}{2}\) ± i\(\frac{√3}{2}\)
Therefore, the three cube roots of unity are
1, -\(\frac{1}{2}\) + i\(\frac{√3}{2}\) and -\(\frac{1}{2}\) - i\(\frac{√3}{2}\)
among them 1 is real number and the other two are conjugate complex numbers and they are also known as imaginary cube roots of unity.
Properties of the cube roots of unity:
Property I: Among the three cube roots of unity one of the cube roots is real and the other two are conjugate complex numbers.
The three cube roots of unity are 1, -\(\frac{1}{2}\) + i\(\frac{√3}{2}\) and -\(\frac{1}{2}\) - i\(\frac{√3}{2}\).
Hence, we conclude that from the cube roots of unity we get 1 is real and the other two i.e., \(\frac{1}{2}\) + i\(\frac{√3}{2}\) and -\(\frac{1}{2}\) - i\(\frac{√3}{2}\) are conjugate complex numbers.
Property II: Square of any one imaginary cube root of unity is equal to the other imaginary cube root of unity.
\((\frac{-1 + \sqrt{3}i}{2})^{2}\) = \(\frac{1}{4}\)[(- 1)^2 - 2 ∙ 1 ∙ √3i + (√3i)\(^{2}\)]
= \(\frac{1}{4}\)[1 - 2√3i - 3]
= \(\frac{-1 - \sqrt{3}i}{2}\),
And \((\frac{-1 - \sqrt{3}i}{2})^{2}\) = \(\frac{1}{4}\)[(1^2 + 2 ∙ 1 ∙ √3i + (√3i)\(^{2}\)]
= \(\frac{1}{4}\)[1 + 2√3 i - 3]
= \(\frac{-1 + \sqrt{3}i}{2}\),
Hence, we conclude that square of any cube root of unity is equal to the other.
Therefore, suppose ω\(^{2}\) is one imaginary cube root of unity then the other would be ω.
Property III: The product of the two imaginary cube roots is 1 or, the product of three cube roots of unity is 1.
Let us assume that, ω = \(\frac{-1 - \sqrt{3}i}{2}\); then, ω\(^{2}\) = \(\frac{-1 + \sqrt{3}i}{2}\)
Therefore, the product of the two imaginary or complex cube roots = ω ∙ ω\(^{2}\) = \(\frac{-1 - \sqrt{3}i}{2}\) × \(\frac{-1 + \sqrt{3}i}{2}\)
Or, ω\(^{3}\) = \(\frac{1}{4}\)[(-1)\(^{2}\) - (√3i)\(^{2}\)] = \(\frac{1}{4}\)[1 - 3i\(^{2}\)] = \(\frac{1}{4}\)[1 + 3] = \(\frac{1}{4}\) × 4 = 1.
Again, the cube roots of unity are 1, ω, ω\(^{2}\). So, product of cube roots of unity = 1 ∙ ω ∙ ω\(^{2}\) = ω\(^{3}\) = 1.
Therefore, product of the three cube roots of unity is 1.
Property IV: ω\(^{3}\) = 1
We know that ω is a root of the equation z\(^{3}\) - 1 = 0. Therefore, ω satisfies the equation z\(^{3}\) - 1 = 0.
Consequently, ω\(^{3}\) - 1 = 0
or, ω = 1.
Note: Since ω\(^{3}\) = 1, hence, ω\(^{n}\) = ω\(^{m}\), where m is the least non-negative remainder obtained by dividing n by 3.
Property V: The sum of the three cube roots of unity is zero i.e., 1 + ω + ω\(^{2}\) = 0.
We know that, the sum of the three cube roots of unity = 1 + \(\frac{-1 - \sqrt{3}i}{2}\) + \(\frac{-1 + \sqrt{3}i}{2}\)
Or, 1 + ω + ω\(^{2}\) = 1 - \(\frac{1}{2}\) + \(\frac{√3}{2}\)i - \(\frac{1}{2}\) - \(\frac{√3}{2}\)i = 0.
Notes:
(i) The cube roots of 1 are 1, ω, ω\(^{2}\) where, ω = \(\frac{-1 - \sqrt{3}i}{2}\) or, \(\frac{-1 + \sqrt{3}i}{2}\)
(ii) 1 + ω + ω\(^{2}\) = 0 ⇒ 1 + ω = - ω\(^{2}\), 1 + ω\(^{2}\) = - ω and ω + ω\(^{2}\) = -1
(iii) ω\(^{4}\) = ω\(^{3}\) ∙ ω = 1 ∙ ω = ω;
ω\(^{5}\) = ω\(^{3}\) ∙ ω\(^{2}\) = 1 ∙ ω\(^{2}\) = ω\(^{2}\);
ω\(^{6}\) = (ω\(^{3}\))\(^{2}\) = (1)\(^{2}\) = 1.
In general, if n be a positive integer then,
ω\(^{3n}\) = (ω\(^{3}\))\(^{n}\) = 1\(^{n}\) = 1;
ω\(^{3n + 1}\) = ω\(^{3n}\) ∙ ω = 1 ∙ ω = ω;
ω\(^{3n + 2}\) = ω\(^{3n}\) ∙ ω\(^{2}\) = 1 ∙ ω\(^{2}\) = ω\(^{2}\).
Property VI: The reciprocal of each imaginary cube roots of unity is the other.
The imaginary cube roots of unity are ω and ω\(^{2}\), where ω = \(\frac{-1 + \sqrt{3}i}{2}\).
Therefore, ω ∙ ω\(^{2}\) = ω\(^{3}\) = 1
⇒ ω = \(\frac{1}{ω^{2}}\) and ω\(^{2}\) = \(\frac{1}{ω}\)
Hence, we conclude that the reciprocal of each imaginary cube roots of unity is the other.
Property VII: If ω and ω\(^{2}\) are the roots of the equation z\(^{2}\) + z + 1 = 0 then - ω and - ω\(^{2}\) are the roots of the equation z\(^{2}\) - z + 1 = 0.
Property VIII: Cube roots of -1 are -1, - ω and - ω\(^{2}\).
11 and 12 Grade Math
From The Cube Roots of Unity to HOME PAGE
Didn't find what you were looking for? Or want to know more information about Math Only Math. Use this Google Search to find what you need.
Recent Articles
-
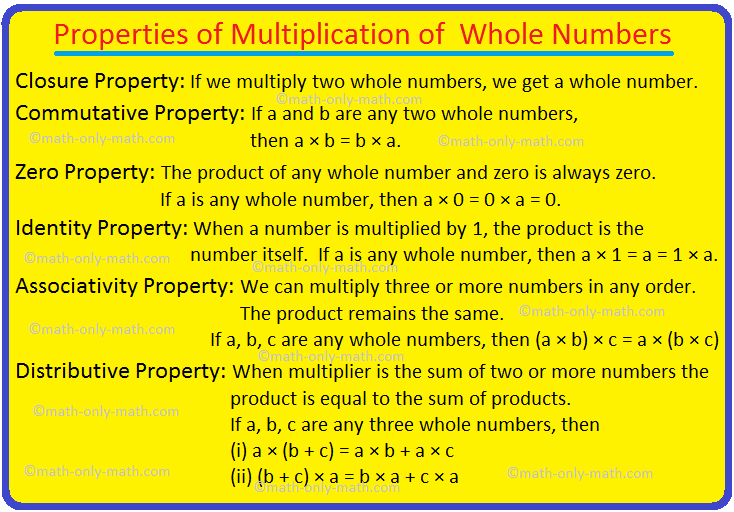
Properties of Multiplication | Multiplicative Identity | Whole Numbers
Jan 15, 25 12:08 AM
There are six properties of multiplication of whole numbers that will help to solve the problems easily. The six properties of multiplication are Closure Property, Commutative Property, Zero Property… -
Multiplication Table | Learn Tables from 0 – 25 | Multiplication Table
Jan 14, 25 11:53 PM
In math multiplication table we will learn the tables from 0 – 25. These multiplication tables help the students to learn the essential multiplication facts. Multiplication tables are very important f… -
3rd Grade Math Worksheets |3rd Grade Math Sheets|3rd Grade Math Lesson
Jan 14, 25 11:02 PM
3rd grade math worksheets is carefully planned and thoughtfully presented on mathematics for the students. Teachers and parents can also follow the worksheets to guide the students. -
3rd Grade Subtraction Worksheet | 3-Digit Subtraction Worksheets | Ans
Jan 14, 25 01:57 PM
In 3th Grade Addition Worksheet we will solve how to subtract 3-digit numbers by expansion, subtraction of 3-digit numbers without regrouping, subtraction of 3-digit numbers with regrouping, propertie… -
Facts about Subtraction | Subtraction of Small Numbers|Solved Examples
Jan 14, 25 12:29 AM
The operation to finding the difference between two numbers is called subtraction. Let us know some facts about subtraction which will help us to learn subtraction of large numbers. 1. Subtraction wit…


New! Comments
Have your say about what you just read! Leave me a comment in the box below. Ask a Question or Answer a Question.