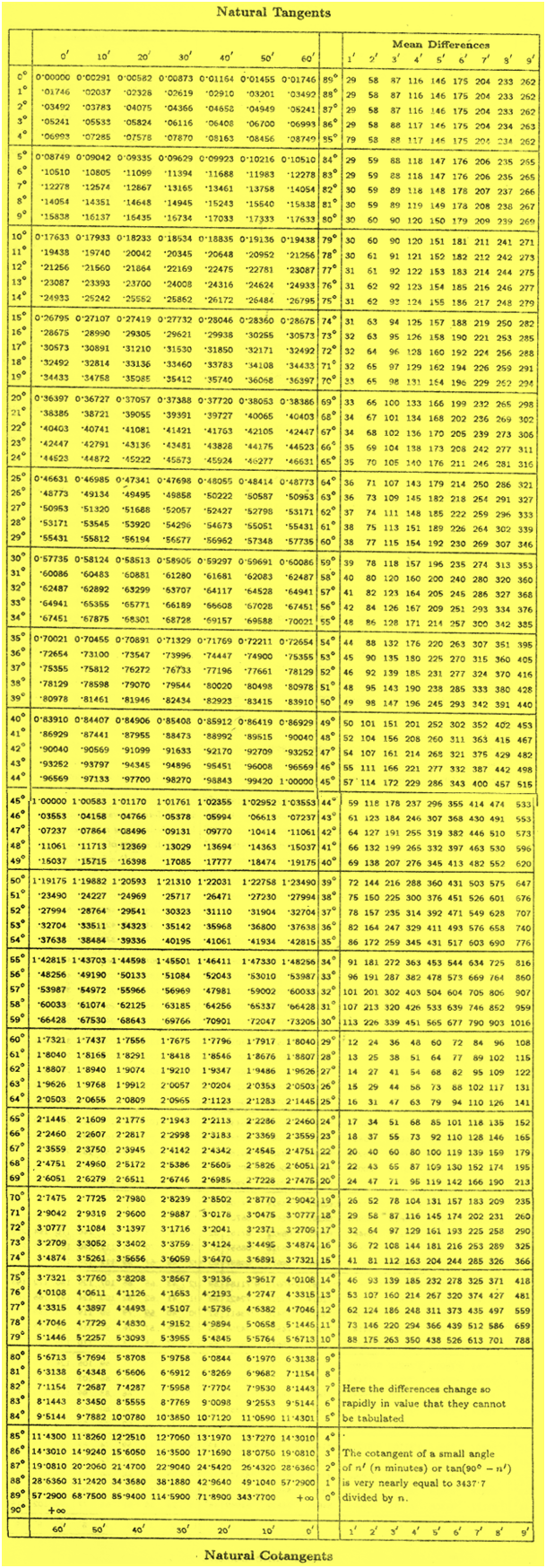
Table of Tangents and Cotangents

We will discuss here the method of using the table of tangents and cotangents.

This table shown below is also known as the table of natural tangents and natural cotangents.

Trigonometric Table of Tangents and Cotangents

Using the table we can find the values of tangents and cotangents of angles ranging from 0° to 90° at intervals of 1'.

We can observe that the table of natural tangents and natural cotangents are generally divided into the following parts. They are the following:

(i) In the extreme left vertical column of the table the angles are from 0° to 90° at intervals of 1°.

(b) In another vertical column about the middle of the table the angles are from 89° to 0° at intervals of 1°.

(ii) In the horizontal row at the top of the table the angles are from 0' to 60' at intervals of 10'.

(iii) In the horizontal row at the bottom of the table the angles are from 60' to 0' at intervals of 10'.

(iv) In the horizontal row at the extreme right of the table the angles are from 1' to 9’ at intervals of 1'. This part of the table is known as Mean Difference Column.

Note:

(i) From the table we get the tangents or cotangents value of any given angle correct to five decimal places.

(ii) We know that the tangents of any given angle is equal to that of cotangents of its complementary angle [i.e., sin θ = cos (90° - θ)]. So, the table is drawn in such a way that we can use the table to find the sin and cosine value of any given angle between 0° and 90°.

Solved examples using the table of natural tangents and natural cotangents:

1. Using table of natural tangents, find the value of tan 59°.

Solution:

To find the value of tan 59° by the using the table of natural tangents we need to go through the extreme left vertical column 0° to 90° and move downwards till we reach the angle 59°.

Then we move horizontally to the right at the top of the column headed by 0' and read the figure 1.66428, which is the require value of tan 59°.

Therefore, tan 59° = 1.66428

2. Using table of natural cosines, find the value of cot 19°

Solution:

To find the value of cot 19° by the using the table of natural cotangents we need to go through the vertical column about the middle of the table 89° to 0° and move upwards till we reach angle 19°.

Then we move horizontally to the left at the bottom of the row above the column 0' and read the figure 2.9042, which is the require value of cot 19°.

Therefore, cot 19° = 2.9042

3. Using the trigonometric table, find the value of tan 78°60'

Solution:

To find the value of tan 78°60' by the using the table of natural tangents and natural cotangents we need to go through the extreme left vertical column 0° to 90° and move downwards till we reach the angle 78°.

Then we move horizontally to the right at the top of the column headed by 60' and read the figure 5.1446, which is the require value of tan 78°60'.

Therefore, tan 78°60' = 5.1446

4. Using table of natural cotangents, find the value of cot 61°10'               

Solution:

To find the value of cot 61°10' by the using the table of natural cotangents we need to go through the vertical column about the middle of the table 89° to 0° and move upwards till we reach angle 61°.

Then we move horizontally to the left at the bottom of the row above the column 10’ and read the figure 0.55051, which is the require value of 61°10'.

Therefore, 61°10' = 0.55051

5. Using the trigonometric table, find the value of tan 21°39'

Solution:

To find the value of tan 21°39’ by the using the trigonometric table table of natural tangents and natural cotangents we need to first find the value of tan 21°30'.

To find the value of tan 21°30' by the using the table of natural tangents we need to go through the extreme left vertical column 0° to 90° and move downwards till we reach the angle 21°.

Then we move horizontally to the right at the top of the column headed by 30' and read the figure 0.39391, which is the require value of tan 21°30'.

Therefore, tan 21°30' = 0.39391

Now we move further right along the horizontal line of angle 21° to the column headed by 9' of mean difference and read the figure 302 there; this figure of the table does not contain decimal sign. In fact, 302 implies 0.00302. Now we know that when the value of an angle increases from 0° to 90°, its tangent value increases continually from 0 to 1. Therefore, to find the value of tan 21°39' we need to add the value corresponding to 9' with the value of tan 21°30'.

Therefore, tan 21°39' = sin (tan 21°30' + 9') = 0.39391 + 0.00302 = 0.39693

6. Using the trigonometric table, find the value of cot 67°68'

Solution:

To find the value of cot 67°68' by the using the trigonometric table of natural cotangents and natural cosines we need to first find the value of cot 67°60’

To find the value of cot 67°60' by the using the table of natural cotangents we need to go through the vertical column about the middle of the table 89° to 0° and move upwards till we reach angle 67°.

Then we move horizontally to the left at the bottom of the row above the column 60’ and read the figure 0.40403, which is the require value of cot 67°60'.

Therefore, cot 67°60' = 0.40403

Now we move further right along the horizontal line of angle 67° to the column headed by 8' of mean difference and read the figure 273 there; this figure of the table does not contain decimal sign. In fact this figure 273 implies 0∙00273. We know that when the value of an angle increases from 0° to 90°, its cotangents value decreases continually from 1 to 0. Therefore, to find the value of cot 67°68' we need to subtract the value corresponding to 8’ from the value of cot 67°60'

Therefore, cot 67°68' = cos (67°60' + 8') = 0.40403 - 0∙00273 = 0.4013

● Trigonometrical Table

• Table of Sines and Cosines
• Table of Tangents and Cotangents

11 and 12 Grade Math

From Table of tangents and Cotangents to HOME PAGE