Sum of an infinite Geometric Progression
The sum of an infinite Geometric Progression whose first term 'a' and common ratio 'r' (-1 < r < 1 i.e., |r| < 1) is
S = a1−r
Proof:
A series of the form a + ar + ar2 + ...... + arn + ............... ∞ is called an infinite geometric series.
Let us consider an infinite Geometric Progression with first term a and common ratio r, where -1 < r < 1 i.e., |r| < 1. Therefore, the sum of n terms of this Geometric Progression in given by
Sn = a(1−rn1−r) = a1−r - arn1−r ........................ (i)
Since - 1< r < 1, therefore rn decreases as n increases and r^n tends to
zero an n tends to infinity i.e., rn → 0 as n → ∞.
Therefore,
arn1−r → 0 as n → ∞.
Hence, from (i), the sum of an infinite Geometric Progression ig given by
S = limx→0 Sn = limx→∞(a1−r−ar21−r) = a1−r if |r| < 1
Note: (i) If an infinite series has a sum, the series is said to be convergent. On the contrary, an infinite series is said to be divergent it it has no sum. The infinite geometric series a + ar + ar2 + ...... + arn + ............... ∞ has a sum when -1 < r < 1; so it is convergent when -1 < r < 1. But it is divergent when r > 1 or, r < -1.
(ii) If r ≥ 1, then the sum of an infinite Geometric Progression tens to infinity.
Solved examples to find the sum to infinity of the Geometric Progression:
1. Find the sum to infinity of the Geometric Progression
-54, 516, -564, 5256, .........
Solution:
The given Geometric Progression is -54, 516, -564, 5256, .........
It has first term a = -54 and the common ratio r = -14. Also, |r| < 1.
Therefore, the sum to infinity is given by
S = a1−r = 541−(−14) = -1
2. Express the recurring decimals as rational number: 3˙6
Solution:
3˙6 = 0.3636363636............... ∞
= 0.36 + 0.0036 + 0.000036 + 0.00000036 + .................. ∞
= 36102 + 36104 + 36106 + 36108 + .................. ∞, which is an infinite geometric series whose first term = 36102 and common ratio = 1102 < 1.
= 361021−1102, [Using the formula S = a1−r]
= 361001−1100
= 36100100−1100
= 3610099100
= 36100 × 10099
= 411
● Geometric Progression
- Definition of Geometric Progression
- General Form and General Term of a Geometric Progression
- Sum of n terms of a Geometric Progression
- Definition of Geometric Mean
- Position of a term in a Geometric Progression
- Selection of Terms in Geometric Progression
- Sum of an infinite Geometric Progression
- Geometric Progression Formulae
- Properties of Geometric Progression
- Relation between Arithmetic Means and Geometric Means
- Problems on Geometric Progression
11 and 12 Grade Math
From Sum of an infinite Geometric Progression to HOME PAGE
Didn't find what you were looking for? Or want to know more information about Math Only Math. Use this Google Search to find what you need.
Recent Articles
-
5th Grade BODMAS Rule Worksheet | PEMDAS | Order of operations|Answers
Apr 03, 25 03:11 PM
In 5th Grade BODMAS Rule Worksheet you will get different types of problems on mathematical expressions involving different operations, mathematical expression with 'brackets' and 'of' and simplifying… -
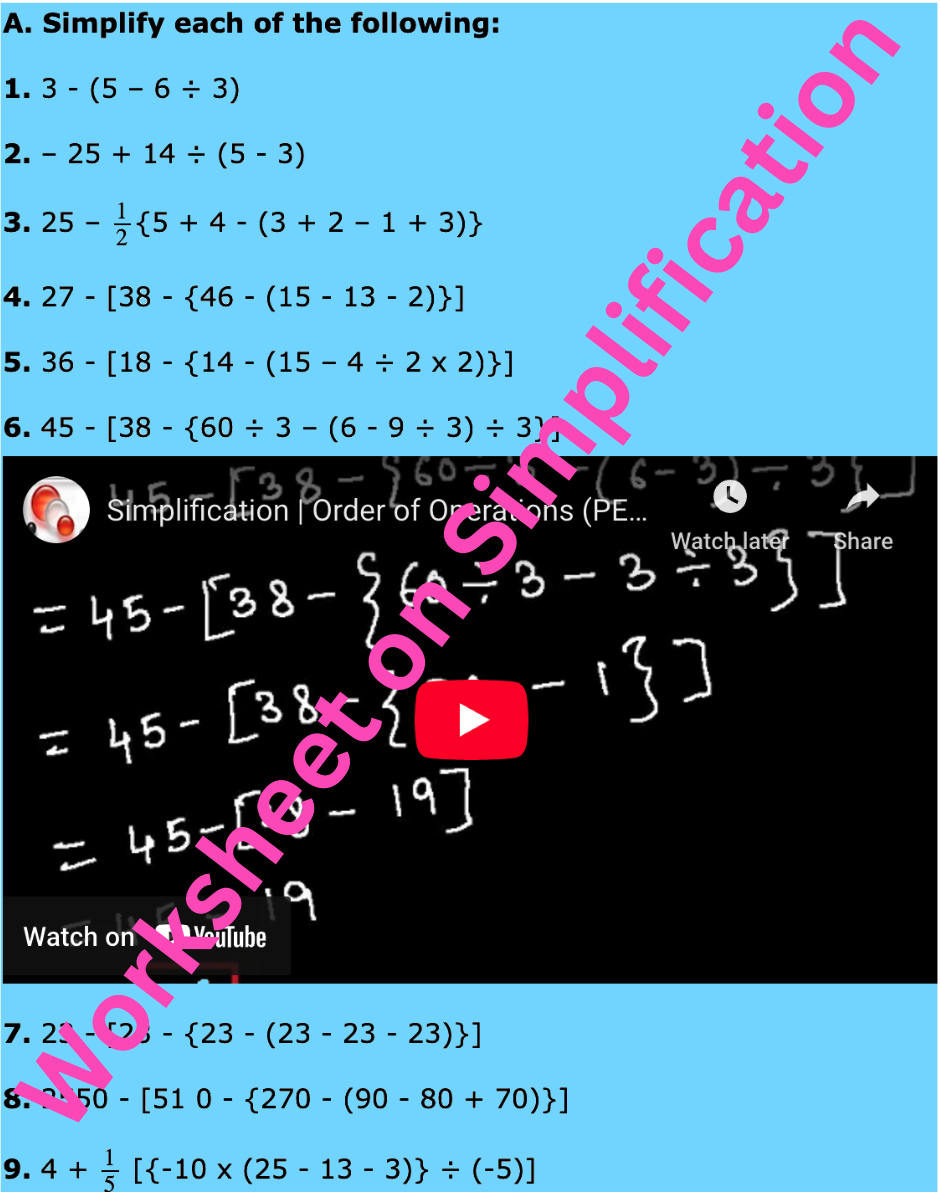
Worksheet on Simplification | Simplify Expressions | BODMAS Questions
Apr 03, 25 02:58 PM
In worksheet on simplification, the questions are based in order to simplify expressions involving more than one bracket by using the steps of removal of brackets. This exercise sheet -
Divisible by 2 Video |Test of Divisibility by 2 Trick| Rules| Examples
Apr 03, 25 10:25 AM
A number is divisible by 2 if the digit at unit place is either 0 or multiple of 2. So a number is divisible by 2 if digit at its units place is 0, 2, 4, 6 or 8. -
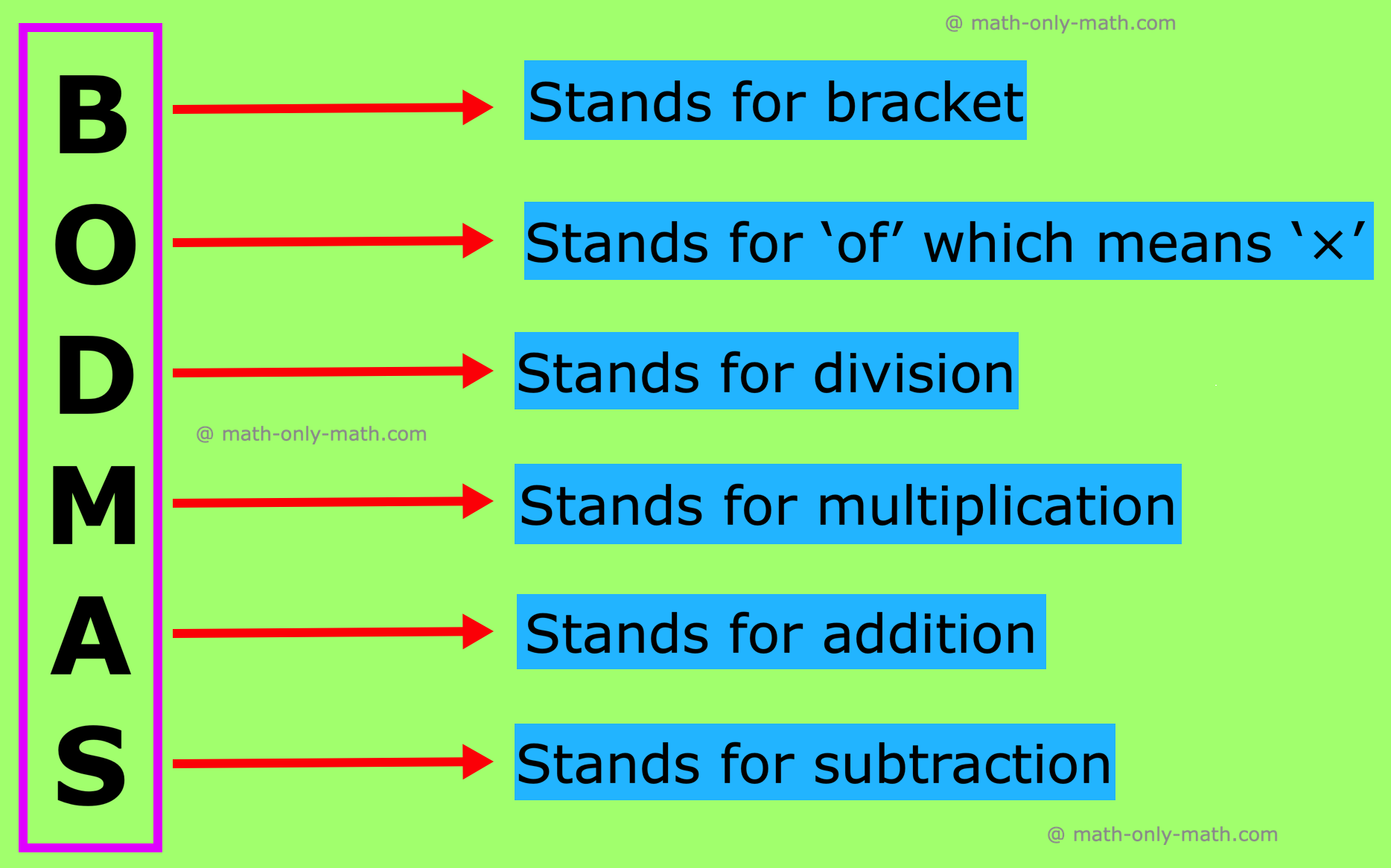
BODMAS Rule | Order of Operation | Definition, Examples, Problems
Apr 03, 25 10:22 AM
Easy and simple way to remember BODMAS rule!! B → Brackets first (parentheses) O → Of (orders i.e. Powers and Square Roots, Cube Roots, etc.) DM → Division and Multiplication -
Before and After Video | Math Worksheets on Number | Before and After
Apr 03, 25 12:44 AM
Free math worksheets on numbers before and after help the kids to check how much they are good at numbers. The purpose of this math activity is to help your child to say a number in order and also hel




New! Comments
Have your say about what you just read! Leave me a comment in the box below. Ask a Question or Answer a Question.