Secant and Tangent

Here we will learn about Secant and Tangent.

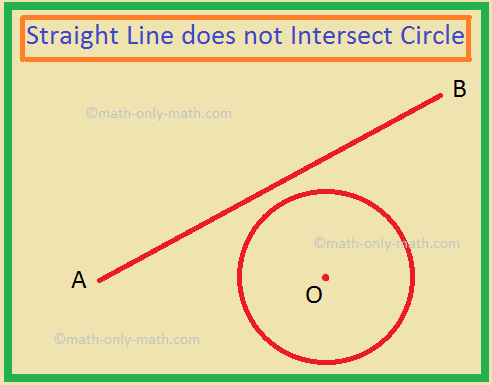
Let a circle with centre O and a straight line AB be drawn on the same plane. Then only one of the following cases is possible:

(i) The straight line AB does not touch or cut the circle at any point.

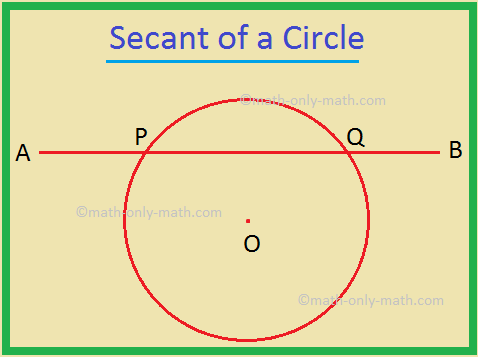
(ii) The straight line AB cuts the circle at two points P and Q.

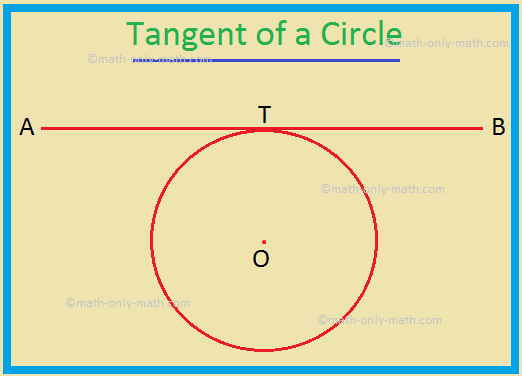
(iii) The straight line AB touches the circle at only one point T.

A straight line which cuts a circle in two distinct points is called a secant to the circle.

In case (ii) above, AB is a secant to the given circle.

A straight line which touches a circle at only one point is called a tangent to the circle. The point at which it touches the circle is known as the point of contact.

In case (iii) above, AB is a tangent to the circle and T is the point of contact. Any other point on the tangent lies outside the circle.

Note:

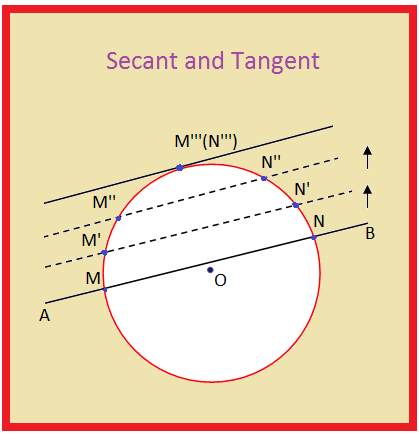
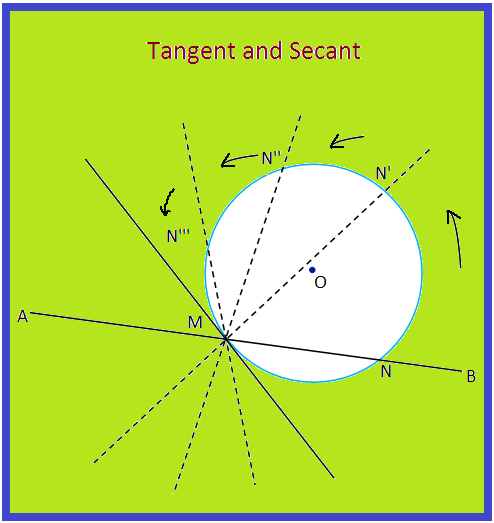
Let a secant cut a circle a circle at P and Q, and move away from the centre O in such a way it remains parallel to its original position.

 We can see that as the line moves away from the centre, the two points of intersection come closer to each other and finally coincide. At this ultimate position, when P and Q coincide to become one point, the secant AB becomes a tangent to the circle at the point P’’’ (or Q’’’).

Let us consider another case in which the secant AB cuts the circle at two points P and Q. 

Suppose the secant AB is turned about the point P such that P remains fixed and Q moves closer and closer to P, and ultimately coincides with P. At this position, the secant AB becomes a tangent to the circle at the point P.

10th Grade Math

From Secant and Tangent to HOME PAGE