Reflection of a Point in Origin
How to find the co-ordinates of the reflection of a point in origin?
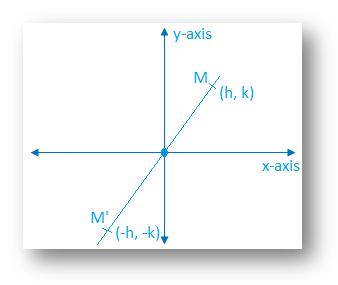
To find the co-ordinates in the adjoining figure, origin represents the plane mirror. M is the any point in the first whose co-ordinates are (h, k). When point M is reflected in the origin, the image M’ is formed in the third quadrant whose co-ordinates are (-h, -k).
Thus, we conclude that when a point is reflected in origin, both x-c-ordinate and y-co-ordinate become negative. Thus, the image of M (h, k) is M’ (-h, -k).
Rules to find the reflection of a point in the origin:
(i) Change the sign of abscissa i.e., x-coordinate.
(ii) Change the sign of ordinate i.e., y-coordinate.
For example:
1. The reflection of the point A (5, 7) in the origin is the point A' (-5, -7).
2. The reflection of the point B (-5, 7) in the origin is the point B' (5, -7).
3. The reflection of the point C (-5, -7) in the origin is the point C' (5, 7).
4. The reflection of the point D (5, -7) in the origin is the point D' (-5, 7).
5. The reflection of the point E (5, 0) in the origin is the point E' (-5, 0).
6. The reflection of the point F (0, 7) in the origin is the point F' (0, -7).
7. The reflection of the point G (-5, 0) in the origin is the point G' (5, 0).
8. The reflection of the point H (0, -7) in the origin is the point H' (0, 7).
Worked-out examples to find the co-ordinates of the reflection of a point in origin:
1. What is the reflection of the following in origin?
(i) P (1, 4)
(ii) Q (-3, -7)
(iii) R (-5, 8)
(iv) S (6, -2)
Solution:
(i) The image of P (1, 4) is P’ (-1, -4).
(ii) The image of Q (-3, -7) is Q’ (3, 7).
(iii) The image of R (-5, 8) is R’ (5, -8).
(iv) The image of S (6, -2) is S’ (-6, 2).
Note:
Thus, we conclude that the origin acts as a plane mirror. M is the point whose co-ordinates are (h, k).
The image of M, i.e., M’ lies in the third quadrant and the co-ordinates of M’ are (h, -k).
● Related Concepts
● Order of Rotational Symmetry
● Reflection of a Point in x-axis
● Reflection of a Point in y-axis
● Rotation
● 90 Degree Clockwise Rotation
● 90 Degree Anticlockwise Rotation
7th Grade Math Problems
8th Grade Math Practice
From Reflection of a Point in Origin to HOME PAGE
Didn't find what you were looking for? Or want to know more information about Math Only Math. Use this Google Search to find what you need.
Recent Articles
-
Word Problems on 4-Digit Numbers |Addition and Subtraction of 4-Digits
Jan 10, 25 02:49 PM
We will solve here some of the word problems on addition and subtraction of 4-digit numbers. We will apply the same method while adding and subtracting the word problems. 1. In a village, there are 25… -
Addition of 10, 100 and 1000 | Adding 10 | Adding 100 | Adding 1000
Jan 10, 25 01:20 AM
Here we will learn Addition of 10, 100 and 1000 with the help of different examples. -
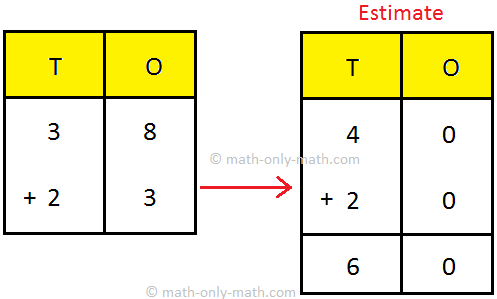
Estimating a Sum | Round the Number | Numbers by Rounding | Estimating
Jan 10, 25 12:10 AM
We will learn the basic knowledge for estimating a sum. Here we will learn an easy way to estimate a sum of two numbers by rounding. In case of two digit numbers we can only round the number -
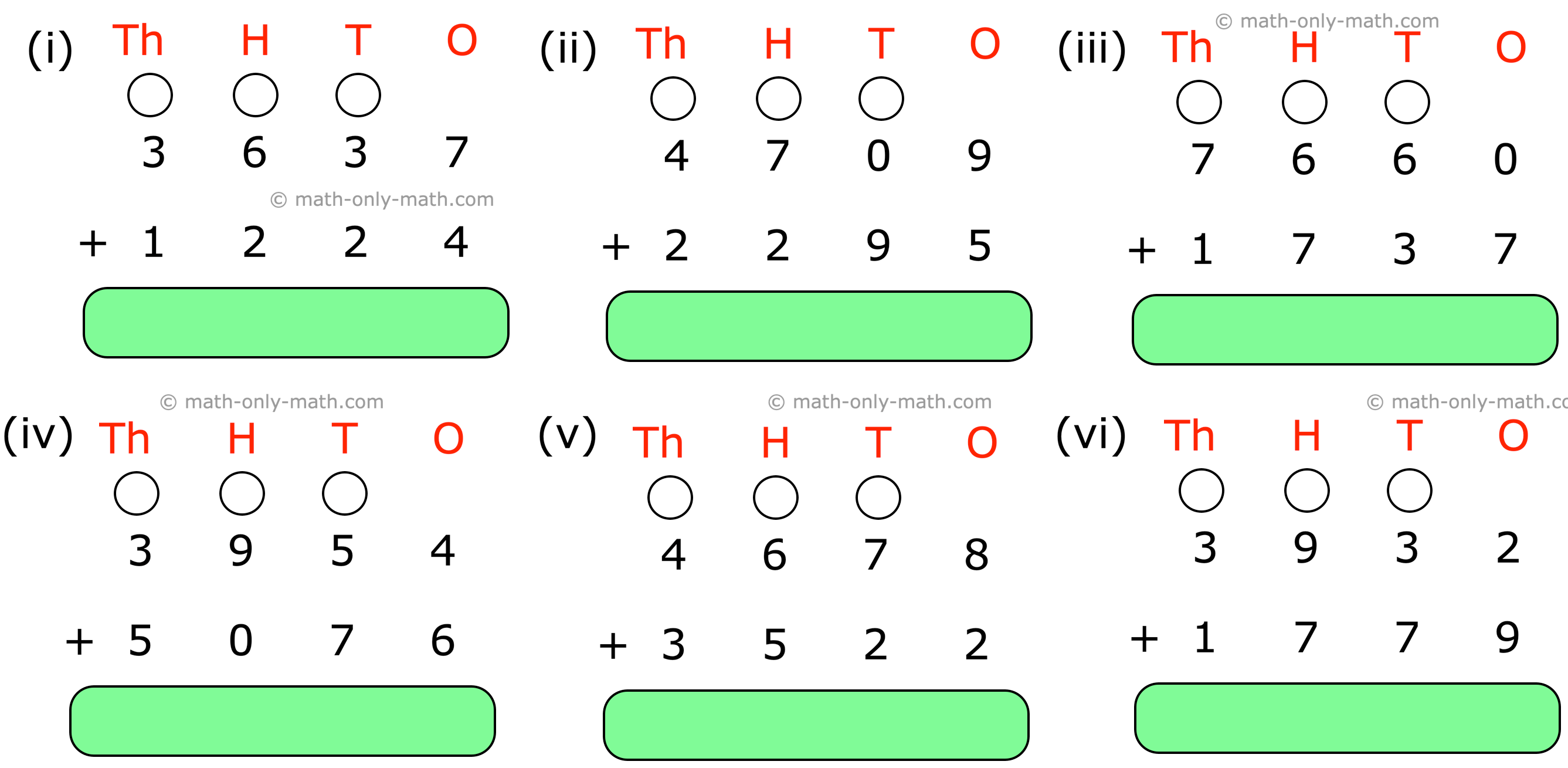
Worksheet on Addition of 4-Digit Numbers | 4 Digit Addition Worksheets
Jan 09, 25 10:50 PM
Practice the questions given in the worksheet on addition of 4-digit numbers. Here we will add two 4-digit numbers (without carrying and with carrying) and three 4-digit numbers -
Addition of 4-Digit Numbers | 4-Digit Addition |Adding 4-Digit Numbers
Jan 09, 25 10:07 PM
We will learn about the addition of 4-digit numbers (without carrying and with carrying). We know how to add 2 or 3, 3-digit numbers without carrying or with carrying.

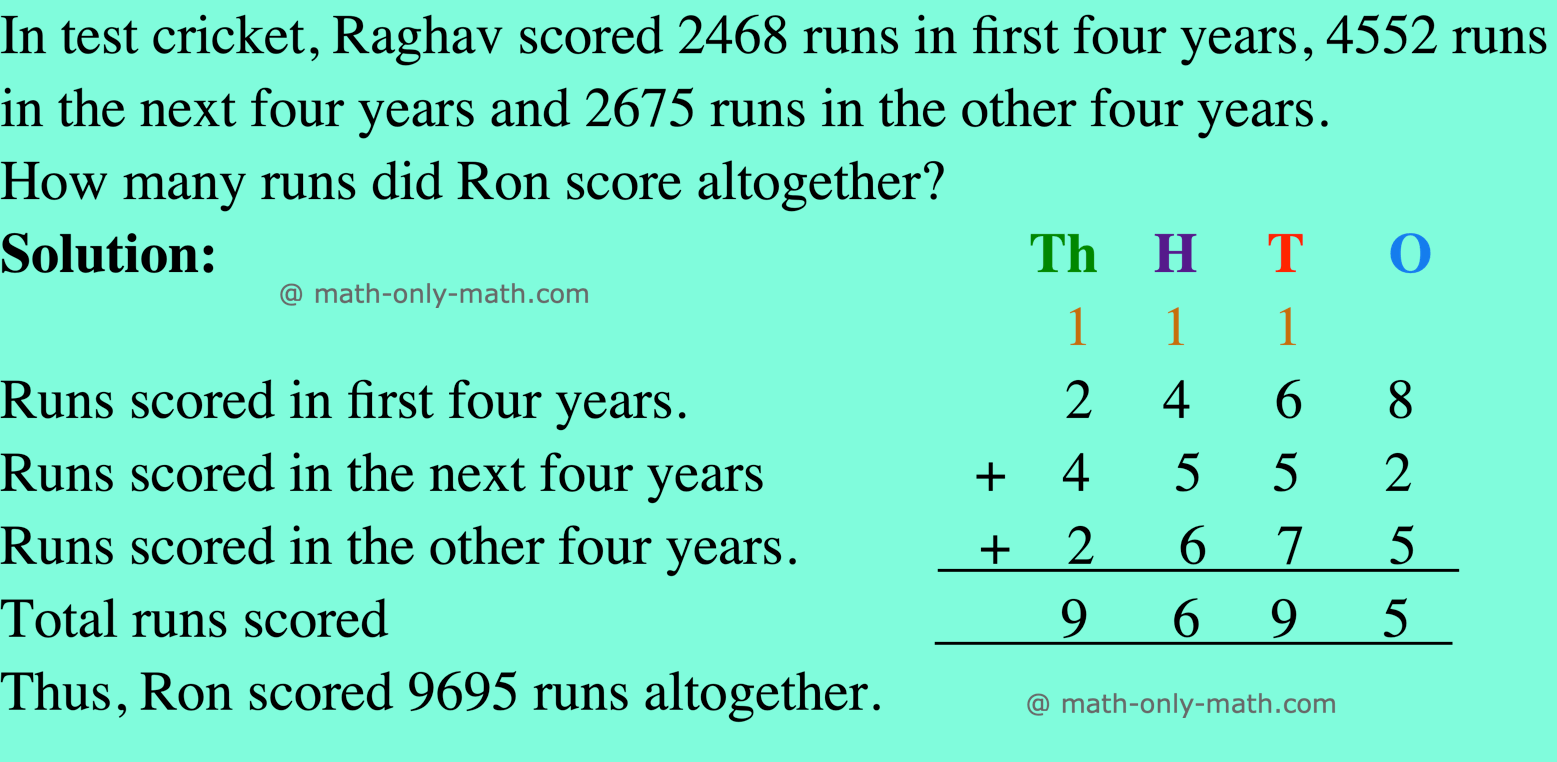


New! Comments
Have your say about what you just read! Leave me a comment in the box below. Ask a Question or Answer a Question.