Recurring Decimals as Rational Numbers
From the previous concept of rational numbers, we are clear about the meaning of rational number. A rational number is a number in pq form where ‘p’ and q’ are the integers and ‘q’ is not equal to zero. Both ‘p’ and ‘q’ could be negative as well as positive. We have also seen as to how rational numbers can be converted to both terminating and non-terminating decimal numbers. Now, non- terminating decimal numbers can be further classified into two types which are recurring and non- recurring decimal numbers.
Recurring numbers: Recurring numbers are those numbers which keep on repeating the same value after decimal point. These numbers are also known as repeating decimals.
For example:
13 = 0.333...... (3 repeats forever)
17 = 0.142857142857....... (14285714 repeats forever)
77600= 0.128333...... (3 repeats forever)
To show a repeating digits in a decimal number, often we put a dot or a line above the repeating digit as given below:
For Example:
13 = 0.333..… = 0.˙3 = 0.¯3
Non- recurring numbers: Non- recurring numbers are those, which do not repeat their values after decimal point. They are also known as non- terminating and non- repeating decimal numbers.
For Example:
√2 = 1.4142135623730950488016887242097…...
√3 = 1.7320508075688772935274463415059…...
π = 3.1415926535897932384626433832795….....
e = 2.7182818284590452353602874713527….....
In previous topic, we have already seen how to convert rational numbers into decimal fractions (may it be terminating or non- terminating decimal number). In this topic we’ll try to understand the steps involved in conversion of recurring (or repeating) decimal numbers into rational fractions. The steps involved are as follows:-
Step I: Let us assume ‘x’ to be the repeating decimal number we are trying to convert into rational number.
Step II: Carefully examine the repeating decimal to find the repeating digits.
Step III: Place the repeating digits to the left of decimal point.
Step IV: After step 3 place the repeating digits to the right of decimal point.
Step V: Now subtract left sides of the two equations. Then, subtract the right sides of the two equations. As we subtract, just make sure the differences of both the sides are positive.
For having a better understanding let’s have look at some of the examples as shown below:
1. Convert 0.7777… into rational fraction.
Solution:
Step I: x = 0.7777
Step II: After examining we find that repeating digit is 7.
Step III: Place the repeating digit (7) to the left of decimal point. To do so, we need to move the decimal point 1 place to the right. This can also be done by multiplying the given no. by 10.
So, 10x = 7.777
Step IV: After step 3 place the repeating digits to the right of decimal point. In this case if we place the repeating digits to the right of decimal point it becomes the original number.
x = 0.7777
Step V: The two equations are-
x = 0.7777,
⟹ 10x = 7.777
Now we have to subtract the right and left hand sides-
10x - x = 7.777- 0.7777
⟹ 9x = 7.0
⟹ x = 79
Hence, x= 79 is the required rational number.
2. Convert 4.567878….. into rational fraction.
Solution:
The conversion of the given decimal number into rational fraction can be carried out by using following conversion steps:
Step I: Let x = 4.567878…
Step II: After examining we find that the repeating digits are ‘78’.
Step III: Now we place the repeating digits ‘78’ to the left of decimal point. To do so we need to shift the decimal point to the right by 4 places. This can be done by multiplying the given number by’10,000’.
10,000x = 45678.787878
Step IV: Now we need to shift the repeating digits to the left of the decimal point in the original decimal number.to do so we need to multiply the original number by ‘100’.
100x = 456.787878
Step V: Now the two equations become:
10,000x = 45678.787878, and
100x = 456.787878
Step VI: Now we have two subtract both the left and right hand sides of the two equations and equate them so that the equality remains the same.
10,000x - 100x = 45678.787878 - 456.787878
⟹ 9,900x = 45,222
⟹ x = 452229900
This rational fraction can further reduced to
x = 75371650 (divide both numerator and denominator by 6)
So, the rational conversion of the given decimal number is 75371650.
All the conversion of this type can be carried out by using the above mentioned steps carefully.
Short-cut method of Conversion of recurring decimal to rational numbers
The method of conversion of recurring decimals in the form p/q is as follows.
Recurring decimal =
The whole number obtained by writing the digits in their order - The whole number made by the nonrecurring digits in order10The number of digits after the decimal point−10The number of digits after the decimal point that do not recur
For example:
Express 15.0˙2 as a rational number.
Solution:
Here, the whole number obtained by writing the digits in their order = 1502,
The whole number made by the nonrecurring digits in order = 150
The number of digits after the decimal point = 2 (two)
The number of digits after the decimal point that do not recur = 1 (one).
Therefore,
15.0˙2 = 1502−150102−101=1352100−10=135290
Rational Numbers
Decimal Representation of Rational Numbers
Rational Numbers in Terminating and Non-Terminating Decimals
Recurring Decimals as Rational Numbers
Laws of Algebra for Rational Numbers
Comparison between Two Rational Numbers
Rational Numbers Between Two Unequal Rational Numbers
Representation of Rational Numbers on Number Line
Problems on Rational numbers as Decimal Numbers
Problems Based On Recurring Decimals as Rational Numbers
Problems on Comparison Between Rational Numbers
Problems on Representation of Rational Numbers on Number Line
Worksheet on Comparison between Rational Numbers
Worksheet on Representation of Rational Numbers on the Number Line
From Recurring Decimals as Rational Numbers to HOME PAGE
Didn't find what you were looking for? Or want to know more information about Math Only Math. Use this Google Search to find what you need.
Recent Articles
-
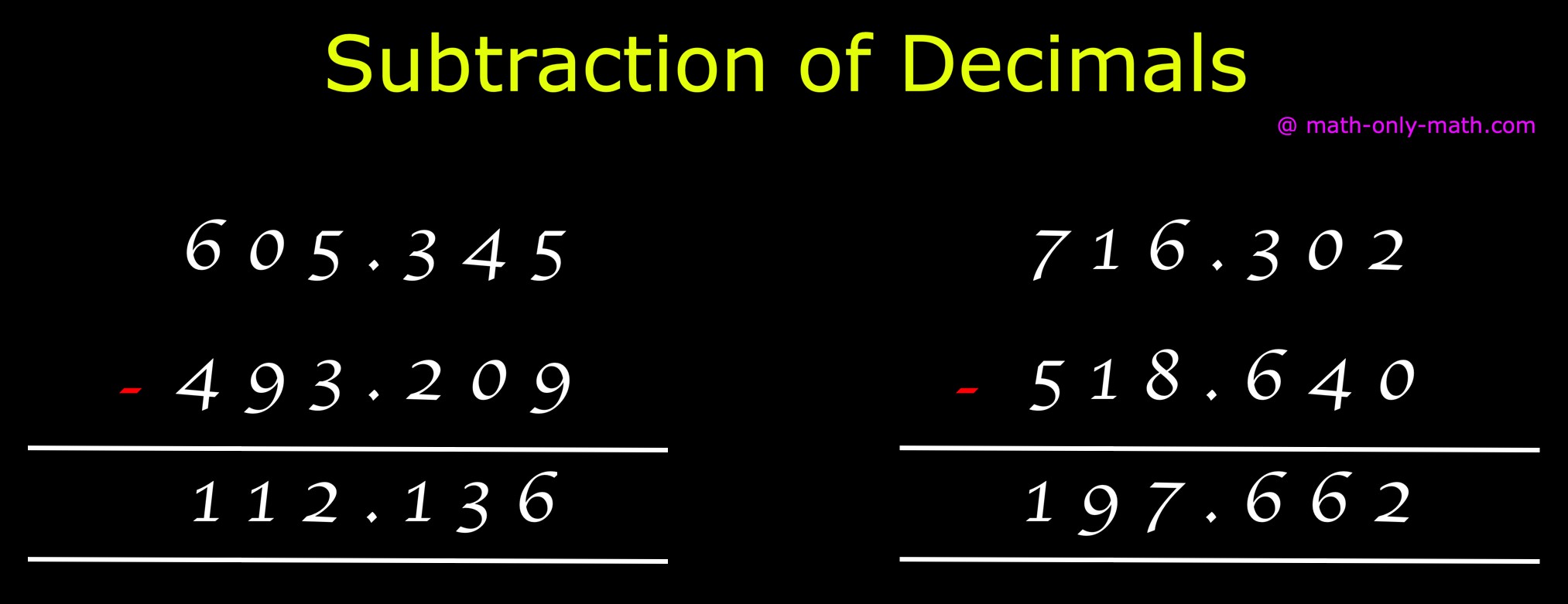
Subtraction of Decimals | Subtracting Decimals | Decimal Subtraction
Apr 24, 25 03:25 PM
We will discuss here about the subtraction of decimals. Decimals are subtracted in the same way as we subtract ordinary numbers. We arrange the digits in columns -
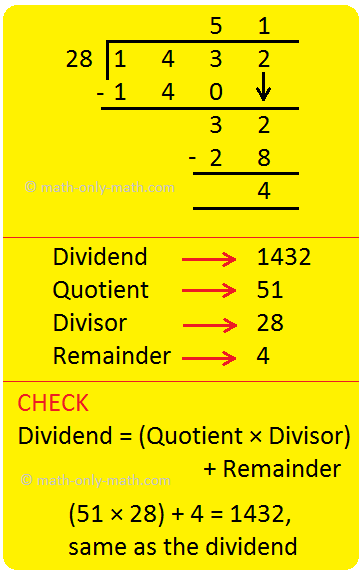
How to Do Long Division? | Method | Steps | Examples | Worksheets |Ans
Apr 24, 25 10:18 AM
As we know that the division is to distribute a given value or quantity into groups having equal values. In long division, values at the individual place (Thousands, Hundreds, Tens, Ones) are dividend… -
Division by Two-Digit Numbers | Knowledge of Estimation | Division
Apr 24, 25 10:12 AM
In division by two-digit numbers we will practice dividing two, three, four and five digits by two-digit numbers. Consider the following examples on division by two-digit numbers: Let us use our knowl… -
Addition of Decimals | How to Add Decimals? | Adding Decimals|Addition
Apr 24, 25 01:45 AM
We will discuss here about the addition of decimals. Decimals are added in the same way as we add ordinary numbers. We arrange the digits in columns and then add as required. Let us consider some -
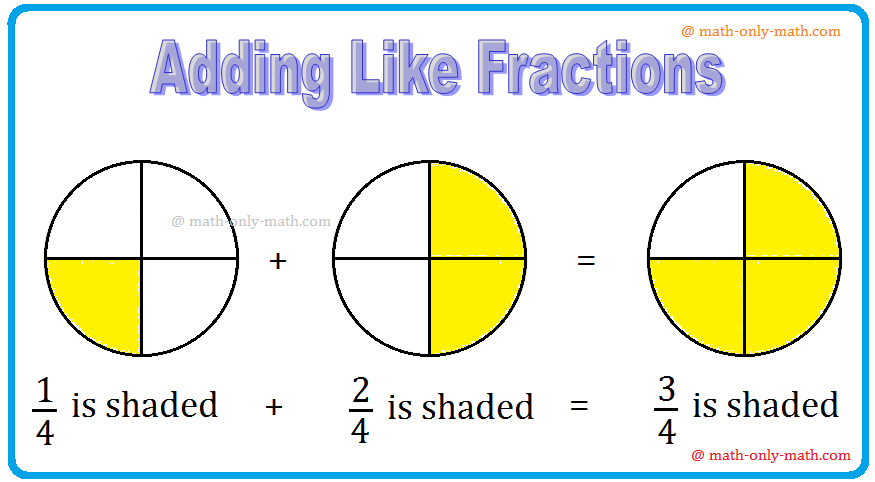
Addition of Like Fractions | Examples | Videos | Worksheet | Fractions
Apr 23, 25 09:23 AM
To add two or more like fractions we simplify add their numerators. The denominator remains same. Thus, to add the fractions with the same denominator, we simply add their numerators and write the com…



New! Comments
Have your say about what you just read! Leave me a comment in the box below. Ask a Question or Answer a Question.