Quotient Relations of Trigonometric Ratios
Quotient relations of trigonometric ratios are tan θ = sin θ/cos θ and cot θ = cos θ/sin θ.
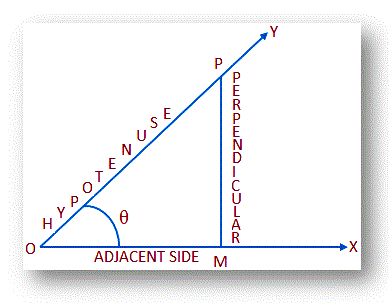
Let OMP be a right angled triangle at M and ∠MOP = θ.
According to the definition of trigonometric ratios we have,
sin θ =
perpendicular/hypotenuse = MP/OP …………..
(i)
and cos θ = adjacent/hypotenuse = OM/OP ………….. (ii)
Now dividing (i) by (ii) we get;
sin θ/cos θ = (MP/OP)/(OM/OP)
= (MP/OP) × (OP/OM)
= MP/OM
= tan θ
Therefore, tan θ = sin θ/cos θ
Again dividing (ii) by (i) we get;
cos θ/sin θ = (OM/OP)/(MP/OP)
= (OM/OP) × (OP/MP)
= OM/MP
= cot θ
Therefore, cot θ = cos θ/sin θ
The above step-by-step explanation on quotient relations of trigonometric ratios is very important to solve various problems on trigonometry.
● Trigonometric Functions
- Basic Trigonometric Ratios and Their Names
- Restrictions of Trigonometrical Ratios
- Reciprocal Relations of Trigonometric Ratios
- Quotient Relations of Trigonometric Ratios
- Limit of Trigonometric Ratios
- Trigonometrical Identity
- Problems on Trigonometric Identities
- Elimination of Trigonometric Ratios
- Eliminate Theta between the equations
- Problems on Eliminate Theta
- Trig Ratio Problems
- Proving Trigonometric Ratios
- Trig Ratios Proving Problems
- Verify Trigonometric Identities
- Trigonometrical Ratios of 0°
- Trigonometrical Ratios of 30°
- Trigonometrical Ratios of 45°
- Trigonometrical Ratios of 60°
- Trigonometrical Ratios of 90°
- Trigonometrical Ratios Table
- Problems on Trigonometric Ratio of Standard Angle
- Trigonometrical Ratios of Complementary Angles
- Rules of Trigonometric Signs
- Signs of Trigonometrical Ratios
- All Sin Tan Cos Rule
- Trigonometrical Ratios of (- θ)
- Trigonometrical Ratios of (90° + θ)
- Trigonometrical Ratios of (90° - θ)
- Trigonometrical Ratios of (180° + θ)
- Trigonometrical Ratios of (180° - θ)
- Trigonometrical Ratios of (270° + θ)
- Trigonometrical Ratios of (270° - θ)
- Trigonometrical Ratios of (360° + θ)
- Trigonometrical Ratios of (360° - θ)
- Trigonometrical Ratios of any Angle
- Trigonometrical Ratios of some Particular Angles
- Trigonometric Ratios of an Angle
- Trigonometric Functions of any Angles
- Problems on Trigonometric Ratios of an Angle
- Problems on Signs of Trigonometrical Ratios
From Quotient Relations of Trigonometric Ratios to HOME PAGE
Didn't find what you were looking for? Or want to know more information about Math Only Math. Use this Google Search to find what you need.
Recent Articles
-
2nd grade math Worksheets | Free Math Worksheets | By Grade and Topic
Nov 20, 24 01:00 PM
2nd grade math worksheets is carefully planned and thoughtfully presented on mathematics for the students. -
2nd Grade Measurement Worksheet | Measuring Length, Mass and Volume
Nov 20, 24 12:50 AM
In 2nd Grade Measurement Worksheet you will get different types of questions on measurement of length, measurement of weight (mass), measurement of capacity (volume), addition of length, addition of w… -
2nd Grade Fractions Worksheet | Basic Concept of Fractions | Answers
Nov 20, 24 12:16 AM
In 2nd Grade Fractions Worksheet we will solve different types of problems on fractions, one-whole, one-half, one-third, one-fourth, three-fourth or s quarter. In a fraction, it is important that the… -
2nd Grade Math Practice | Second Grade Math |2nd Grade Math Worksheets
Nov 18, 24 02:23 PM
In 2nd grade math practice you will get all types of examples on different topics along with the solutions. Second grade math games are arranged in such a way that students can learn math -
Worksheet on Addition of Length | Word Problems on Addition of Length
Nov 17, 24 10:29 PM
Practice the third grade math worksheet on addition of length. This sheet provides different types of questions where you need to arrange the values of length under different columns to find their sum
New! Comments
Have your say about what you just read! Leave me a comment in the box below. Ask a Question or Answer a Question.