Properties of Multiplication of Fractional Numbers
The properties of multiplication of fractional numbers are discussed here.
Property I: If two fractional numbers are multiplied in either order, the product remains the same.
or, The product of two fractional numbers does not change even if we change the order of multiplication.
Let us understand this property with the help of some examples.
For Example:
1. 23 × 75
= 2×73×5
= 1415
And now if you interchange the place of the fractional numbers the product does not change.
75 × 23
= 7×25×3
= 1415
We observe that the product in both the cases are same.
So, 23 × 75 = 75 × 23.
2. (423 × 513) × 15 = 423 (513 × 15)
3. 13 × 59 = 1×53×9 = 527
59 × 13 = 5×19×3
= 527
4. 8 × 57 = 8×57 = 407 = 557
57 × 8 = 5×87 = 407 = 557
5. 38 × 123 = 38 × 1×3+23 = 38 × 53 = 1524 = 58
123 × 38 = 1×3+23 × 38 = 53 × 38 = 1524 = 58
6. 323 × 4211 = 3×3+23 * 4×11+211 = 113 × 4611 = 463 = 1513
4211 × 323 = 4×11+211 × 3×3+23= 4611 × 113 = 463 = 1513
Property II: The product of three or more fractional numbers does not change even if we change their groups.
Let us understand this with the help of some examples.
1. 13 × (25 × 47) = 13 × (2×45×7) = 13 × 835 = 8105
(13 × 47) × 25 = (1×43×7) × 25 = 421 × 25 = 8105
47 × (13 × 25) = 47 × (1×23×5) = 47 × 215 = 8105
Note: From the above examples we understand that, changing the order of fractional numbers does not change the product.
Property III: If a fractional number is multiplied by one, the product is the fractional number itself.
or, The product of a fractional number and 1 is the fractional number it self.
Let us consider some examples.
For Example:
1. 79 × 1
= 79 × 11
= 7×19×1
= 79
2. 58 × 1
= 58 × 11
= 5×1(8×1
= 58
3. 1519 × 1
= 1519 × 11
= 15×1(19×1
= 1519
4. 2737 × 1 = 27×137 = 2737
5. 434 × 1 = 4×4+34 × 1 = 194 × 1 = 19×14 = 194 = 434
So, we observe that a fraction multiplied by 1 is the fraction itself.
Property IV: If a fractional number is multiplied by zero, the product is zero.
or, The product of a fractional number and 0 is always 0.
Let us consider some examples.
For Example:
1. 311 × 0
= 3×011
= 0
2. 715 × 0
= 7×015
= 0
3. 227 × 0 = 0
4. 234 × 0 = 0
Property V: Multiplicative Inverse Property.
When the product of a fractional number and a whole number is 1, each one is a multiplicative inverse of the other.
Let us consider some examples.
1. 19 × 9 = 1×99 = 99 = 1
Here, 19 the multiplicative inverse of 9 and 9 is the multiplicative inverse of 19
2. 118 × 18 = 1×1818 = 1818 = 1
Here, 118 and 18 are the multiplicative inverse of each other.
Property VI: When the product of two fractional numbers is 1, both are the multiplicative inverse of each other.
Let us consider some examples.
1. 47 × 74 = 2626 = 1
Here, 47 and 74 are multiplicative inverse of each other.
2. 434 × 419 = 4×4+34 × 419 = 194 * 419 19×44×19= 7676 = 1
Here, 434 and 419 are multiplicative inverse of each other.
● Multiplication is Repeated Addition.
● Multiplication of Fractional Number by a Whole Number.
● Multiplication of a Fraction by Fraction.
● Properties of Multiplication of Fractional Numbers.
● Worksheet on Multiplication on Fraction.
● Division of a Fraction by a Whole Number.
● Division of a Fractional Number.
● Division of a Whole Number by a Fraction.
● Properties of Fractional Division.
● Worksheet on Division of Fractions.
● Simplification of Fractions.
● Worksheet on Simplification of Fractions.
● Worksheet on Word Problems on Fractions.
5th Grade Numbers Page
5th Grade Math Problems
From Properties of Multiplication of Fractional Numbers to HOME PAGE
Didn't find what you were looking for? Or want to know more information about Math Only Math. Use this Google Search to find what you need.
Recent Articles
-
Subtraction of Decimals | Subtracting Decimals | Decimal Subtraction
Apr 24, 25 03:25 PM
We will discuss here about the subtraction of decimals. Decimals are subtracted in the same way as we subtract ordinary numbers. We arrange the digits in columns -
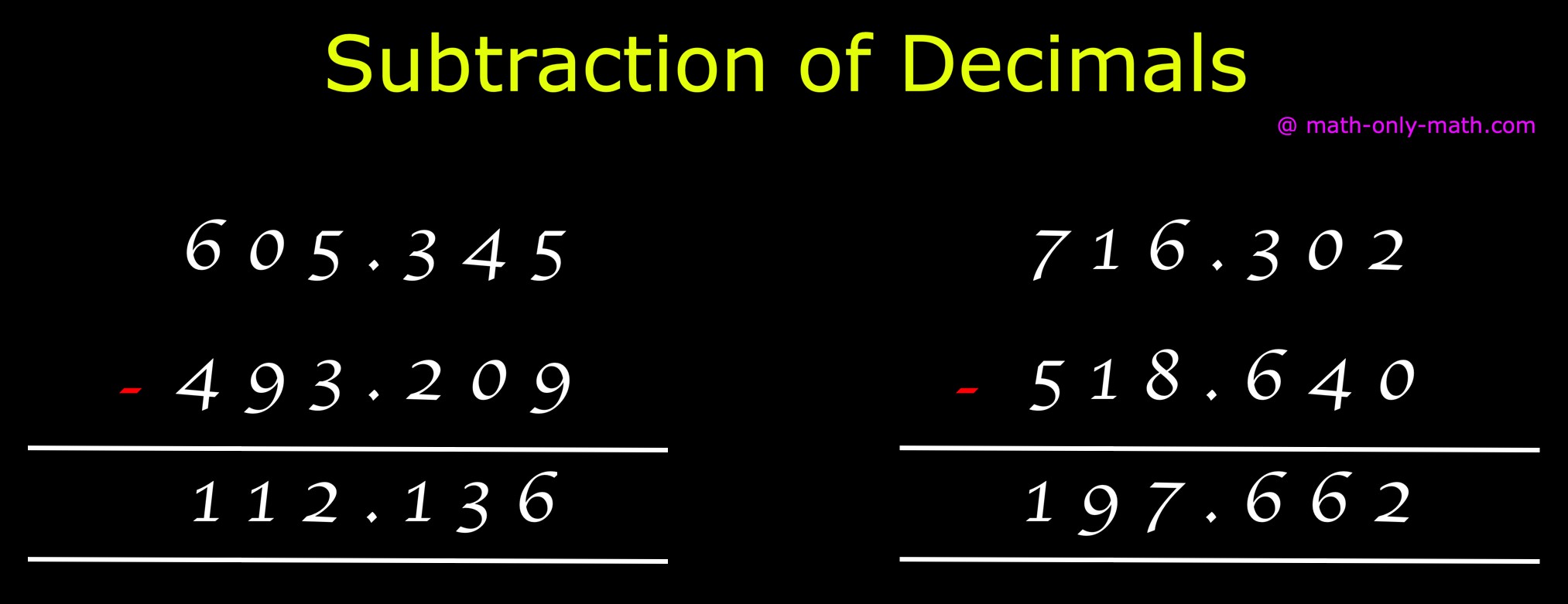
How to Do Long Division? | Method | Steps | Examples | Worksheets |Ans
Apr 24, 25 10:18 AM
As we know that the division is to distribute a given value or quantity into groups having equal values. In long division, values at the individual place (Thousands, Hundreds, Tens, Ones) are dividend… -
Division by Two-Digit Numbers | Knowledge of Estimation | Division
Apr 24, 25 10:12 AM
In division by two-digit numbers we will practice dividing two, three, four and five digits by two-digit numbers. Consider the following examples on division by two-digit numbers: Let us use our knowl… -
Addition of Decimals | How to Add Decimals? | Adding Decimals|Addition
Apr 24, 25 01:45 AM
We will discuss here about the addition of decimals. Decimals are added in the same way as we add ordinary numbers. We arrange the digits in columns and then add as required. Let us consider some -
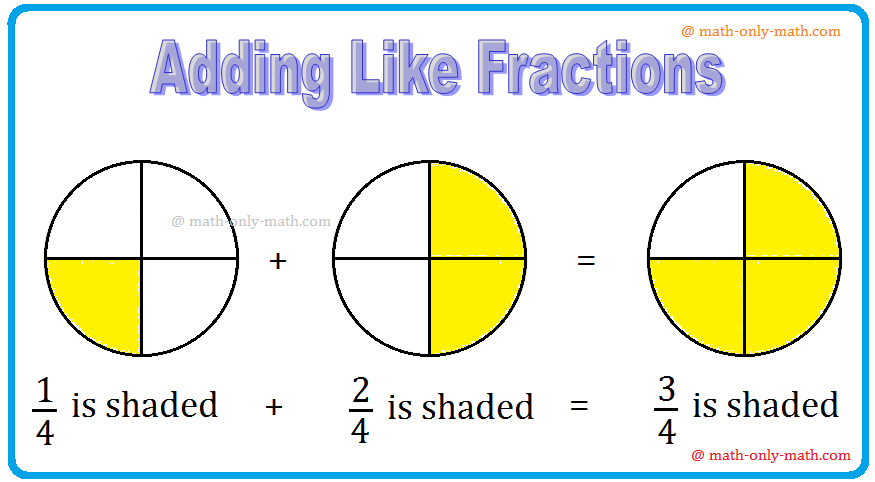
Addition of Like Fractions | Examples | Videos | Worksheet | Fractions
Apr 23, 25 09:23 AM
To add two or more like fractions we simplify add their numerators. The denominator remains same. Thus, to add the fractions with the same denominator, we simply add their numerators and write the com…
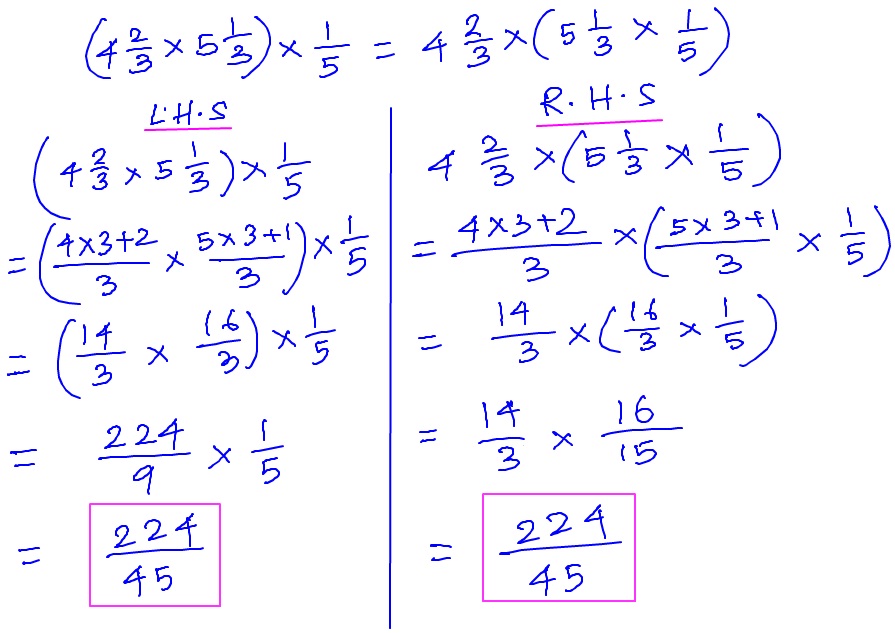



New! Comments
Have your say about what you just read! Leave me a comment in the box below. Ask a Question or Answer a Question.