Parallel and Transversal Lines
Here we discuss how the angles formed between parallel and transversal lines.
When the transversal intersects two parallel lines:
• Pairs of corresponding angles are equal.
• Pairs of alternate angles are equal
• Interior angles on the same side of transversal are supplementary.
Worked-out problems for solving parallel and transversal lines:
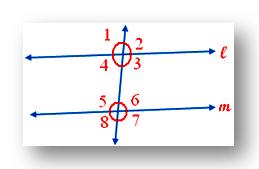
1. In adjoining figure l ∥ m is cut by the transversal t. If ∠1 = 70, find the measure of ∠3, ∠5, ∠6.
Solution:
We have ∠1 = 70°
∠1 = ∠3 (Vertically opposite angles)
Therefore, ∠3 = 70°
Now, ∠1 = ∠5 (Corresponding angles)
Therefore, ∠5 = 70°
Also, ∠3 + ∠6 = 180° (Co-interior angles)
70° + ∠6 = 180°
Therefore, ∠6 = 180° - 70° = 110°
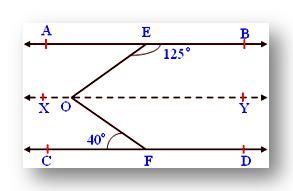
2. In the given figure AB ∥ CD, ∠BEO = 125°, ∠CFO = 40°. Find the measure of ∠EOF.
Solution:
Draw a line XY parallel to AB and CD passing through O such that AB ∥ XY and CD ∥ XY
∠BEO + ∠YOE = 180° (Co-interior angles)
Therefore, 125° + ∠YOE = 180°
Therefore, ∠YOE = 180° - 125° = 55°
Also, ∠CFO = ∠YOF (Alternate angles)
Given ∠CFO = 40°
Therefore, ∠YOF = 40°
Then ∠EOF = ∠EOY + ∠FOY
= 55° + 40° = 95°
3. In the given figure AB ∥ CD ∥ EF and AE ⊥ AB.
Also, ∠BAE = 90°. Find the values of ∠x, ∠y and ∠z.
Solution:
y + 45° = 1800
Therefore, ∠y = 180° - 45° (Co-interior angles)
= 135°
∠y =∠x (Corresponding angles)
Therefore, ∠x = 135°
Also, 90° + ∠z + 45° = 180°
Therefore, 135° + ∠z = 180°
Therefore, ∠z = 180° - 135° = 45°
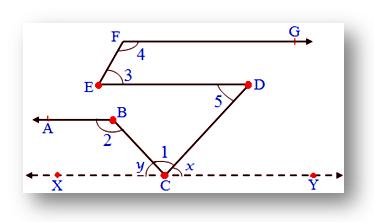
4. In the given figure, AB ∥ ED, ED ∥ FG, EF ∥ CD
Also, ∠1 = 60°, ∠3 = 55°, then find ∠2, ∠4, ∠5.
Solution:
Since, EF ∥ CD cut by transversal ED
Therefore, ∠3 = ∠5 we know, ∠3 = 55°
Therefore, ∠5 = 55°
Also, ED ∥ XY cut by transversal CD
Therefore, ∠5 = ∠x we know ∠5 = 55°
Therefore,∠x = 55°
Also, ∠x + ∠1 + ∠y = 180°
55° + 60° + ∠y = 180°
115° + ∠y = 180°
∠y = 180° - 115°
Therefore, ∠y = 65°
Now, ∠y + ∠2 = 1800 (Co-interior angles)
65° + ∠2 = 180°
∠2 = 180° - 65°
∠2 = 115°
Since, ED ∥ FG cut by transversal EF
Therefore, ∠3 + ∠4 = 180°
55° + ∠4 = 180°
Therefore, ∠4 = 180° - 55° = 125°
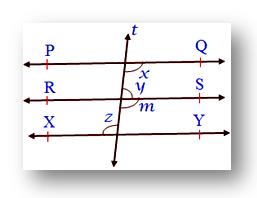
5. In the given figure PQ ∥ XY. Also, y : z = 4 : 5 find.
Solution:
Let the common ratio be a
Then y = 4a and z = 5a
Also, ∠z = ∠m (Alternate interior angles)
Since, z = 5a
Therefore, ∠m = 5a [RS ∥ XY cut by transversal t]
Now, ∠m = ∠x (Corresponding angles)
Since, ∠m = 5a
Therefore, ∠x = 5a [PQ ∥ RS cut by transversal t]
∠x + ∠y = 180° (Co-interior angles)
5a + 4a = 1800
9a = 180°
a = 180/9
a = 20
Since, y = 4a
Therefore, y = 4 × 20
y = 80°
z = 5a
Therefore, z = 5 × 20
z = 100°
x = 5a
Therefore, x = 5 × 20
x = 100°
Therefore, ∠x = 100°, ∠y = 80°, ∠z = 100°
● Lines and Angles
Fundamental Geometrical Concepts
Some Geometric Terms and Results
Complementary and Supplementary Angles
Parallel and Transversal Lines
8th Grade Math Practice
From Parallel and Transversal Lines to HOME PAGE
Didn't find what you were looking for? Or want to know more information about Math Only Math. Use this Google Search to find what you need.
Recent Articles
-
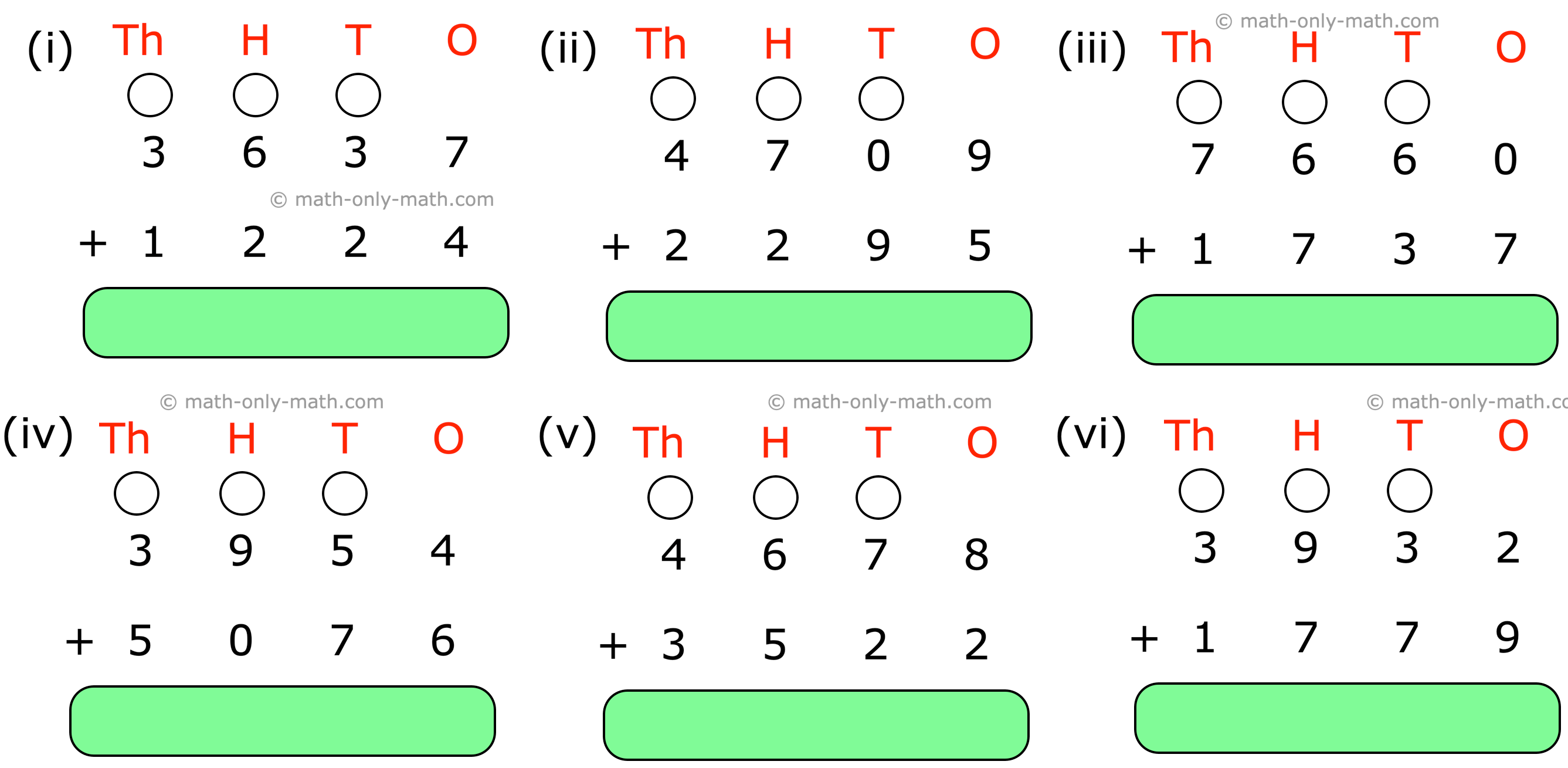
Worksheet on Addition of 4-Digit Numbers | 4 Digit Addition Worksheets
Jan 10, 25 03:02 PM
Practice the questions given in the worksheet on addition of 4-digit numbers. Here we will add two 4-digit numbers (without carrying and with carrying) and three 4-digit numbers -
Word Problems on 4-Digit Numbers |Addition and Subtraction of 4-Digits
Jan 10, 25 02:49 PM
We will solve here some of the word problems on addition and subtraction of 4-digit numbers. We will apply the same method while adding and subtracting the word problems. 1. In a village, there are 25… -
Addition of 10, 100 and 1000 | Adding 10 | Adding 100 | Adding 1000
Jan 10, 25 01:20 AM
Here we will learn Addition of 10, 100 and 1000 with the help of different examples. -
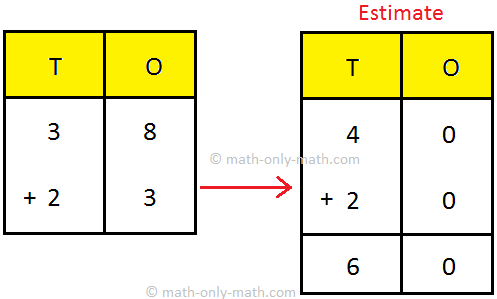
Estimating a Sum | Round the Number | Numbers by Rounding | Estimating
Jan 10, 25 12:10 AM
We will learn the basic knowledge for estimating a sum. Here we will learn an easy way to estimate a sum of two numbers by rounding. In case of two digit numbers we can only round the number -
Addition of 4-Digit Numbers | 4-Digit Addition |Adding 4-Digit Numbers
Jan 09, 25 10:07 PM
We will learn about the addition of 4-digit numbers (without carrying and with carrying). We know how to add 2 or 3, 3-digit numbers without carrying or with carrying.



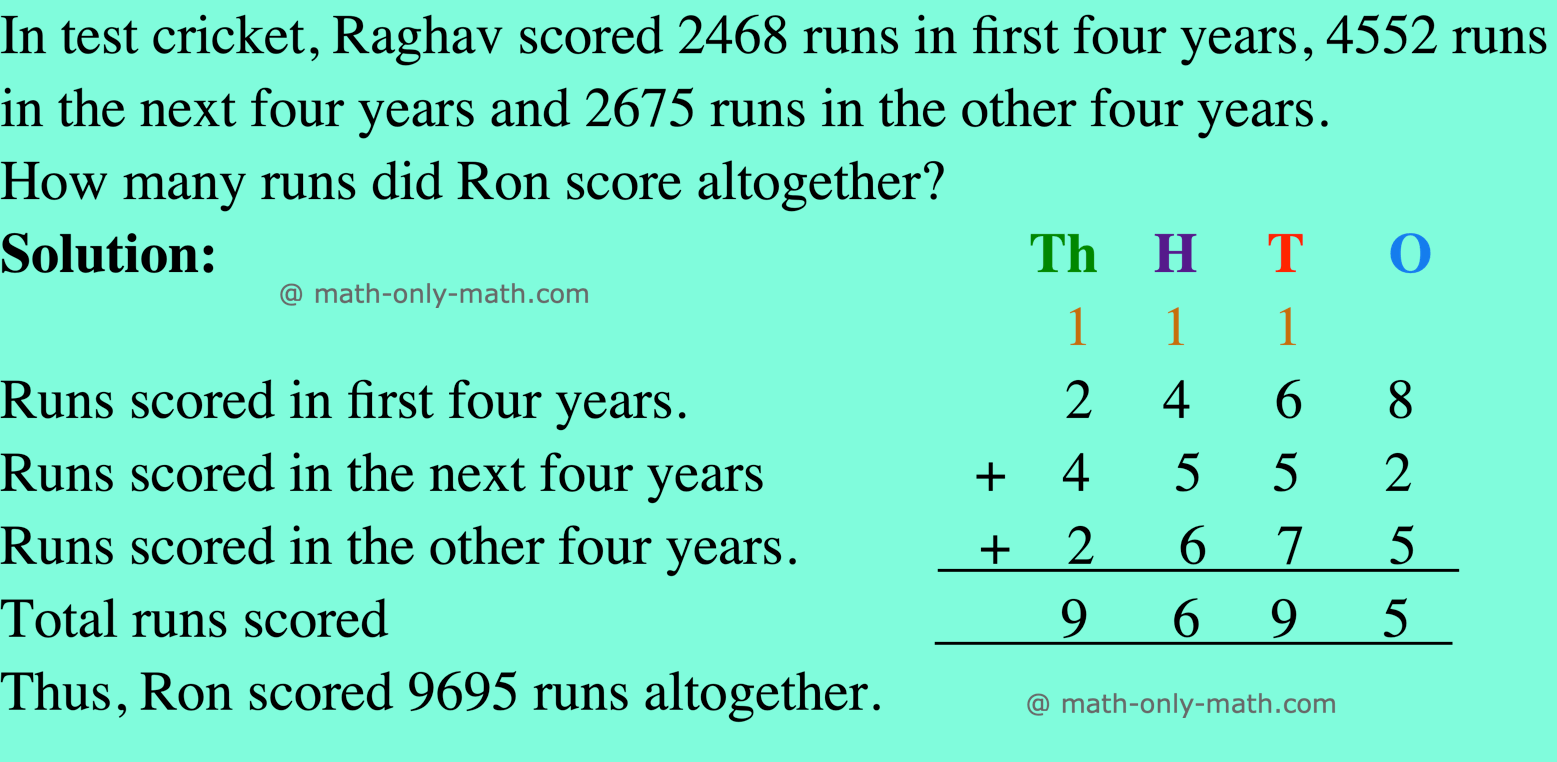


New! Comments
Have your say about what you just read! Leave me a comment in the box below. Ask a Question or Answer a Question.