Parabola whose Vertex at a given Point and Axis is Parallel to y-axis
We will discuss how to find the equation of the parabola whose vertex at a given point and axis is parallel to y-axis.
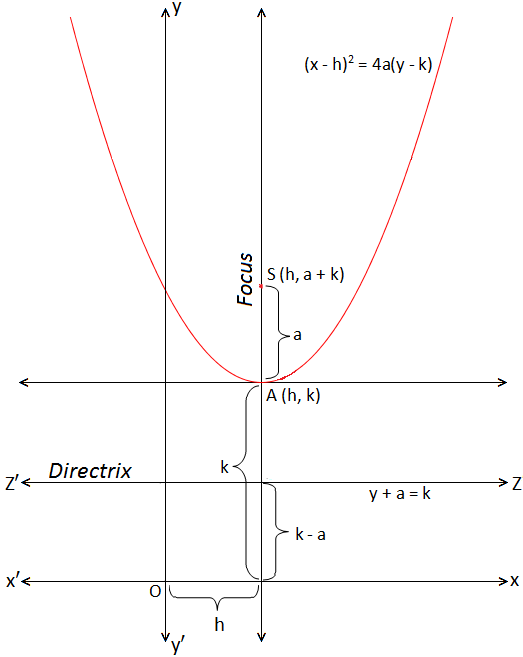
Let A (h, k) be the vertex of the parabola, AM is the axis of the parabola which is parallel to y-axis. The distance between the vertex and focus is AS = a and let P (x, y) be any point on the required parabola.
Now we shift the origin of co-ordinate system at A. Draw two
mutually perpendicular straight lines AM and AN through
the point A as y and x-axes respectively.
According to the new co-ordinate axes (x', y ') be the co-ordinates of P. Therefore, the equation of the parabola is (x’)\(^{2}\) = 4ay' (a > 0) …………….. (i)
Therefore, we get,
AM = y' and PM = x'
Also, OR = k, AR = h, OQ = y, PQ = x
Again, x = PQ
= PM + MQ
= PM + AR
= x' + h
Therefore, x' = x - h
And, y = OQ = OR + RQ
= OR + AM
= k + y'
Therefore, y' = y - k
Now putting the value of x' and y' in (i) we get
(x - h)\(^{2}\) = 4a(y - k), which is the equation of the required parabola.
The equation (x - h)\(^{2}\) = 4a(y - k) represents the equation of a parabola whose co-ordinate of the vertex is at (h, k), the co-ordinates of the focus are (h, a + k), the distance between its vertex and focus is a, the equation of directrix is y - k = - a or, y + a = k, the equation of the axis is x = h, the axis is parallel to positive y-axis, the length of its latus rectum = 4a, co-ordinates of the extremity of the latus rectum are (h + 2a, k + a) and (h - 2a, k + a) and the equation of tangent at the vertex is y = k.
Solved example to find the equation of the parabola with its vertex at a given point and axis is parallel to y-axis:
Find the axis, co-ordinates of vertex and focus, length of latus rectum and the equation of directrix of the parabola x\(^{2}\) - y = 6x - 11.
Solution:
The given parabola x\(^{2}\) - y = 6x - 11.
⇒ x\(^{2}\) - 6x = y - 11.
⇒ x\(^{2}\) - 6x + 9 = y - 11 + 9
⇒ (x - 3)\(^{2}\) = y - 2
⇒ (x - 3)\(^{2}\) = 4 ∙ ¼(y - 2) ………….. (i)
Compare the above equation (i) with standard form of parabola (x - h)\(^{2}\) = 4a(y - k), we get, h = 3, k = 2 and a = ¼.
Therefore, the axis of the given parabola is along parallel to positive y-axis and its equation is x = h i.e., x = 3 i.e., x - 3 = 0.
The co-ordinates of its vertex are (h, k) i.e., (3, 2).
The co-ordinates of its focus are (h, a + k) i.e., (3, ¼ + 2) i.e., (3, \(\frac{9}{4}\)).
The length of its latus rectum = 4a = 4 ∙ ¼ = 1 unit
The equation of its directrix is y + a = k i.e., y + ¼ = 2 i.e., y + ¼ - 2 = 0 i.e., y - \(\frac{7}{4}\) = 0 i.e., 4y - 7 = 0.
● The Parabola
- Concept of Parabola
- Standard Equation of a Parabola
- Standard form of Parabola y22 = - 4ax
- Standard form of Parabola x22 = 4ay
- Standard form of Parabola x22 = -4ay
- Parabola whose Vertex at a given Point and Axis is Parallel to x-axis
- Parabola whose Vertex at a given Point and Axis is Parallel to y-axis
- Position of a Point with respect to a Parabola
- Parametric Equations of a Parabola
- Parabola Formulae
- Problems on Parabola
11 and 12 Grade Math
From Parabola whose Vertex at a given Point and Axis is Parallel to y-axis to HOME PAGE
Didn't find what you were looking for? Or want to know more information about Math Only Math. Use this Google Search to find what you need.
Recent Articles
-
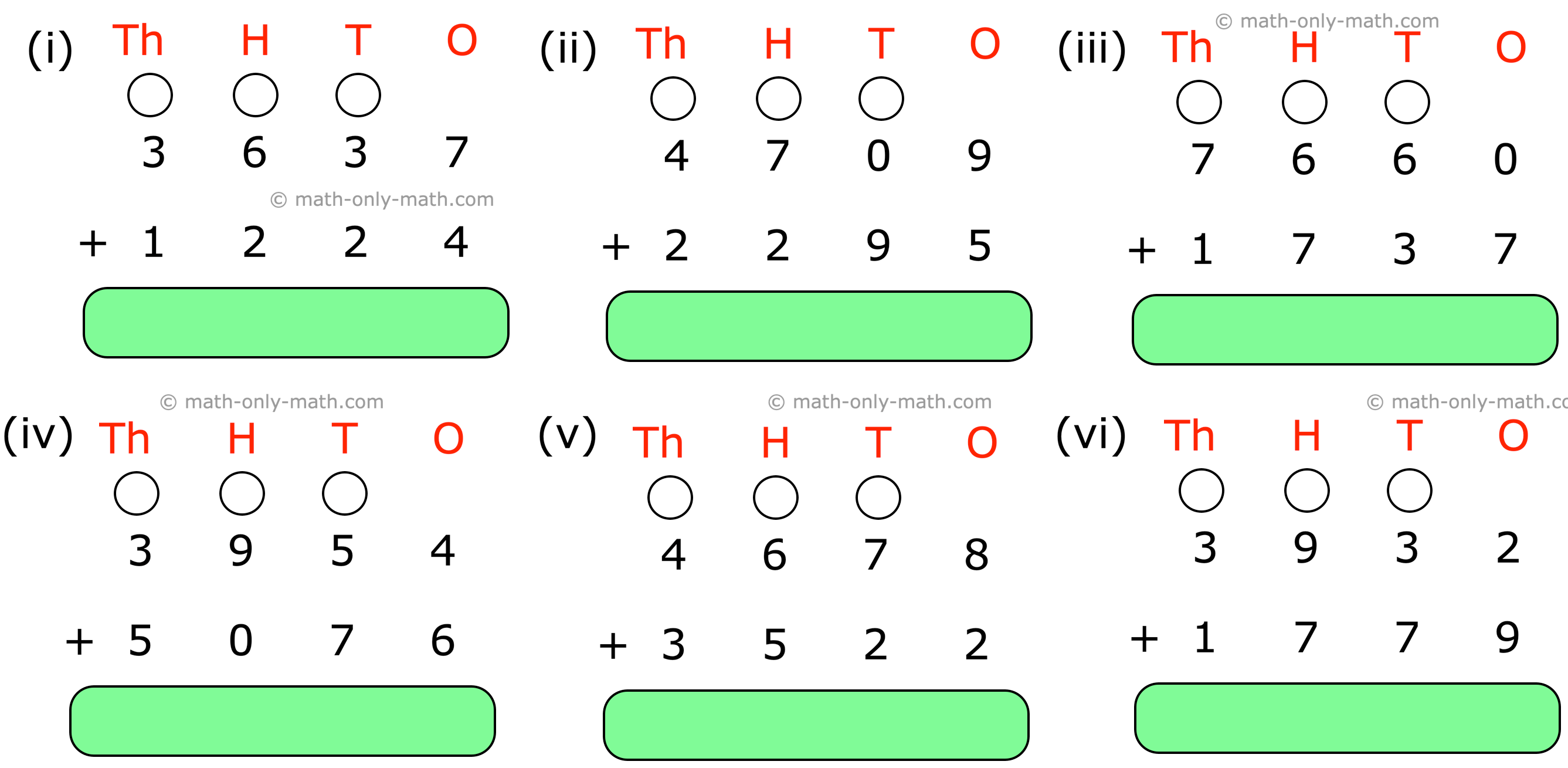
Addition of 4-Digit Numbers | 4-Digit Addition |Adding 4-Digit Numbers
Jan 11, 25 02:56 AM
We will learn about the addition of 4-digit numbers (without carrying and with carrying). We know how to add 2 or 3, 3-digit numbers without carrying or with carrying. -
Worksheet on Addition of 4-Digit Numbers | 4 Digit Addition Worksheets
Jan 11, 25 02:48 AM
Practice the questions given in the worksheet on addition of 4-digit numbers. Here we will add two 4-digit numbers (without carrying and with carrying) and three 4-digit numbers -
Word Problems on 4-Digit Numbers |Addition and Subtraction of 4-Digits
Jan 10, 25 02:49 PM
We will solve here some of the word problems on addition and subtraction of 4-digit numbers. We will apply the same method while adding and subtracting the word problems. 1. In a village, there are 25… -
Addition of 10, 100 and 1000 | Adding 10 | Adding 100 | Adding 1000
Jan 10, 25 01:20 AM
Here we will learn Addition of 10, 100 and 1000 with the help of different examples. -
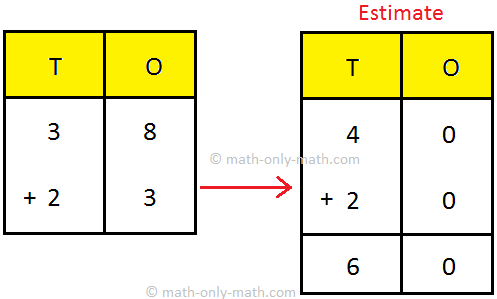
Estimating a Sum | Round the Number | Numbers by Rounding | Estimating
Jan 10, 25 12:10 AM
We will learn the basic knowledge for estimating a sum. Here we will learn an easy way to estimate a sum of two numbers by rounding. In case of two digit numbers we can only round the number


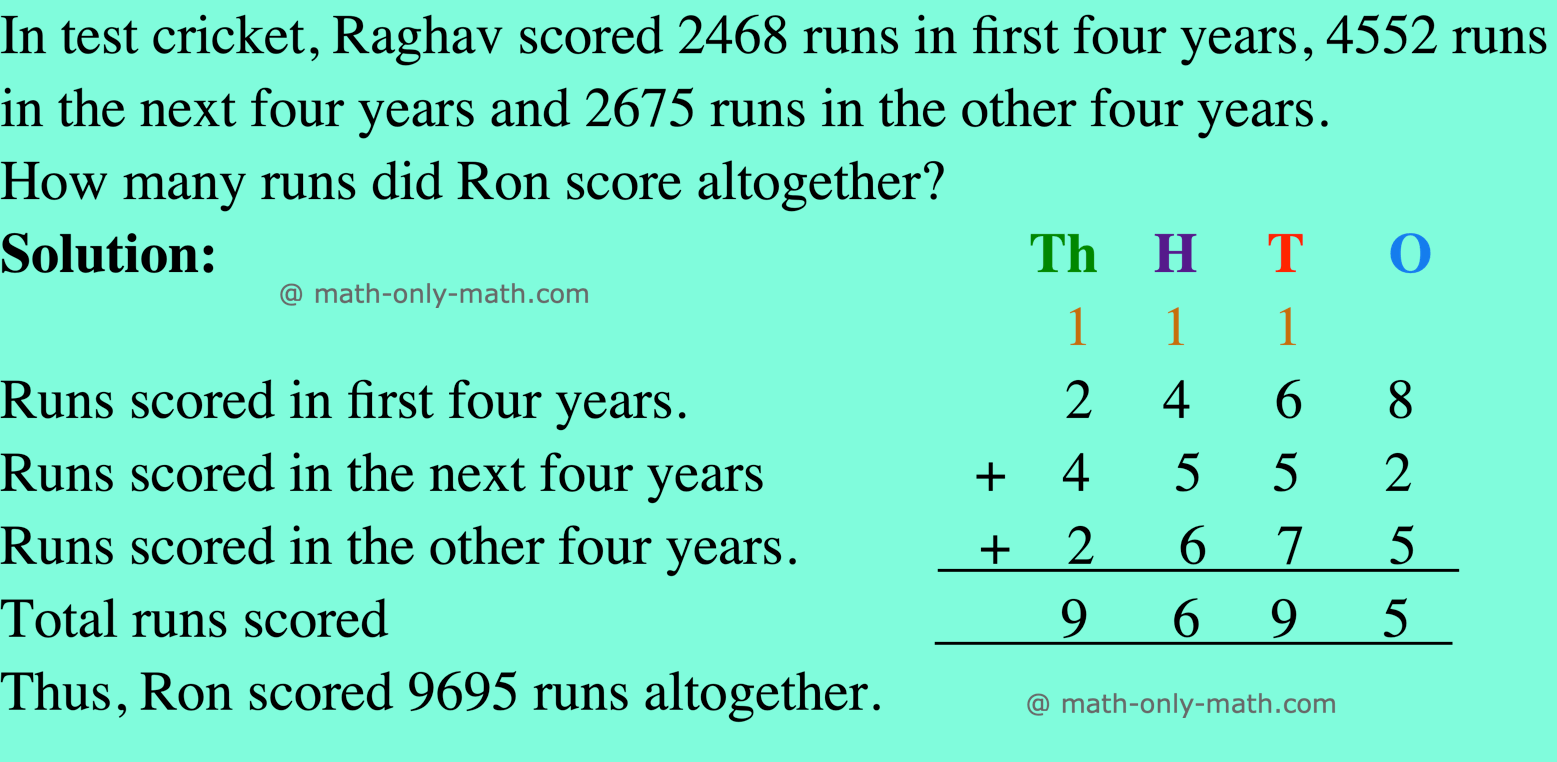

New! Comments
Have your say about what you just read! Leave me a comment in the box below. Ask a Question or Answer a Question.