Multiplication of Matrices
Two matrices A and B are said to be conformable for the product AB if the number of columns of A be equal to the number of rows of B.
If A be an m × n matrix and B an n × p matrix then their product AB is defined to be the m × p matrix whose (ij)th element is obtained by multiplying the elements of the ijth row of A into the corresponding elements of the jth column of B and summing the products so obtained.
In other words, if A = (aij) m, n, B = (bij) n, p then the product AB is a matrix of order m × p and AB = C = (cij) m, p where (cij) = ∑nk=1aikbkj, i = 1, 2, 3, ...., m; j = 1, 2, 3, ...., p.
The ijth element of the product AB is obtained by multiplying the corresponding elements of the ithrow of A and jth column of B and adding the products. This sum is called the inner product of the ith row of A and jth column of B. So the (ij)th element of the product AB is the inner product of the ith row of A and the jth column of B.
If the number of columns of A be not equal to number of rows of B, then AB is not defined.
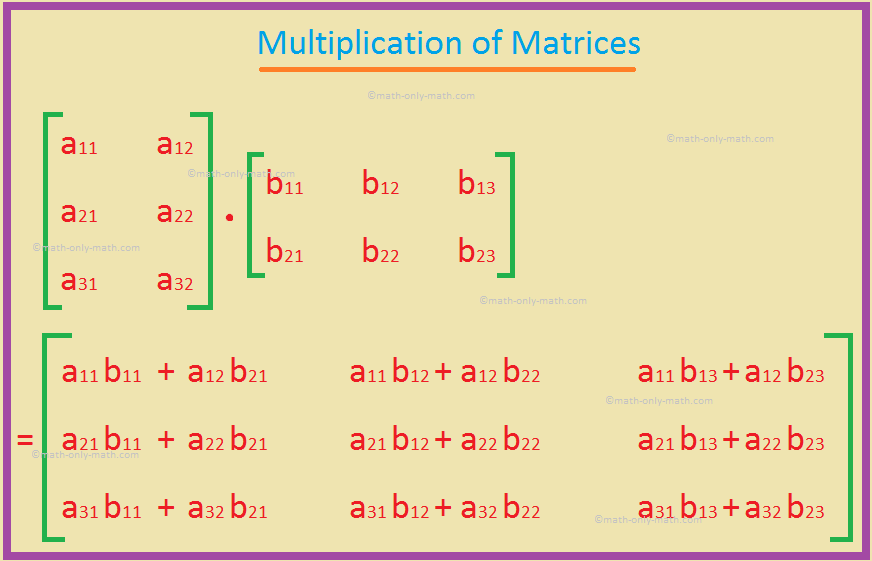
Let A = [a11a12a21a22a31a32] and B = [b11b12b13b21b22b23]
Here we can see that the matrix A has two columns and the matrix B has two rows. Therefore, A and B are conformable for the product AB.
Thus the product of the matrix A and the matrix B = AB = [a11a12a21a22a31a32] . [b11b12b13b21b22b23]
= [a11b11+a12b21a11b12+a12b22a11b13+a12b23a21b11+a22b21a21b12+a22b22a21b13+a22b23a31b11+a32b21a31b12+a32b22a31b13+a32b23]
In the product AB, A is said to be a pre-factor or pre-multiplier
and B is said to be a post factor or post-multiplier.
It is obvious that the products AB and BA are two distinct entities. Indeed, one of them may exist while the other may not. In order that both AB and BA should exist, if A be the order of m × n, B must be of order n × m. In this case, however, AB and BA are matrix of different orders. In order that both AB and BA should exist as matrices of the same order, both A and B must be square matrices of the same order.
Note: Matrix multiplication is not commutative. That is, for two matrices A and B, AB ≠ BA, in general.
First of all, if we choose the orders of A and B to be m × n and n × m respectively so that the conformability conditions for both the products AB and BA are satisfied then we observe that the orders of AB and BA are m × m and n × n respectively and therefore AB cannot be equal to BA.
In order that AB and BA may be equal, both of them must be of the same order and this requires that A, B must be square matrices of the same order. However if we choose the order of A and B to be n × n and n × n, then although AB and BA become matrices of the same order, they may not be equal, in general. This can be shown by taking at random.
A = [1520], B = [3−146]
Here AB = [23296−2], BA = [1151620].
In some special cases, however, AB = BA.
For example, let A = [1223], B = [1001].
Here AB = [1223], BA = [1223].
Definition: Two matrices A and B are said to commute with each other if AB = BA. Since AB = BA, A and B must be square matrices of the same order.
Examples of Commuting Matrices:
1. Let A be a square matrix. Then A commutes with A itself.
2. Let A be a square matrix of order n. Then A commutes with In, because A . In = In . A = A.
3. Let A be a square matrix of order n. Then A commutes with On,n, because A. On,n = On,n . A = On,n.
4. Let A be square matrix of order n. Then A commutes with the scalar matrix cIn, because A . cIn . A = cIn . A = cA.
From Multiplication of Matrices to HOME
Didn't find what you were looking for? Or want to know more information about Math Only Math. Use this Google Search to find what you need.
Recent Articles
-
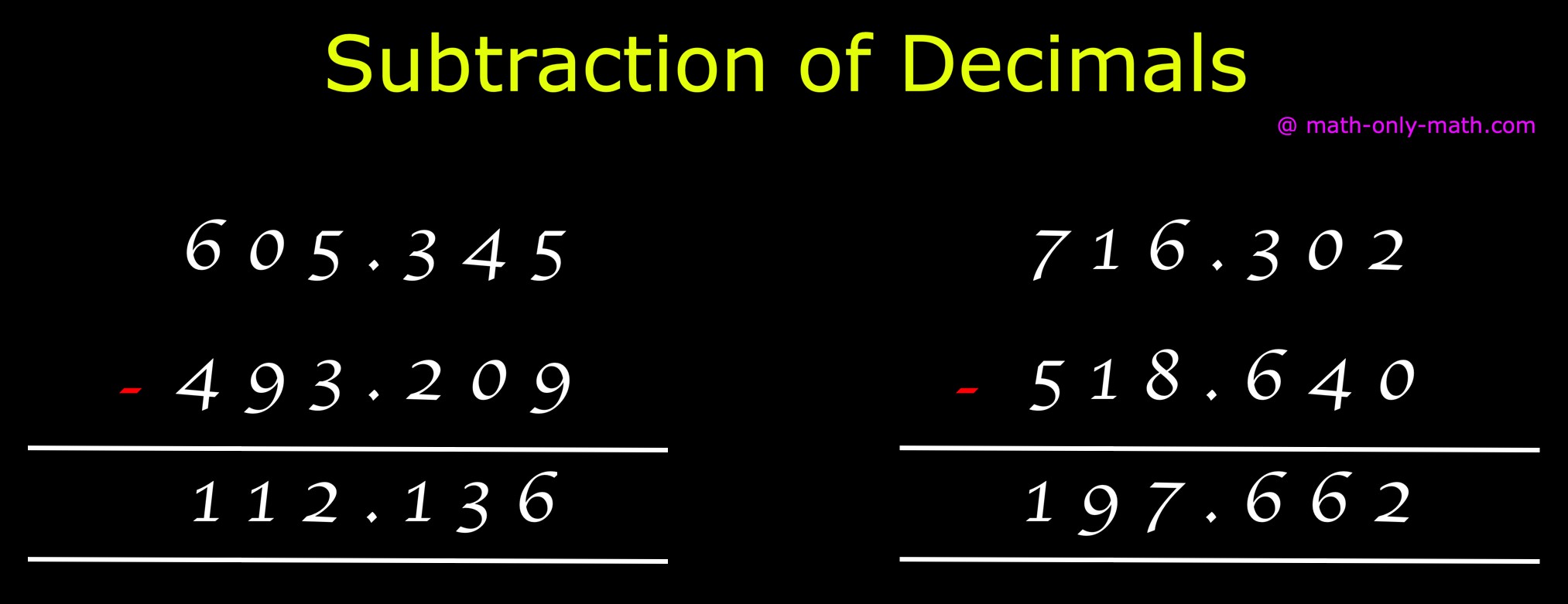
Subtraction of Decimals | Subtracting Decimals | Decimal Subtraction
Apr 24, 25 03:25 PM
We will discuss here about the subtraction of decimals. Decimals are subtracted in the same way as we subtract ordinary numbers. We arrange the digits in columns -
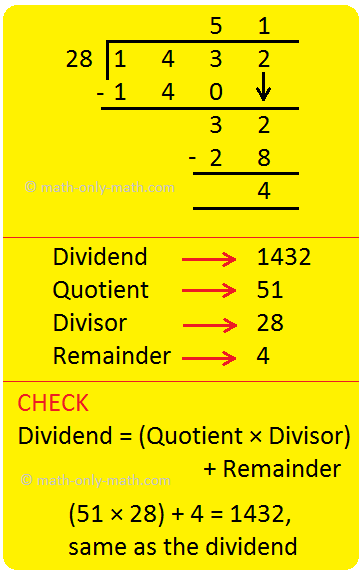
How to Do Long Division? | Method | Steps | Examples | Worksheets |Ans
Apr 24, 25 10:18 AM
As we know that the division is to distribute a given value or quantity into groups having equal values. In long division, values at the individual place (Thousands, Hundreds, Tens, Ones) are dividend… -
Division by Two-Digit Numbers | Knowledge of Estimation | Division
Apr 24, 25 10:12 AM
In division by two-digit numbers we will practice dividing two, three, four and five digits by two-digit numbers. Consider the following examples on division by two-digit numbers: Let us use our knowl… -
Addition of Decimals | How to Add Decimals? | Adding Decimals|Addition
Apr 24, 25 01:45 AM
We will discuss here about the addition of decimals. Decimals are added in the same way as we add ordinary numbers. We arrange the digits in columns and then add as required. Let us consider some -
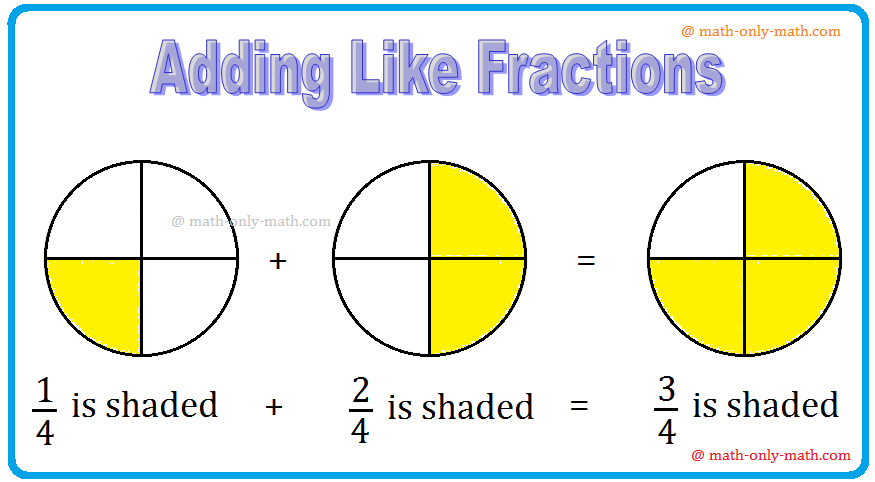
Addition of Like Fractions | Examples | Videos | Worksheet | Fractions
Apr 23, 25 09:23 AM
To add two or more like fractions we simplify add their numerators. The denominator remains same. Thus, to add the fractions with the same denominator, we simply add their numerators and write the com…



New! Comments
Have your say about what you just read! Leave me a comment in the box below. Ask a Question or Answer a Question.