Measure of the Angles of the Cyclic Quadrilateral
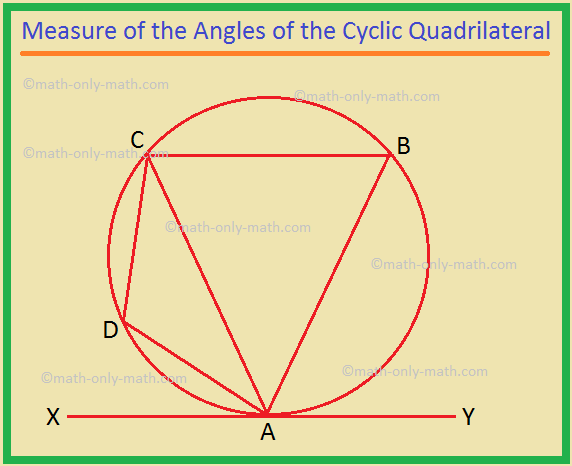
We will prove that, in the figure ABCD is a cyclic quadrilateral and the tangent to the circle at A is the line XY. If ∠CAY : ∠CAX = 2 : 1 and AD bisects the angle CAX while AB bisects ∠CAY then find the measure of the angles of the cyclic quadrilateral. Also, prove that DB is a diameter of the circle.
Solution:
∠CAY + ∠CAX = 180° and ∠CAY : ∠CAX = 2 : 1.
Therefore, ∠CAY = 23 × 180° = 120° and ∠CAX = 13 × 180° = 60°.
As AD bisects ∠CAX, ∠DAX = ∠CAD = 12 × 60° = 30°
As AB bisects ∠CAY, ∠YAB = ∠CAB = 12 × 120° = 60°.
Now, ∠CAY = ∠ADC = 120° (Since, angle between tangent and chord is equal to the angle in the alternate segment).
Therefore, ∠CBA = 180° - ∠ADC = 180° - 120° = 60° (Since opposite angles of a cyclic quadrilateral are supplementary).
Again, ∠DAB = ∠DAC + ∠CAB = 30° + 60° = 90°.
Therefore, ∠BCD = 180° - ∠DAB = 180° - 90° = 90°.
We can see that the chord DB subtends a right angle at A.
Therefore, DB is a diameter of the circle (as an angle in a semicircle is a right-angle).
From Measure of the Angles of the Cyclic Quadrilateral to HOME PAGE
Didn't find what you were looking for? Or want to know more information about Math Only Math. Use this Google Search to find what you need.
Recent Articles
-
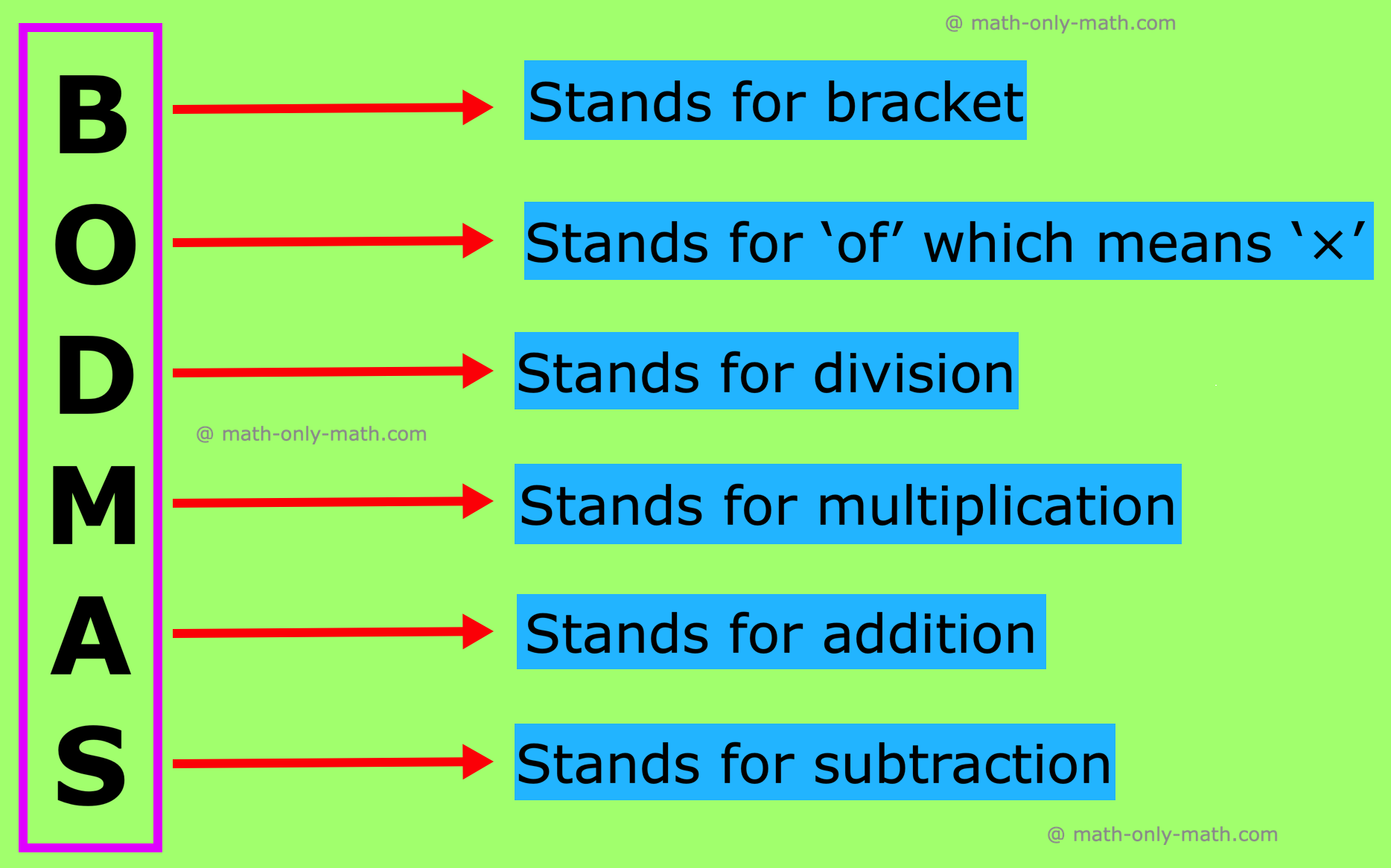
BODMAS Rule | Order of Operation | Definition, Examples, Problems
Mar 28, 25 02:17 AM
Easy and simple way to remember BODMAS rule!! B → Brackets first (parentheses) O → Of (orders i.e. Powers and Square Roots, Cube Roots, etc.) DM → Division and Multiplication -
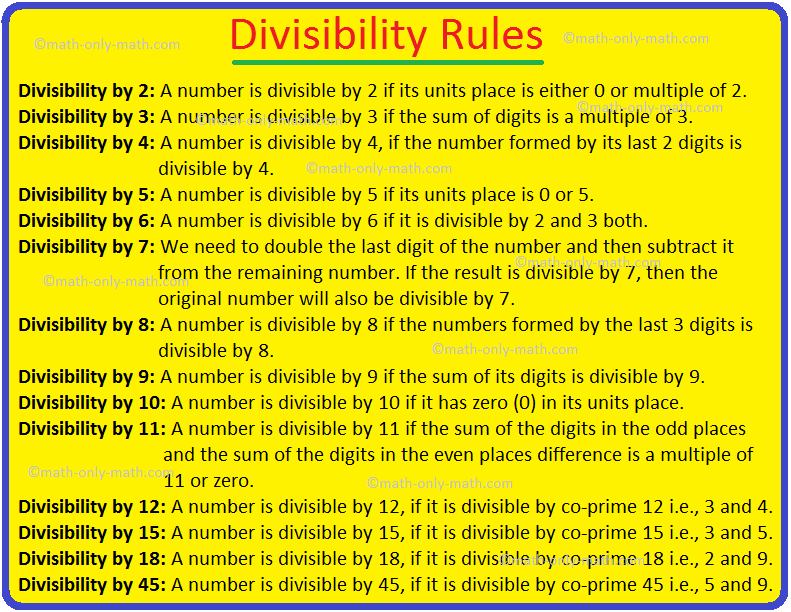
Divisibility Rules From 2 to 18 | Math Divisibility Test | Videos |
Mar 27, 25 01:04 PM
To find out factors of larger numbers quickly, we perform divisibility test. There are certain rules to check divisibility of numbers. Divisibility tests of a given number by any of the number 2, 3, 4… -
Divisible by 2 Video |Test of Divisibility by 2 Trick| Rules| Examples
Mar 27, 25 11:10 AM
A number is divisible by 2 if the digit at unit place is either 0 or multiple of 2. So a number is divisible by 2 if digit at its units place is 0, 2, 4, 6 or 8. -
Divisible by 3 | Test of Divisibility by 3 Trick | Rules | Video
Mar 27, 25 11:01 AM
A number is divisible by 3, if the sum of its all digits is a multiple of 3 or divisibility by 3. Consider the following numbers to find whether the numbers are divisible or not divisible by 3: (i) 54… -
Divisible by 4 | Test of Divisibility by 4 Trick | Rules | Video
Mar 27, 25 11:00 AM
A number is divisible by 4 if the number is formed by its digits in ten’s place and unit’s place (i.e. the last two digits on its extreme right side) is divisible by 4. Consider the following numbers…




New! Comments
Have your say about what you just read! Leave me a comment in the box below. Ask a Question or Answer a Question.