Intercepts on the Axes made by a Circle
We will learn how to find the intercepts on the axes made by a circle.
The lengths of intercepts made by the circle x2 + y2 + 2gx + 2fy + c = 0 with X and Y axes are 2√g2−c and 2√f2−c respectively.
Proof:
Let the given equation of the circle be x2 + y2 + 2gx + 2fy + c = 0 ………. (1)
Clearly, the centre of the circle is c (-g, -f) and the radius = √g2+f2−c
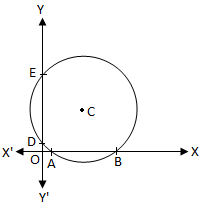
Let AB be the intercept made by the given circle on x-axe.
Since on x-axis, y = 0. Therefore, x-coordinates of the points A and B are the
roots of the equation x2 + 2gx + c = 0.
Let x1 and x2 be the x-coordinates of the points A and B respectively. Then, x1 and x2 also the roots of the equation x2 + 2gx + c = 0.
Therefore, x1 + x2 = - 2g and x1x2 = c
Clearly the intercept on x-axis = AB
= x2 - x1 = √(x2−x1)2
= √(x2+x1)2−4x1x2
= √4g2−4c
= 2√g2−c
Therefore, the intercept made by the circle (1) on the x-axis = 2√g2−c
Again,
Let DE be the intercept made by the given circle on y-axe. Since on y-axis, x = 0. Therefore, y-coordinates of the points D and E are the roots of the equation y2 + 2fy + c = 0.
Let y1 and y2 be the x-coordinates of the points D and E respectively. Then, y1 and y2 also the roots of the equation y2 + 2fy + c = 0
Therefore, y1 + y2 = - 2f and y1y2 = c
Clearly the intercept on y-axis = DE
= y2 - y1 = √(y2−y1)2
= √(y2+y1)2–4y1y2
= √4f2−4c
= 2√f2−c
Therefore, the intercept made by the circle (1) on the y-axis = 2√f2−c
Solved examples to find the intercepts made by a given circle on the co-ordinate axes:
1. Find the length of the x-intercept and y-intercept made by the circle x2 + y2 - 4x -6y - 5 = 0 with the co-ordinate axes.
Solution:
Given equation of the circle is x2 + y2 - 4x -6y - 5 = 0.
Now comparing the given equation with the general equation of the circle x2 + y2 + 2gx + 2fy + c = 0, we get g = -2 and f = -3 and c = -5
Therefore, length of the x-intercept = 2√g2−c = 2√4−(−5) = 2√9 = 6.
The length of the y-intercept = 2√f2−c = 2√9−(−5) = 2√14.
2. Find the equation of a circle which touches the y-axis at a distance -3 from the origin and cuts an intercept of 8 units with the positive direction of x-axis.
Solution:
Let the equation of the circle be x2 + y2 + 2gx + 2fy + c = 0 …………….. (i)
According to the problem, the equation (i) touches the y-axis
Therefore, c = f2 ………………… (ii)
Again, the point (0, -3) lies on the circle (i).
Therefore, putting the value of x = 0 and y = -3 in (i) we get,
9 - 6f + c = 0 …………………… (iii)
From (ii) and (iii), we get 9 - 6f + f2 = 0 ⇒ (f - 3)2 = 0 ⇒ f - 3 = 0 ⇒ f = 3
Now putting f = 3 in (i) we get, c = 9
Again, according to the problem the equation of the circle (i) cuts an intercept of 8 units with the positive direction of x-axis.
Therefore,
2√g2−c = 8
⇒ 2√g2−9 = 8
⇒ √g2−9 = 4
⇒ g2 - 9 = 16, [Squaring both sides]
⇒ g2 = 16 + 9
⇒ g2 = 25
⇒ g = ±5.
Hence, the required equation of the circle is x^2 + y^2 ± 10x + 6y + 9 = 0.
● The Circle
- Definition of Circle
- Equation of a Circle
- General Form of the Equation of a Circle
- General Equation of Second Degree Represents a Circle
- Centre of the Circle Coincides with the Origin
- Circle Passes through the Origin
- Circle Touches x-axis
- Circle Touches y-axis
- Circle Touches both x-axis and y-axis
- Centre of the Circle on x-axis
- Centre of the Circle on y-axis
- Circle Passes through the Origin and Centre Lies on x-axis
- Circle Passes through the Origin and Centre Lies on y-axis
- Equation of a Circle when Line Segment Joining Two Given Points is a Diameter
- Equations of Concentric Circles
- Circle Passing Through Three Given Points
- Circle Through the Intersection of Two Circles
- Equation of the Common Chord of Two Circles
- Position of a Point with Respect to a Circle
- Intercepts on the Axes made by a Circle
- Circle Formulae
- Problems on Circle
11 and 12 Grade Math
From Intercepts on the Axes made by a Circle to HOME PAGE
Didn't find what you were looking for? Or want to know more information about Math Only Math. Use this Google Search to find what you need.
Recent Articles
-
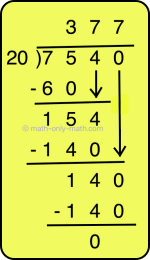
How to Do Long Division? | Method | Steps | Examples | Worksheets |Ans
Apr 20, 25 11:46 AM
As we know that the division is to distribute a given value or quantity into groups having equal values. In long division, values at the individual place (Thousands, Hundreds, Tens, Ones) are dividend… -
Word Problems on Division | Examples on Word Problems on Division
Apr 20, 25 11:17 AM
Word problems on division for fourth grade students are solved here step by step. Consider the following examples on word problems involving division: 1. $5,876 are distributed equally among 26 men. H… -
Subtraction of 4-Digit Numbers | Subtract Numbers with Four Digit
Apr 20, 25 10:27 AM
We will learn about the subtraction of 4-digit numbers (without borrowing and with borrowing). We know when one number is subtracted from another number the result obtained is called the difference. -
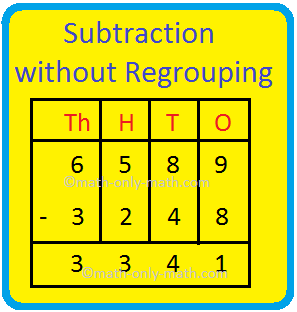
Subtraction without Regrouping |4-Digit, 5-Digit & 6-Digit Subtraction
Apr 20, 25 10:25 AM
We will learn subtracting 4-digit, 5-digit and 6-digit numbers without regrouping. We first arrange the numbers one below the other in place value columns and then subtract the digits under each colum… -
Worksheets on Missing Numbers from 1 to 20 | Counting Missing Numbers
Apr 20, 25 10:17 AM
Printable worksheets on missing numbers from 1 to 20 help the kids to practice counting of the numbers.



New! Comments
Have your say about what you just read! Leave me a comment in the box below. Ask a Question or Answer a Question.