Induction Proof
In math induction proof we will work on some examples using mathematical induction.
Induction proof is a mathematical method of proving a set of formula or theory or series of natural numbers. Induction proof is used from the theory of mathematical induction which is similar to the incident of fall of dominoes. When we push a domino in a set of dominoes the falling of first domino leads to the falling of other dominoes. So if there is a set of infinite dominoes then a falling effect of single domino will lead to the falling of other dominoes one by one. Now to prove this incident we can say the first domino falls to second and the second will fall to third and this way others will fall. Similarly in induction proof for infinite series of n numbers set where P (n) is the set property, we do not need to prove the property for all natural numbers. We prove it for two instances. First is for P (0) or P (1) and this step is called base step. In the second step we need to assume first that the property P (k) is true which is called induction hypothesis. By using inductive hypothesis we need to prove the P (k+1) is true and this step is called inductive step. This is how in induction proof for property P (n), we need to prove it for P (0) or P (1) and P (k+1) and then it can be stated that the property statement is true or holds for all the natural numbers.
Now we will try to understand induction proof from an example. First we take a property of sum of n natural numbers.
1 + 2 + 3 + ……. + n = \(\frac{n(n + 1)}{2}\)
The above set of natural numbers is property P (n) which is simply a formula of sum of n natural numbers. By using induction proof technique we need to prove that this formula holds true for all natural numbers. As stated before the first step is base step P (1).
For P (1),
LHS = 1
RHS = \(\frac{1(1 + 1)}{2}\) = 1.
So, LHS = RHS.
It is proved that P (1) is true.
Now in second step by using induction hypothesis of mathematical induction we assume P (k) is true.
1 + 2 + 3 + ……. + k = \(\frac{k(k + 1)}{2}\)
We need to prove P(k + 1) is true by using P (k) true.
For P(k + 1),
LHS = 1 + 2 + 3 + ……. + k + (k + 1)
= \(\frac{k(k + 1)}{2}\) + (k+1) ………by using induction hypothesis
= \(\frac{(k + 1)(k + 2)}{2}\)
= \(\frac{(k + 1)((k + 1) + 1}{2}\) = RHS for P(k + 1)
P(k + 1) is true, whenever P(k) is true.
Thus P(1) is true and P(k + 1) is true whenever p(k) is true.
Hence, by the principle of mathematical induction, P(n) is true for all n ∈ N.
Mathematical Induction - Problems with Solutions (induction proof):
1. Using the principle of mathematical induction, prove that n(n + 1)(n + 5) is a multiple of 3 for all n ∈ N.
Solution:
Let P(n): n(n + 1)(n + 5) is a multiple of 3.
For n = 1, the given expression becomes (1 × 2 × 6) = 12, which is a multiple of 3.
So, the given statement is true for n = 1, i.e. P(1) is true.
Let P(k) be true. Then,
P(k): k(k + 1)(k + 5) is a multiple of 3
⇒ K(k + 1)(k + 5) = 3m for some natural number m, ... (i)
Now, (k + 1l)(k + 2)(k + 6) = (k + 1)(k + 2)k + 6(k + 1)(k + 2)
= k(k + 1)(k + 2) + 6(k + 1)(k + 2)
= k(k + 1)(k + 5 – 3) + 6(k + 1)(k + 2)
= k(k + 1)(k + 5) – 3k(k + 1) + 6(k + 1)(k + 2)
= k(k + 1)(k + 5) + 3(k + 1)(k +4) [on simplification]
= 3m + 3(k + 1 )(k + 4) [using (i)]
= 3[m + (k + 1)(k + 4)], which is a multiple of 3
⇒ P(k + 1): (k + 1 )(k + 2)(k + 6) is a multiple of 3
⇒ P(k + 1) is true, whenever P(k) is true.
Thus, P(1) is true and P(k + 1) is true, whenever P(k) is true.
Hence, by the principle of mathematical induction, P(n) is true for all n ∈ N.
Induction Proof
2. Using the principle of mathematical induction, prove that (n² + n) is even for all n ∈ N.
Solution:
Let P(n): (n² + n) is even.
For n = 1, the given expression becomes (1² + 1) = 2, which is even.
So, the given statement is true for n = 1, i.e., P(1)is true.
Let P(k) be true. Then,
P(k): (k² + k) is even
⇒ (k² + k) = 2m for some natural number m. ... (i)
Now, (k + 1)² + (k + 1) = k² + 3k + 2
= (k² + k) + 2(k + 1)
= 2m + 2(k + 1) [using (i)]
= 2[m + (k + 1)], which is clearly even.
Therefore, P(k + 1): (k + 1)² + (k + 1) is even
⇒ P(k + 1) is true, whenever P(k) is true.
Thus, P(1) is true and P(k + 1) is true, whenever P(k) is true.
Hence, by the principle of mathematical induction, P(n)is true for all n ∈ N.
Induction Proof
More Examples on math Induction Proof
3. Using the principle of mathematical induction, prove that(1 + x)n ≥ (1 + nx)for all n ∈ N,where x > - 1.
Solution:
Let P(n): (1 + x) )n ≥ (1 + nx).
For n = 1, we have LHS = (1 + x) )1 = (1 + x),and
RHS = (1 + 1 ∙ x) = (1 + x).
Therefore LHS ≥ RHS is true.
Thus, P(1) is true.
Let P(k) is true. Then,
P(k): (1 + X)k ≥ (1 + kx). …….. (i)
Now,(1 + x)k + 1 = (1 + x)k (1 + x)
≥ (1 + kx)(1 + x) [using (i)]
=1 + (k + 1)x + kx2
≥ 1 + (k + 1)x + x [Since kx2 ≥ 0]
Therefore P(k + 1): (1 + x)k + 1 ≥ 1 + (k + 1)x
⇒ P(k +1) is true, whenever P(k) is true.
Thus, P(1) is true and P(k + 1) is true, whenever P(k) is true. Hence, by the principle of mathematical induction, P(n) is true for all n ∈ N.
Induction Proof
4. Using the principle of mathematical induction, prove that n < 2n for all n ∈ N.
Solution:
Let P(n): n <2n.
When n = 1, LHS = 1 and RHS = 21 = 2.
Clearly, 1 < 2.
Thus, P(n) is true for n = 1, i.e., P(1) is true.
Let P(1) be true. Then,
P(k): k < 2k.
Now, k < 2k ⇒ 2k < 2k + 1
⇒ (k + k) < 2k + 1
⇒ (k + 1) ≤ (k + k) < 2k + 1 [Since 1 ≤ k]
⇒ (k + 1) < 2k + 1
Therefore P(k + 1): (k + 1) < 2k + 1
⇒ P(k + 1) is true, whenever P(k) is true.
Thus, P(1) is true and P(k + 1) is true, whenever P(k) is true.
Hence, by the principle of mathematical induction P(n) is true for all n ∈ N.
Induction Proof
5. Using the principle of mathematical induction, prove that
(1² + 2² + …... + n²) > n³/3 for all values of n ∈ N.
Solution:
Let P(n): (1² + 2² + ….. + n²) > n³/3.
When = 1, LHS = 1² = 1 and RHS = 1³/3 = 1/3.
Since 1 > 1/3, it follows that P(1) is true.
Let P(k) be true. Then,
P(k): (1² + 2² + ….. + k²) > k³/3 .... (i)
Now, 1² + 2² + ..... + k² + (k + 1)²
= {1² + 2² + ..... + k² + (k + 1)²} + (k + 1)²
> k³/3 + (k + 1)² [using (i)]
= 1/3 ∙ (k³ + 3 + (k + 1)²) = 1/3 ∙ {k² + 3k² + 6k + 3}
= 1/3[k³ + 1 + 3k(k + 1) + (3k + 2)]
= 1/3 ∙ [(k + 1)³ + (3k + 2)]
> 1/3(k + 1)³
P(k + 1): 1² + 2² + ….... + k² + (k + 1) ² > 1/3 ∙ (k + 1)³.
P(k + 1) is true, whenever P(k) is true.
Thus P(1) is true and P(k + 1) is true whenever p(k) is true.
Hence, by the principle of mathematical induction, P(n) is true for all n ∈ N.
6. Using the principle of mathematical induction, prove that
(2n + 7) < (n + 3)² for all values of n ∈ N.
Solution:
Let P(n): (2n + 7) < (n + 3)²
When = 1, LHS = (2 × 1 + 7) = 9 and RHS = (1 + 3)² = 4² = 16.
Clearly, 9 < 16.
Thus, P(n) is true for n = 1, i.e., P(1) is true.
Let P(k) be true. Then
P(k): (2k + 7) < (k + 3)². ... (i)
Now, 2(k + 1) + 7 = (2k + 7) + 2
< (k + 3)² + 2 = (k² + 6k + 11) [using(i)]
< (k² + 8k + 16) = (k + 4)² = (k + 1 + 3)².
P(k + 1): 2(k + 1) + 7 < (k + 1 + 3)².
P(k + 1) is true, whenever P(k) is true.
Thus, P(1) is true and P(k + 1) is true, whenever P(k) is true.
Hence, by Principle of Mathematical Induction, P(n) is true for all n ∈ N.
From the above examples it can be understood that induction proof can be divided into four parts.
1) The Set P (n) that we need to prove.
2) The base step P (1).
3) Induction Hypothesis or the assumption step for P (k).
4) Inductive step where P (k+1) is to be proved.
Induction proof is used to prove general structures such as trees termed as Structural Induction. This structural induction is used in computer science like recursion. Also for correctness proofs induction proof is used for programs in computer science.
● Mathematical Induction
Problems on Principle of Mathematical Induction
Proof by Mathematical Induction
11 and 12 Grade Math
From Induction Proof to HOME PAGE
Didn't find what you were looking for? Or want to know more information about Math Only Math. Use this Google Search to find what you need.
Recent Articles
-
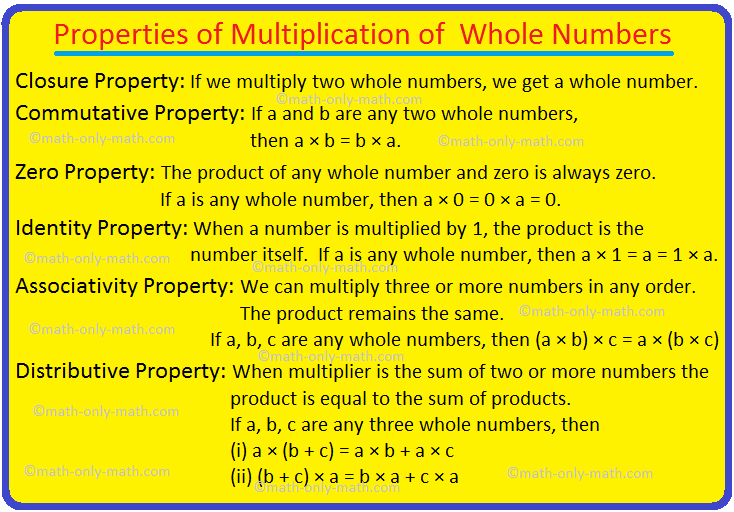
Properties of Multiplication | Multiplicative Identity | Whole Numbers
Jan 15, 25 12:08 AM
There are six properties of multiplication of whole numbers that will help to solve the problems easily. The six properties of multiplication are Closure Property, Commutative Property, Zero Property… -
Multiplication Table | Learn Tables from 0 – 25 | Multiplication Table
Jan 14, 25 11:53 PM
In math multiplication table we will learn the tables from 0 – 25. These multiplication tables help the students to learn the essential multiplication facts. Multiplication tables are very important f… -
3rd Grade Math Worksheets |3rd Grade Math Sheets|3rd Grade Math Lesson
Jan 14, 25 11:02 PM
3rd grade math worksheets is carefully planned and thoughtfully presented on mathematics for the students. Teachers and parents can also follow the worksheets to guide the students. -
3rd Grade Subtraction Worksheet | 3-Digit Subtraction Worksheets | Ans
Jan 14, 25 01:57 PM
In 3th Grade Addition Worksheet we will solve how to subtract 3-digit numbers by expansion, subtraction of 3-digit numbers without regrouping, subtraction of 3-digit numbers with regrouping, propertie… -
Facts about Subtraction | Subtraction of Small Numbers|Solved Examples
Jan 14, 25 12:29 AM
The operation to finding the difference between two numbers is called subtraction. Let us know some facts about subtraction which will help us to learn subtraction of large numbers. 1. Subtraction wit…


New! Comments
Have your say about what you just read! Leave me a comment in the box below. Ask a Question or Answer a Question.