Exact Value of sin 18°

We will learn to find the exact value of sin 18 degrees using the formula of multiple angles.

How to find exact value of sin 18°?

Let A = 18°                          

Therefore, 5A = 90°

⇒ 2A + 3A = 90˚

⇒ 2θ = 90˚ - 3A

Taking sine on both sides, we get

sin 2A = sin (90˚ - 3A) = cos 3A

⇒ 2 sin A cos A = 4 cos^3 A - 3 cos A

⇒ 2 sin A cos A - 4 cos^3A + 3 cos A = 0

⇒ cos A (2 sin A - 4 cos^2 A + 3) = 0 

Dividing both sides by cos A = cos 18˚ ≠ 0, we get

⇒ 2 sin θ - 4 (1 - sin^2 A) + 3 = 0

⇒ 4 sin^2 A + 2 sin A - 1 = 0, which is a quadratic in sin A

Therefore, sin θ = −2±√−4(4)(−1)2(4)

⇒ sin θ = −2±√4+168

⇒ sin θ = −2±2√58

⇒ sin θ = −1±√54

Now sin 18° is positive, as 18° lies in first quadrant.

Therefore, sin 18° = sin A = −1±√54

● Submultiple Angles






11 and 12 Grade Math

From Exact Value of sin 18° to HOME PAGE




Didn't find what you were looking for? Or want to know more information about Math Only Math. Use this Google Search to find what you need.



New! Comments

Have your say about what you just read! Leave me a comment in the box below. Ask a Question or Answer a Question.




Share this page: What’s this?

Recent Articles

RSS

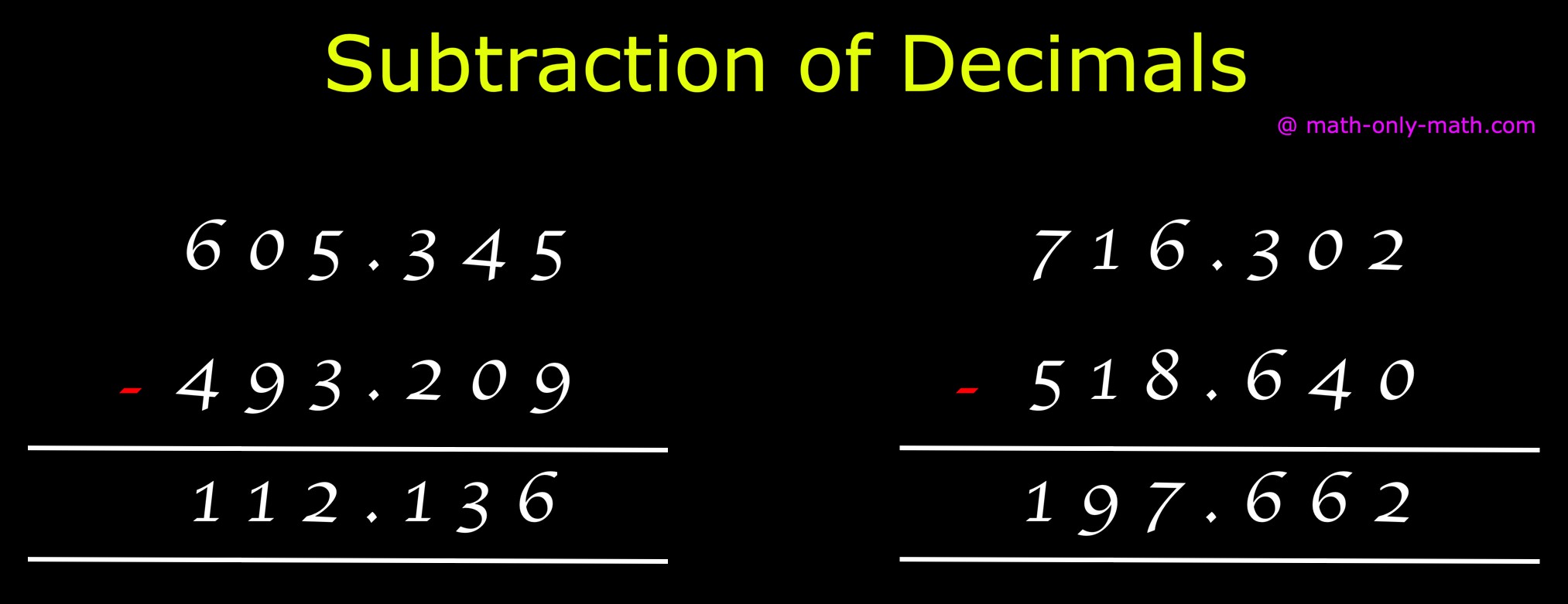
  1. Subtraction of Decimals | Subtracting Decimals | Decimal Subtraction

    Apr 24, 25 03:25 PM

    Subtraction of Decimals
    We will discuss here about the subtraction of decimals. Decimals are subtracted in the same way as we subtract ordinary numbers. We arrange the digits in columns

    Read More

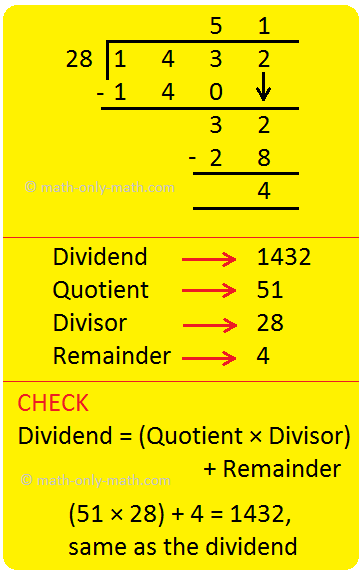
  2. How to Do Long Division? | Method | Steps | Examples | Worksheets |Ans

    Apr 24, 25 10:18 AM

    Long Division and Short Division Forms
    As we know that the division is to distribute a given value or quantity into groups having equal values. In long division, values at the individual place (Thousands, Hundreds, Tens, Ones) are dividend…

    Read More

  3. Division by Two-Digit Numbers | Knowledge of Estimation | Division

    Apr 24, 25 10:12 AM

    Divide 5-Digit by 2-Digit Number
    In division by two-digit numbers we will practice dividing two, three, four and five digits by two-digit numbers. Consider the following examples on division by two-digit numbers: Let us use our knowl…

    Read More

  4. Addition of Decimals | How to Add Decimals? | Adding Decimals|Addition

    Apr 24, 25 01:45 AM

    Addition of Decimals
    We will discuss here about the addition of decimals. Decimals are added in the same way as we add ordinary numbers. We arrange the digits in columns and then add as required. Let us consider some

    Read More

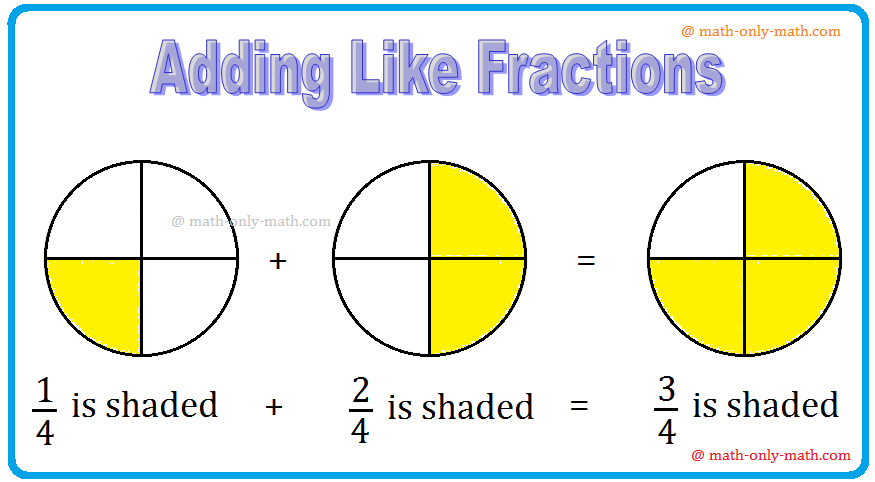
  5. Addition of Like Fractions | Examples | Videos | Worksheet | Fractions

    Apr 23, 25 09:23 AM

    Adding Like Fractions
    To add two or more like fractions we simplify add their numerators. The denominator remains same. Thus, to add the fractions with the same denominator, we simply add their numerators and write the com…

    Read More