Different Types of Polygons
The different types of polygons are discussed here.
In
any polygon a pair of sides that are inclined to each other meet at a
point. This point is called a vertex of the polygon. In the polygon
there are as many vertices as there are sides.
Definition of Polygon:
A polygon is a plane figure that is made by joining the line segments where each line segment meets exactly two other line segments.
The intersection point of two line segments is called the vertex of the polygon.
Polygons are classified according to their number of sides.
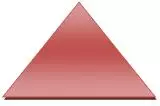
Polygon - Triangle:
The triangle has 3 sides and 3 vertices.
Triangle
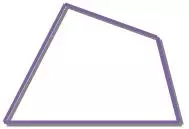
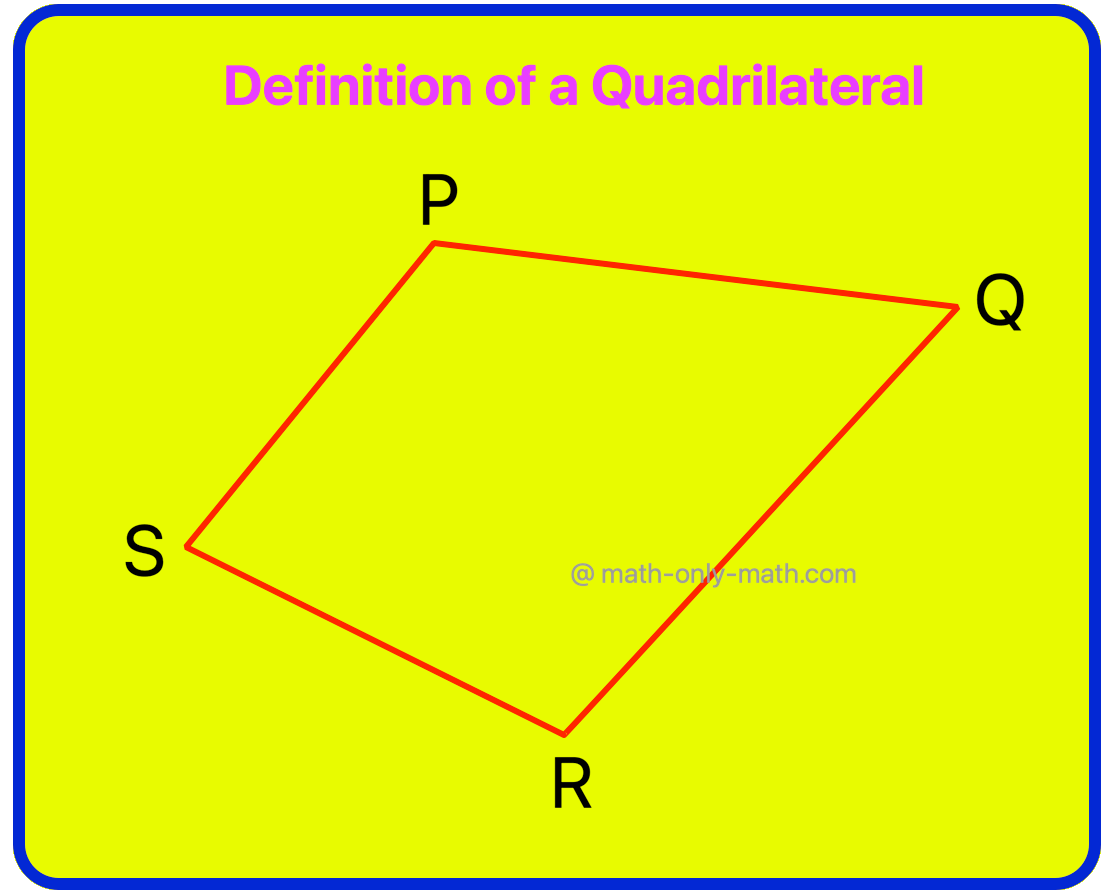
Polygon - Quadrilateral:
The quadrilateral has 4 sides and 4 vertices.
Quadrilateral
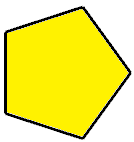
Polygon - Pentagon:
The pentagon has 5 sides and 5 vertices.
Pentagon
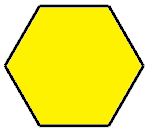
Polygon - Hexagon:
The hexagon has 6 sides and 6 vertices.
Hexagon
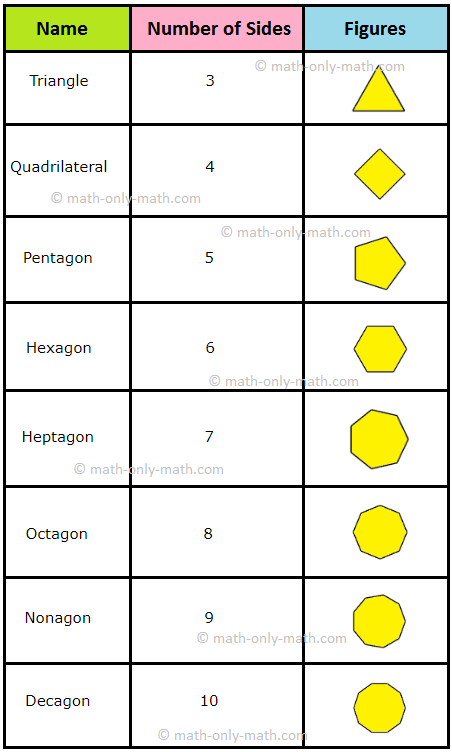
Different Types of Polygons:
|
Name |
Number of Sides |
Figures |
|
Triangle |
3 |
 |
|
Quadrilateral |
4 |
 |
|
Pentagon |
5 |
 |
|
Hexagon |
6 |
 |
|
Heptagon |
7 |
 |
|
Octagon |
8 |
 |
|
Nonagon |
9 |
 |
|
Decagon |
10 |
 |
Questions and Answers on Polygons:
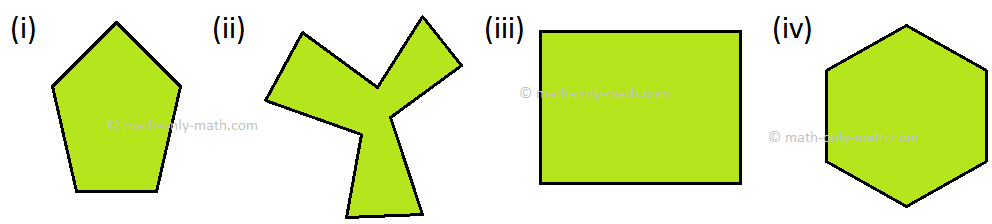
1. Classify the following polygons according to the number of sides:
Solution:
(i) Pentagon
(iii) Quadrilateral
(ii) Nonagon
(iv) Hexagon
|
2. In the adjacent figure, the polygon according to sides is __________. (i) hexagon (ii) octagon (iii) heptagon (iv) nonagon Solution: Here the no. of sides in the polygon = 8. Hence, it will be an octagon. |
You might like these
Line Symmetry | Vertical & Horizontal Lines of Symmetry | Symmetrical
Line symmetry is discussed here. We know that any object or shape which can be cut in two equal halves in such a way that both the parts are exactly the same is called symmetrical and the line which divides the shape in two equal halves is called the line of symmetry.
Simple Closed Curves | Types of Closed Curves | Collection of Curves
In simple closed curves the shapes are closed by line-segments or by a curved line. Triangle, quadrilateral, circle, etc., are examples of closed curves.
What is a Quadrilateral? | Symbol of a Quadrilateral □ | Rectangle
What is a quadrilateral? A simple closed curve or a polygon formed by four line-segments is called a quadrilateral.
Worksheet on Line Symmetry | Geometry Worksheet | Line of Symmetry
Worksheet on line symmetry we will solve different types of questions. 4th grade students can practice this geometry worksheet to get the basic ideas on line symmetry. Fill in the blanks: (i) A square has …... lines of symmetry. (ii) An equilateral triangle has
What is a Triangle? | Types of Triangle | Scalene Triangle | Isosceles
A simple closed curve or a polygon formed by three line-segments (sides) is called a triangle. The above shown shapes are triangles. The symbol of a triangle is ∆. A triangle is a polygon with three sides. In the given figure ABC is a triangle. AB, BC and CA are its sides.
Circle Math | Parts of a Circle | Terms Related to the Circle | Symbol
In circle math the terms related to the circle are discussed here. A circle is such a closed curve whose every point is equidistant from a fixed point called its centre. The symbol of circle is O. We have learned to draw a circle, by tracing the outlines of objects like a
What is a Polygon? | Simple Closed Curve | Triangle | Quadrilateral
What is a polygon? A simple closed curve made of three or more line-segments is called a polygon. A polygon has at least three line-segments.
Symmetrical Shapes | One, Two, Three, Four & Many-line Symmetry
Symmetrical shapes are discussed here in this topic. Any object or shape which can be cut in two equal halves in such a way that both the parts are exactly the same is called symmetrical. The line which divides the shape is called the symmetry. So, if we place a mirror
Classification of Angles | Types of Angles | Acute, Right, Obtuse, ...
Classification of angles on the basis of their degree measures are given below: Acute angle: An angle whose measure is more than 0° but less than 90° is called an acute angle. Angles having mag
Measuring the Line Segment | Comparing Line Segments
We will discuss here about measuring the line segment i.e. how to draw and measure a line segment using scale or ruler. We can measure a line segment by two ways. (i) With the help of scale
Comparing Different Line Segments | Difference of two line segments
We will discuss here about comparing different line segments. Different line segments can be of different lengths. We can compare the lengths of the different line segments
Constructing a Line Segment |Construction of Line Segment|Constructing
We will discuss here about constructing a line segment. We know how to draw a line segment of a certain length. Suppose we want to draw a line segment of 4.5 cm length.
Measuring an Angle | Using Protractor | Comparison of Angles | Example
We will discuss here about measuring an angle with the help of a protractor. You can find it in your Geometry Box. It has the semi-circular shape. Its semi-circular edge is divided
Perimeter of a Triangle | Perimeter of a Triangle Formula | Examples
We will discuss here how to find the perimeter of a triangle. We know perimeter of a triangle is the total length (distance) of the boundary of a triangle. Perimeter of a triangle is the sum of lengths of its three sides. The perimeter of a triangle ABC Perimeter
Perimeter of a Rectangle | How to Find the Perimeter of a Rectangle?
We will discuss here how to find the perimeter of a rectangle. We know perimeter of a rectangle is the total length (distance) of the boundary of a rectangle. ABCD is a rectangle. We know that the opposite sides of a rectangle are equal. AB = CD = 5 cm and BC = AD = 3 cm
Related Concepts on Geometry - Simple Shapes & Circle
● Polygon
● Angle
● Triangle
From Different Types of Polygons to HOME PAGE
Didn't find what you were looking for? Or want to know more information about Math Only Math. Use this Google Search to find what you need.
Recent Articles
-
5th Grade Relation Between HCF and LCM | Solved Examples | Worksheet
Mar 25, 25 10:23 AM
Here we will discuss about the relationship between hcf and lcm of two numbers. Product of two numbers = Product of H.C.F. and L.C.M. of the numbers. Solved Examples on 5th Grade Relation Between HCF… -
5th Grade Least Common Factor (LCM) | Factorization & Division Method
Mar 25, 25 02:39 AM
We already familiar with the least common multiple which is the smallest common multiple of the numbers. The least (lowest) common multiple of two or more numbers is exactly divisible by each of the g… -
5th Grade Highest Common Factor | HCF | GCD|Prime Factorization Method
Mar 24, 25 11:58 PM
The highest common factor (H.C.F.) of two or more numbers is the highest or greatest common number or divisor which divides each given number exactly. Hence, it is also called Greatest Common Divisor… -
5th Grade Factors and Multiples | Definitions | Solved Examples | Math
Mar 23, 25 02:39 PM
Here we will discuss how factors and multiples are related to each other in math. A factor of a number is a divisor which divides the dividend exactly. A factor of a number which is a prime number is… -
Adding 2-Digit Numbers | Add Two Two-Digit Numbers without Carrying
Mar 23, 25 12:43 PM
Here we will learn adding 2-digit numbers without regrouping and start working with easy numbers to get acquainted with the addition of two numbers.
New! Comments
Have your say about what you just read! Leave me a comment in the box below. Ask a Question or Answer a Question.