Coin Toss Probability Worksheet
Practice different types of coin toss questions like tossing a coin, tossing two coins simultaneously and tossing three coins simultaneously in coin toss probability worksheet.
1. If a coin is tossed 500 times and the tail appears 159 times, find the probability of getting a tail.
2. On tossing a coin 1000 times, the head appeared 465 times and the tail appeared 535 times. Find the probability of getting a (i) head (ii) tail.
3. Three coins were tossed simultaneously 200 times. The outcomes are given in the table below:
|
Outcome |
3 heads |
2 heads |
1 head |
No head |
Total |
|
Frequency |
24 |
70 |
76 |
30 |
200 |
Find the probability of getting (i) exactly two heads (ii) 1 head 2 tails (iii) at least one head.
4. Write down the total number of possible outcomes when the
following experiment is done.
(i) A coin is tossed
(ii) A die is rolled
(iii) Two coins are tossed
5. Two coins are tossed simultaneously. Write the number of favourable outcomes for the following events:
(i) getting at least one tail
(ii) getting on tail.
6. Fill in the blanks.
(i) A coin tossed at random. The probability of getting a tail is ...... .
(ii) A coin tossed at random. The probability of getting a head is ...... .
7. Two coins are tossed together. What is the probability of getting different faces on the coin?
8. A coin is tossed twice. What is the probability of getting
(i) no head
(ii) at most 1 tail
9. Three coins are tossed simultaneously. What is the probability of getting at least two heads?
Note: All possible outcomes are HHH, HHT, HTH, THH, HTT, THT, TTH, TTT.
Answers for the coin toss probability worksheet are given below to check the exact answers.
Answers
1. 159500
2. (i) 93200
(ii) 107200
3. (i) 720
(ii) 1950
(iii) 1720
4. (i) 2
(ii) 6
(iii) 4
5. (i) 3
(ii) 1
6. (i) 12
(ii) 12
7. 12
8. (i) 14
(ii) 34
9. 12
From Coin Toss Probability Worksheet to HOME PAGE
Didn't find what you were looking for? Or want to know more information about Math Only Math. Use this Google Search to find what you need.
Recent Articles
-
Converting Fractions to Decimals | Solved Examples | Free Worksheet
Apr 26, 25 05:04 PM
In converting fractions to decimals, we know that decimals are fractions with denominators 10, 100, 1000 etc. In order to convert other fractions into decimals, we follow the following steps: -
Converting Decimals to Fractions | Solved Examples | Free Worksheet
Apr 26, 25 04:56 PM
In converting decimals to fractions, we know that a decimal can always be converted into a fraction by using the following steps: Step I: Obtain the decimal. Step II: Remove the decimal points from th… -
Worksheet on Decimal Numbers | Decimals Number Concepts | Answers
Apr 26, 25 03:48 PM
Practice different types of math questions given in the worksheet on decimal numbers, these math problems will help the students to review decimals number concepts. -
Multiplication Table of 4 |Read and Write the Table of 4|4 Times Table
Apr 26, 25 01:00 PM
Repeated addition by 4’s means the multiplication table of 4. (i) When 5 candle-stands having four candles each. By repeated addition we can show 4 + 4 + 4 + 4 + 4 = 20 Then, four 5 times -
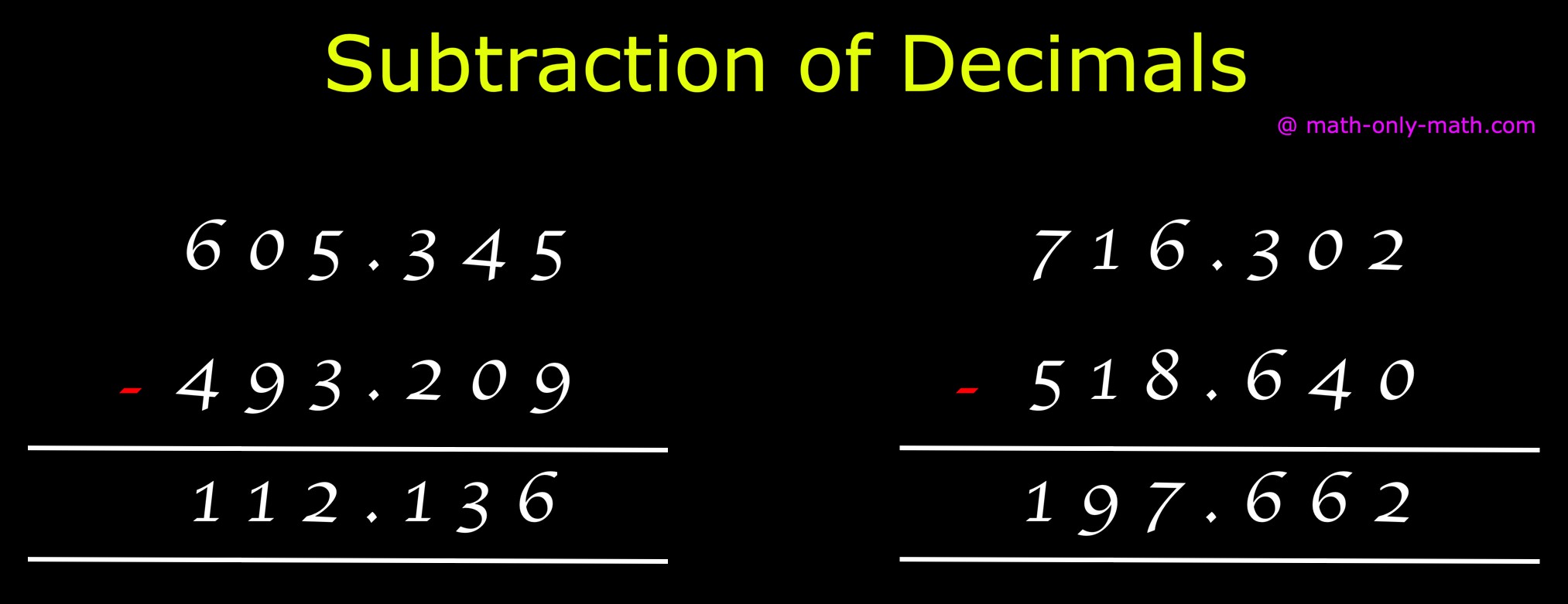
Subtraction of Decimals | Subtracting Decimals | Decimal Subtraction
Apr 24, 25 03:25 PM
We will discuss here about the subtraction of decimals. Decimals are subtracted in the same way as we subtract ordinary numbers. We arrange the digits in columns





New! Comments
Have your say about what you just read! Leave me a comment in the box below. Ask a Question or Answer a Question.