Circumcentre and Incentre of a Triangle

We will discuss circumcentre and incentre of a triangle.

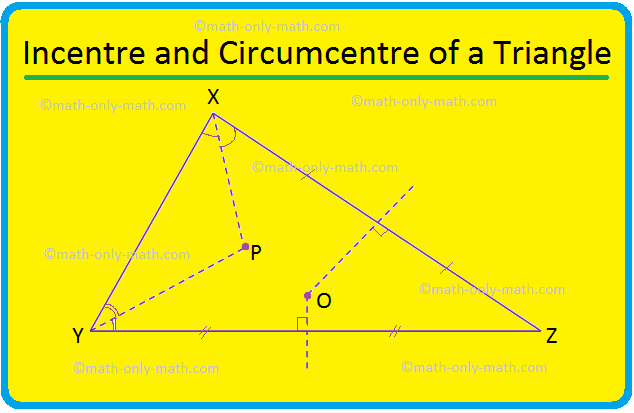
In general, the incentre and the circumcentre of a triangle are two distinct points.

Incentre and Circumcentre of a Triangle

Here in the triangle XYZ, the incentre is at P and the circumcentre is at O. 

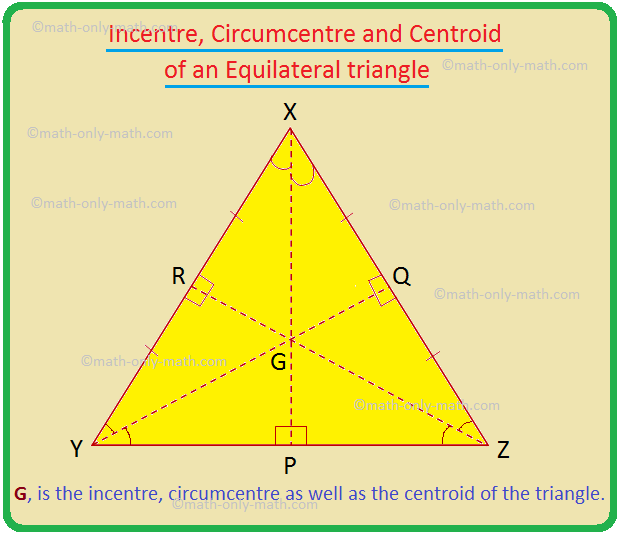
A special case: an equilateral triangle, the bisector of the opposite side, so it is also a median.

In the ∆XYZ, XP, YQ and ZR are the bisectors of ∠YXZ, ∠XYZ and ∠YZX respectively; they are also the perpendicular bisectors of YZ, ZX and XY respectively; they are also the medians of the triangle. So, their point of intersection, G, is the incentre, circumcentre as well as the centroid of the triangle. So, in an equilateral triangle, these three points are coincident.

Incentre, Circumcentre and Centroid of an Equilateral Triangle

If XY = YZ = ZX = 2a then in ∆XYP, YP = a and XP = \(\sqrt{3}\)a.

Now, XG = \(\frac{}{}\) = \(\frac{2}{3}\)XP = \(\frac{2\sqrt{3}a}{3}\), and GP = \(\frac{1}{3}\)XP = \(\frac{\sqrt{3}a}{3}\).

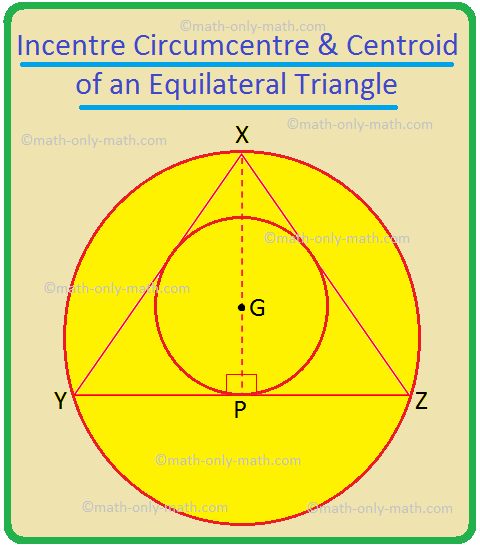
Therefore, radius of the circumcircle is XG = \(\frac{2\sqrt{3}a}{3}\) = \(\frac{2a}{\sqrt{3}}\) = \(\frac{Any side of the equilateral triangle}{\sqrt{3}}\).

Incentre, Circumcentre & Centroid of an Equilateral Triangle

The radius of the incircle = GP = \(\frac{a}{\sqrt{3}}\) = \(\frac{2a}{2\sqrt{3}}\) = \(\frac{Any side of the equilateral triangle}{2\sqrt{3}}\).

Therefore, radius of the circumcircle of an equilateral triangle = 2 × (Radius of the incircle).

You might like these

  • Problems on Relation Between Tangent and Secant | Square of Tangent

    Here we will solve different types of Problems on relation between tangent and secant. 1. XP is a secant and PT is a tangent to a circle. If PT = 15 cm and XY = 8YP, find XP. Solution: XP = XY + YP = 8YP + YP = 9YP. Let YP = x. Then XP = 9x. Now, XP × YP = PT^2, as the

  • Problems on Two Tangents to a Circle from an External Point | Diagram

    We will solve some Problems on two tangents to a circle from an external point. 1. If OX any OY are radii and PX and PY are tangents to the circle, assign a special name to the quadrilateral OXPY and justify your answer. Solution: OX = OY, are radii of a circle are equal.

  • Solved Examples on the Basic Properties of Tangents | Tangent Circle

    The solved examples on the basic properties of tangents will help us to understand how to solve different type problems on properties of triangle. 1. Two concentric circles have their centres at O. OM = 4 cm and ON = 5 cm. XY is a chord of the outer circle and a tangent to

  • Incircle of a Triangle |Incentre of the Triangle|Point of Intersection

    We will discuss here the Incircle of a triangle and the incentre of the triangle. The circle that lies inside a triangle and touches all the three sides of the triangle is known as the incircle of the triangle. If all the three sides of a triangle touch a circle then the

  • Circumcircle of a Triangle | Circumcentre of the Triangle | Diagram

    We will discuss here the Circumcircle of a Triangle and the circumcentre of a triangle. A tangent that passes through the three vertices of a triangle is known as the circumcircle of the triangle. When the vertices of a triangle lie on a circle, the sides of the triangle

  • Examples of Loci Based on Circles Touching Straight Lines | Diagram

    We will discuss here some Examples of Loci Based on Circles Touching Straight Lines or Other Circles. 1. The locus of the centres of circles touching a given line XY at a point M, is the straight line perpendicular to XY at M. Here, PQ is the required locus. 2. The locus of

  • Important Properties of Transverse Common Tangents |Proof with Diagram

    We will discuss about the important properties of transverse common tangents. I. The two transverse common tangents drawn to two circles are equal in length. Given: WX and YZ are two transverse common tangents drawn to the two given circles with centres O and P. WX and YZ

  • Problems on Common Tangents to Two Circles | Transverse Common Tangent

    Here we will solve different types of problems on common tangents to two circles. 1.There are two circles touch each other externally. Radius of the first circle with centre O is 8 cm. Radius of the second circle with centre A is 4 cm Find the length of their common tangent

  • Equilateral Triangle Inscribed | Sum of Three Angles of a Triangle

    We will prove that, PQR is an equilateral triangle inscribed in a circle. The tangents at P, Q and R form the triangle P’Q’R’. Prove that P’Q’R’ is also an equilateral triangle. Solution: Given: PQR is an equilateral triangle inscribed in a circle whose centre is O.

  • Measure of the Angles of the Cyclic Quadrilateral | Proof with Diagram

    We will prove that, in the figure ABCD is a cyclic quadrilateral and the tangent to the circle at A is the line XY. If ∠CAY : ∠CAX = 2 : 1 and AD bisects the angle CAX while AB bisects ∠CAY then find the measure of the angles of the cyclic quadrilateral. Also, prove that DB

  • Tangent is Parallel to a Chord of a Circle | Extremities of the Chord

    We will prove that, A tangent, DE, to a circle at A is parallel to a chord BC of the circle. Prove that A is equidistant from the extremities of the chord. Solution: Proof: Statement 1. ∠DAB = ∠ACB 2. ∠DAB = ∠ABC 3. ∠ACB = ∠ABC

  • Contact of Two Circles | Point of Contact of Two Circles | Proof

    Here we will prove that two circles with centres X and Y touch externally at T. A straight line is drawn through T to cut the circles at M and N. Proved that XM is parallel to YN. Solution: Given: Two circles with centres X and Y touch externally at T. A straight line is

  • Two Parallel Tangents of a Circle Meet a Third Tangent | Proof

    Here we will prove that two parallel tangents of a circle meet a third tangent at points A and B. Prove that AB subtends a right angle at the centre. Solution: Given: CA, AB and EB are tangents to a circle with centre O. CA ∥ EB. To prove: ∠AOB = 90°. Proof: Statement

  • Two Tangents are Drawn to a Circle from an External Point | Proof

    We will prove that the tangents MX and MY are drawn to a circle with centre O from an external point M. Prove that ∠XMY = 2∠OXY. Solution: Proof: Statement 1. In ∆MXY, MX = MY. 2. ∠MXY = ∠MYX = x°. 3. ∠XMY = 180° - x°. 4. OX ⊥ XM, i.e., ∠OXM = 90°. 5. ∠OXY = 90° - ∠MXY

  • Transverse Common Tangents | Circles lie on Opposite Sides | Diagram

    A common tangent is called a transverse common tangent if the circles lie on opposite sides of it. In the figure, WX is a transverse common tangent as the circle with centre O lies below it and the circle with P lie above it. YZ is the other transverse common tangent as the

  • Important Properties of Direct Common Tangents |Explained With Diagram

    Important Properties of Direct common tangents. The two direct common tangents drawn to two circles are equal in length. The point of intersection of the direct common tangents and the centres of the circles are collinear. The length of a direct common tangent to two circles

  • Direct Common Tangents | Common Tangent | Three Different Cases

    A common tangent is called a direct common tangent if both the circles lie on the same side of it. The figures given below shows common tangents in three different cases, that is when the circles are apart, as in (i); when they are touching each other as in (ii); and when

  • Chord and Tangent Intersect Externally | The Length of the Tangent

    Here we will prove that if a chord and a tangent intersect externally then the product of the lengths of the segments of the chord is equal to the square of the length of the tangent from the point of contact to the point of intersection. Given: XY is a chord of a circle and

  • Problems on Properties of Tangents | Tangents of Circles and Angles

    Here we will solve different types of Problems on properties of tangents. 1. A tangent, PQ, to a circle touches it at Y. XY is a chord such that ∠XYQ = 65°. Find ∠XOY, where O is the centre of the circle. Solution: Let Z be any point on the circumference in the segment

  • Angles between the Tangent and the Chord | A Line Touches a Circle

    Here we will prove that if a line touches a circle and from the point of contact a chord is down, the angles between the tangent and the chord are respectively equal to the angles in the corresponding alternate segments. Given: A circle with centre O. Tangent XY touches






10th Grade Math

From Circumcentre and Incentre of a Triangle to HOME PAGE


New! Comments

Have your say about what you just read! Leave me a comment in the box below. Ask a Question or Answer a Question.



Didn't find what you were looking for? Or want to know more information about Math Only Math. Use this Google Search to find what you need.