Worksheet on Theorems of Solid Geometry
Practice the questions given in the worksheet on theorems of solid geometry. Keeping in mind the theorems of solid geometry students need to practice the questions by solving it step-by-step.
1. Find the locus in the three dimensional space of a point equidistant from two given points.
2. Find the locus of a point in space equidistant from three given non-collinear point.
3. O is the circum-centre of a given triangle ABC. If P be any point outside the plane of the triangle ABC such that PA = PB = PC, show that PO is perpendicular to the plane of the triangle ABC.
4. Prove that one and only one perpendicular can be drawn to a plane through a given point outside the plane.
5. The straight line OA, drawn through the centre O of a circle, is perpendicular to the two radii OB and OC of the circle. Prove that all points on the circumference of the circle are equidistant from any points on the line OA.
6. P is a point outside a given plane and O, A, B, C and D are point in the plane such that POA = POB = 1 right angle. If PA = PB = PC = PD, show that the points A, B, C and D are concyclic. Determine the centre of the circle passing through A. B, C and D.
7. How many horizontal lines can be drawn through a given point in a vertical line and how do they lie.
8. If a triangle revolves about its base, prove that its vertex describes a circle.
9. Through the intersection O of the diagonals of a horizontal square ABCD, a vertical line OP is drawn. Prove that, PA = PB = PC = PD.
10. Find a point on a given straight line in space which is equidistant from two given points outside the line. When this impossible?
11. Prove that the straight lines joining the middle points of the opposite sides of a skew quadrilateral bisect each other.
12. The straight lines AB and CD are perpendicular to a plane and meet it at B and D respectively. If the lines are on the same side of the plane and AB = CD, prove that ABCD is a rectangle.
13. P is a point outside the plane of two parallel straight lines AB and CD. From the point P, PL is drawn perpendicular to AB and LM is drawn perpendicular to CD. Show that PM is perpendicular to CD.
14. Two Straight lines AB and AC intersect at right angles. From B a perpendicular BD is drawn to the plane of △ ABC. Prove that AD is perpendicular to the straight line AC.
15. AB, CD, EF are three parallel straight lines not lying in one plane and their extremities form two triangles ACE and BDF. If AB = CD = EF, prove that the triangles are congruent.
● Geometry
- Solid Geometry
- Worksheet on Solid Geometry
- Theorems on Solid Geometry
- Theorems on Straight Lines and Plane
- Theorem on Co-planar
- Theorem on Parallel Lines and Plane
- Theorem of Three Perpendiculars
- Worksheet on Theorems of Solid Geometry
11 and 12 Grade Math
From Worksheet on Theorems of Solid Geometry to HOME PAGE
Recent Articles
-
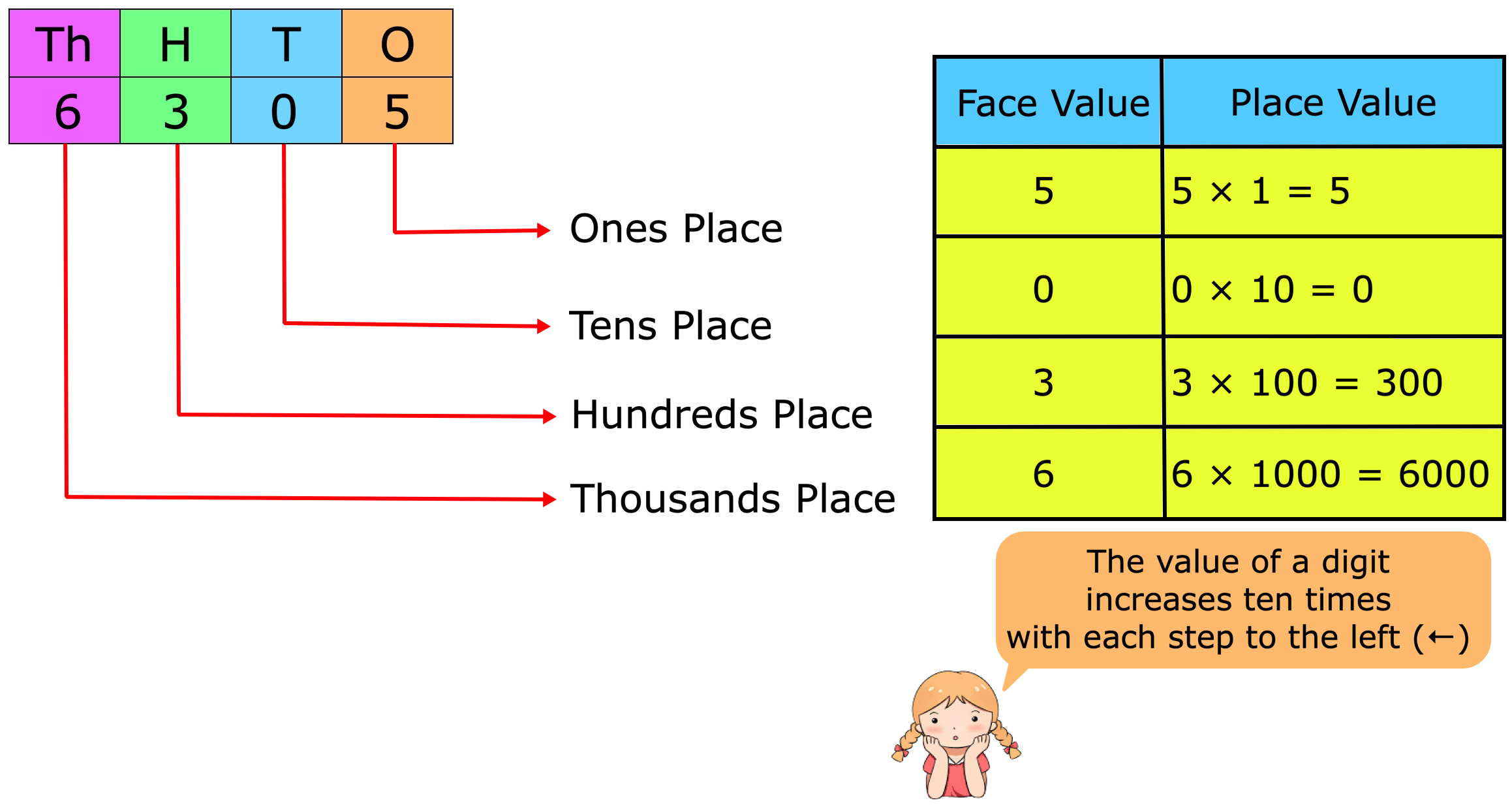
Place Value and Face Value | Place and Face Value of Larger Number
Apr 13, 25 03:12 PM
The place value of a digit in a number is the value it holds to be at the place in the number. We know about the place value and face value of a digit and we will learn about it in details. We know th… -
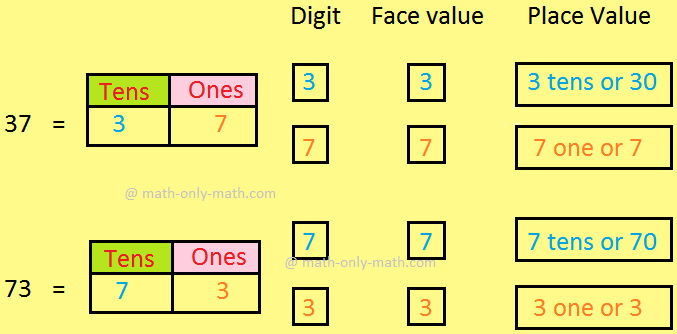
Face Value and Place Value|Difference Between Place Value & Face Value
Apr 13, 25 03:07 PM
What is the difference between face value and place value of digits? Before we proceed to face value and place value let us recall the expanded form of a number. The face value of a digit is the digit… -
Place Value and Face Value | Basic Concept on Place Value | Face Value
Apr 13, 25 02:59 PM
Learn the easiest way to understand the basic concept on place value and face value in the second grade. Suppose we write a number in figures 435 in words we write four hundred thirty five. -
Expressing Place Value and Face Value | International & Indian System
Apr 13, 25 02:35 PM
We will learn expressing place value and face value of a digit in any number in International and Indian system. Place value: We know how to find out the place value of a digit in any number. -
5th Grade Decimals | Word Problem on Decimals | Concept of Decimals
Apr 13, 25 02:16 PM
A fractional number whose denominator is 10 or multiple of 10 is called a decimal. Every decimal has two parts whole number part and decimal part. These two parts are separated by a dot or point. This…



New! Comments
Have your say about what you just read! Leave me a comment in the box below. Ask a Question or Answer a Question.