Word Problems on Simultaneous Linear Equations
Solving the solution of two variables of system equation that leads for the word problems on simultaneous linear equations is the ordered pair (x, y) which satisfies both the linear equations.
Problems of different problems with the help of linear simultaneous equations:
We have already learnt the steps of forming simultaneous equations from mathematical problems and different methods of solving simultaneous equations.
In connection with any problem, when we have to find the values of two unknown quantities, we assume the two unknown quantities as x, y or any two of other algebraic symbols.
Then we form the equation according to the given condition or conditions and solve the two simultaneous equations to find the values of the two unknown quantities. Thus, we can work out the problem.
Worked-out examples for the word problems on simultaneous linear equations:
1. The sum of two number is 14 and their difference is 2. Find the numbers.
Solution:
Let the two numbers be x and y.
x + y = 14 ………. (i)
x - y = 2 ………. (ii)
Adding equation (i) and (ii), we get 2x = 16
or, 2x/2 = 16/2
or, x = 16/2
or, x = 8
Substituting the value x in equation (i), we get
8 + y = 14
or, 8 – 8 + y = 14 - 8
or, y = 14 - 8
or, y = 6
Therefore, x = 8 and y = 6
Hence, the two numbers are 6 and 8.
2. In a two digit number. The units digit is thrice the tens digit. If 36 is added to the number, the digits interchange their place. Find the number.
Solution:
Let the digit in the units place is x
And the digit in the tens place be y.
Then x = 3y and the number = 10y + x
The number obtained by reversing the digits is 10x + y.
If 36 is added to the number, digits interchange their places,
Therefore, we have 10y + x + 36 = 10x + y
or, 10y – y + x + 36 = 10x + y - y
or, 9y + x – 10x + 36 = 10x - 10x
or, 9y - 9x + 36 = 0
or, 9x - 9y = 36
or, 9(x - y) = 36
or, 9(x - y)/9 = 36/9
or, x - y = 4 ………. (i)
Substituting the value of x = 3y in equation (i), we get
3y - y = 4
or, 2y = 4
or, y = 4/2
or, y = 2
Substituting the value of y = 2 in equation (i),we get
x - 2 = 4
or, x = 4 + 2
or, x = 6
Therefore, the number becomes 26.
3. If 2 is added to the numerator and denominator it becomes 9/10 and if 3 is subtracted from the numerator and denominator it become 4/5. Find the fractions.
Solution:
Let the fraction be x/y.
If 2 is added to the numerator and denominator fraction becomes 9/10 so, we have
(x + 2)/(y + 2) = 9/10
or, 10(x + 2) = 9(y + 2)
or, 10x + 20 = 9y + 18
or, 10x – 9y + 20 = 9y – 9y + 18
or, 10x – 9x + 20 – 20 = 18 – 20
or, 10x – 9y = -2 ………. (i)
If 3 is subtracted from numerator and denominator the fraction becomes 4/5 so, we have
(x – 3)/(y – 3) = 4/5
or, 5(x – 3) = 4(y – 3)
or, 5x – 15 = 4y – 12
or, 5x – 4y – 15 = 4y – 4y – 12
or, 5x – 4y – 15 + 15 = – 12 + 15
or, 5x – 4y = 3 ………. (ii)
So, we have 10x – 9y = – 2 ………. (iii)
and 5x – 4y = 3 ………. (iv)
Multiplying both sided of equation (iv) by 2, we get
10x – 8y = 6 ………. (v)
Now, solving equation (iii) and (v) , we get
10x – 9y = -2
10x – 8y = 6
- y = - 8
y = 8
Substituting the value of y in equation (iv)
5x – 4 × (8) = 3
5x – 32 = 3
5x – 32 + 32 = 3 + 32
5x = 35
x = 35/5
x = 7
Therefore, fraction becomes 7/8.
4. If twice the age of son is added to age of father, the sum is 56. But if twice the age of the father is added to the age of son, the sum is 82. Find the ages of father and son.
Solution:
Let father’s age be x years
Son’s ages = y years
Then 2y + x = 56 …………… (i)
And 2x + y = 82 …………… (ii)
Multiplying equation (i) by 2, (2y + x = 56 …………… × 2)we get
or, 3y/3 = 30/3
or, y = 30/3
or, y = 10 (solution (ii) and (iii) by subtraction)
Substituting the value of y in equation (i), we get;
2 × 10 + x = 56
or, 20 + x = 56
or, 20 – 20 + x = 56 – 20
or, x = 56 – 20
x = 36
5. Two pens and one eraser cost Rs. 35 and 3 pencil and four erasers cost Rs. 65. Find the cost of pencil and eraser separately.
Solution:
Let the cost of pen = x and the cost of eraser = y
Then 2x + y = 35 ……………(i)
And 3x + 4y = 65 ……………(ii)
Multiplying equation (i) by 4,
Subtracting (iii) and (ii), we get;
5x = 75
or, 5x/5 = 75/5
or, x = 75/5
or, x = 15
Substituting the value of x = 15 in equation (i) 2x + y = 35 we get;
or, 2 × 15 + y = 35
or, 30 + y = 35
or, y = 35 – 30
or, y = 5
Therefore, the cost of 1 pen is Rs. 15 and the cost of 1 eraser is Rs. 5.
● Simultaneous Linear Equations
Solvability of Linear Simultaneous Equations
Word Problems on Simultaneous Linear Equations
Word Problems on Simultaneous Linear Equations
Practice Test on Word Problems Involving Simultaneous Linear Equations
● Simultaneous Linear Equations - Worksheets
Worksheet on Simultaneous Linear Equations
Worksheet on Problems on Simultaneous Linear Equations
8th Grade Math Practice
From Word Problems on Simultaneous Linear Equations to HOME PAGE
Didn't find what you were looking for? Or want to know more information about Math Only Math. Use this Google Search to find what you need.
Recent Articles
-
Adding 2-Digit Numbers | Add Two Two-Digit Numbers without Carrying
Mar 23, 25 12:43 PM
Here we will learn adding 2-digit numbers without regrouping and start working with easy numbers to get acquainted with the addition of two numbers. -
Worksheet on 12 Times Table | Printable Multiplication Table | Video
Mar 23, 25 10:28 AM
Worksheet on 12 times table can be printed out. Homeschoolers can also use these multiplication table sheets to practice at home. -
Vertical Subtraction | Examples | Word Problems| Video |Column Method
Mar 22, 25 05:20 PM
Vertical subtraction of 1-digit number are done by arranging the numbers column wise i.e., one number under the other number. How to subtract 1-digit number vertically? -
Worksheet on 11 Times Table | Printable Multiplication Table | Video
Mar 22, 25 05:08 PM
Worksheet on 11 times table can be printed out. Homeschoolers can also use these multiplication table sheets to practice at home. -
Worksheet on 10 Times Table | Printable Multiplication Table | Video
Mar 21, 25 03:46 PM
Worksheet on 10 times table can be printed out. Homeschoolers can also use these multiplication table sheets to practice at home.
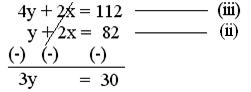
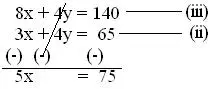






New! Comments
Have your say about what you just read! Leave me a comment in the box below. Ask a Question or Answer a Question.