Theorems on Locus of a Point which is Equidistant from Two Fixed Points
The locus of a point which is equidistant from two fixed points is the perpendicular bisector of the line segment joining the two fixed points.
Given,
Let X and Y be two given fixed points. PQ is the path traced out by the moving point P such that each point on it is equidistant from X and Y. Therefore, PX = PY.
To prove: PQ is the perpendicular bisector of the line segment XY.
Construction: Join X to Y. Let PQ cut XY at O.
Proof:
From △PXO and △PYO,
PX and PY (Given)
XO = YO (Since, every point of PQ is equidistant from X and Y, and O is a point on PQ.)
PO = PO (Common side.)
Therefore, by the SSS criterion of congruency△PXO ≅ △PYO.
Now ∠POX = ∠POY (since, corresponding parts of congruent triangles are congruent.)
Again ∠POX + ∠POY = 180° (Since, XOY is a straight line.
Therefore, ∠POX = ∠POY = 180°2 = 90°
Also, PQ bisects XY (Since, XO = YO)
Therefore, PQ ⊥ XY and PQ bisects XY, i.e., PQ is the perpendicular bisector of XY (Proved)
● Loci
From Theorems on Locus of a Point which is Equidistant from Two Fixed Points to HOME
Didn't find what you were looking for? Or want to know more information about Math Only Math. Use this Google Search to find what you need.
Recent Articles
-
Addition of 3-Digit Numbers with Regrouping | Step-by-Step Method
Apr 07, 25 02:53 AM
We will learn addition of 3-digit numbers with regrouping. Do you know the addition of 3-digit number? Yes I know how to add the numbers. Now, let us learn to add the 3-digit numbers with regrouping. -
Worksheet on Fractions | Questions on Fractions | Representation | Ans
Apr 07, 25 02:37 AM
In worksheet on fractions, all grade students can practice the questions on fractions on a whole number and also on representation of a fraction. This exercise sheet on fractions can be practiced -
Counting Numbers from 1 to 50 | Match the Number | Missing Numbers
Apr 04, 25 03:46 PM
In counting numbers from 1 to 50, recognize the numbers, count and then join the numbers in the correct number order. Here we mainly need eye-hand coordination to draw the picture and maintain the num -
Counting Eleven to Twenty with Numbers and Words |Numbers from 11 - 20
Apr 04, 25 03:21 PM
Counting eleven to twenty with numbers and words are explained below. One ten and one more is eleven. Eleven comes after ten. One ten and two more is twelve. Twelve comes after eleven. -
5th Grade BODMAS Rule Worksheet | PEMDAS | Order of operations|Answers
Apr 03, 25 03:11 PM
In 5th Grade BODMAS Rule Worksheet you will get different types of problems on mathematical expressions involving different operations, mathematical expression with 'brackets' and 'of' and simplifying…
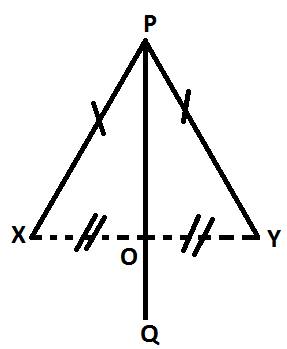



New! Comments
Have your say about what you just read! Leave me a comment in the box below. Ask a Question or Answer a Question.