Slope of a Straight Line

What is slope of a straight line?

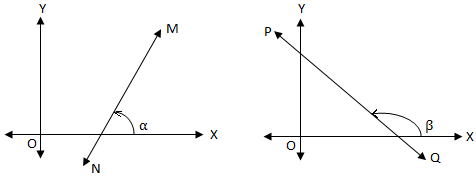
The tangent value of any trigonometric angle that a straight line makes with the positive direction of the x-axis in anticlockwise direction is called the slope or gradient of a straight line.

The angle of inclination of a line is the angle made by the line with the positive direction of the x-axis. It is usually measured from the positive x-axis in the anti-clockwise direction.

The slope of the line is generally denoted by ‘m’. Thus, m = tan θ. The gradient or slope of a line (not parallel to the axis of y) is the trigonometrical tangent of the angle which the line makes with the positive direction of the x-axis. Thus, if a line makes an angle θ with the positive direction of the x-axis, then its slope will be tan θ. The slop of a line is positive or negative according as θ is acute or obtuse. Sine a line parallel to x-axis makes an angle of 0° with x-axis, therefore its slope is tan 0° = 0. A line parallel to y-axis that is i.e., perpendicular to x-axis makes an angle of 90° with x-axis, so its slope is tan \(\frac{π}{2}\) = infinity. Also the slope of a line equally inclined with axes is 1 or -1 as it makes 45° or 135° angle with x-axis.

In short, the slope of a line is the trigonometrical tangent of its inclination.

In the above figure the inclination of the lines MN and PQ are α and β respectively.

 

Solved examples to find the slope of a straight line:

1. Find the slope or gradient of a straight line whose inclination to the positive (+ve) direction of x-axis in anticlockwise direction is

(i) 30°

(ii) 0°

(iii) 45°

(iv) 135°

Solution:

(i) 30°

Slope or gradient = tan 30° = \(\frac{1}{√3}\)

(ii) 0°

Slope or gradient = tan 0° = 0

(iii) 45°

Slope or gradient = tan 45° = 1

(iv) 135°

Slope or gradient = tan 135° = -cot 40° = -1

2. What can be said regarding a line if its Slope or gradient is

(i) (+ve)

(ii) Zero (0)

(iii) (-ve)

Solution:

Let ∅ be the angle of inclination of the given straight line with the positive (+ve) direction of x-axis in anticlockwise direction. Then its Slope or gradient is given by m = tan ∅.

(i) Slope or gradient is positive (+ve)

⇒ m = tan ∅ > 0

⇒ ∅ lies between 0° and 90°

⇒ ∅ is an acute angle.

(ii) Slope or gradient is zero (0)

⇒ m = tan ∅ = 0

⇒ ∅ = 0°

⇒ either the line is x-axis or is parallel to x-axis.

(iii) Slope or gradient is negative (-ve)

⇒ m = tan ∅ < 0

⇒ ∅ lies between 0° and 180°

⇒ ∅ is an obtuse angle.

● The Straight Line

• Straight Line
• Slope of a Straight Line
• Slope of a Line through Two Given Points
• Collinearity of Three Points
• Equation of a Line Parallel to x-axis
• Equation of a Line Parallel to y-axis
• Slope-intercept Form
• Point-slope Form
• Straight line in Two-point Form
• Straight Line in Intercept Form
• Straight Line in Normal Form
• General Form into Slope-intercept Form
• General Form into Intercept Form
• General Form into Normal Form
  
• Point of Intersection of Two Lines
  
• Concurrency of Three Lines
• Angle between Two Straight Lines
• Condition of Parallelism of Lines
• Equation of a Line Parallel to a Line
• Condition of Perpendicularity of Two Lines
• Equation of a Line Perpendicular to a Line
• Identical Straight Lines
• Position of a Point Relative to a Line
• Distance of a Point from a Straight Line
• Equations of the Bisectors of the Angles between Two Straight Lines
• Bisector of the Angle which Contains the Origin
• Straight Line Formulae
• Problems on Straight Lines
• Word Problems on Straight Lines
• Problems on Slope and Intercept

11 and 12 Grade Math

From Slope of a Straight Line to HOME PAGE