Problems Based on Systems of Measuring Angles
Problems based on systems of measuring angles will help us to learn converting one measuring systems to other measuring systems. We know, the three different systems are Sexagesimal System, Centesimal System and Circular System. The examples will help us to solve various types of problems involving the three different systems of measuring angles.
Worked-out problems based on systems of measuring angles:
1. Find in sexagesimal, centesimal and circular units an internal angle of a regular Hexagon.
Solution:
We know that the sum of the internal angles of a polygon of n sides = (2n - 4) rt. angles.
Therefore, the sum of the six internal angles of a regular pentagon = (2 × 6 - 4) = 8 rt. angles.
Hence, each internal angle of the Hexagon = 8/6 rt. angles. = 4/3 rt. angles.
Therefore,
each internal angle of the regular Hexagon in sexagesimal system
measures 4/3 × 90°, (Since, 1 rt. angle = 90°) = 120°;
In centesimal system measures
= (400/3)g
= 1331/3
and in circular system measures (4/3 × π/2)c, [Since, 1 rt. angle = πc/2]
= (2π/3)c.
2. Two regular polygons have sides m and n respectively. If the number of degrees in an angle of the first is equal to the number of grades in an angle of the second, show that,
20/n - 18/m = 1.
Solution:
Sum of the internal angles of a regular polygon of m sides = (2m - 4) rt. angles.
Therefore, one angle of a regular polygon of m sides measures (2m - 4)/m rt. angles.
Similarly, one angle of a regular polygon of n sides measures (2n - 4)/n rt. angles.
By question, [(2m - 4)/m] × 90 = [(2n - 4)/n] × 100
or, (1 - 2/m) × 180 = (1 - 2/n) × 200
or, 9 - 18/m = 10 - 20/n
or, 20/n - 18/m = 1. Proved
● Measurement of Angles
- Sign of Angles
- Trigonometric Angles
- Measure of Angles in Trigonometry
- Systems of Measuring Angles
- Important Properties on Circle
- S is Equal to R Theta
- Sexagesimal, Centesimal and Circular Systems
- Convert the Systems of Measuring Angles
- Convert Circular Measure
- Convert into Radian
- Problems Based on Systems of Measuring Angles
- Length of an Arc
- Problems based on S R Theta Formula
From Problems Based on Systems of Measuring Angles to
HOME PAGE
Didn't find what you were looking for? Or want to know more information about Math Only Math. Use this Google Search to find what you need.
Recent Articles
-
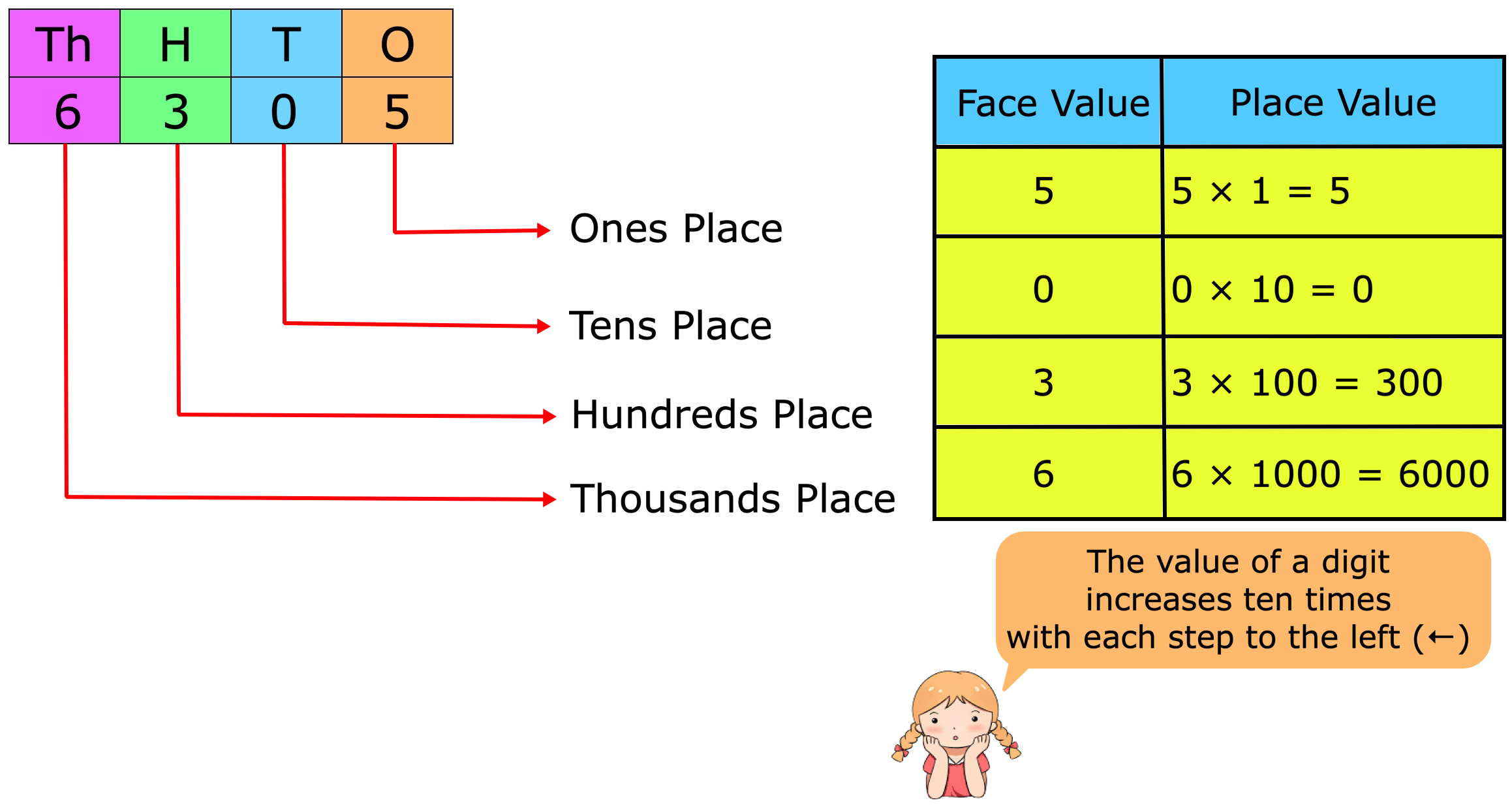
Place Value and Face Value | Place and Face Value of Larger Number
Apr 13, 25 03:12 PM
The place value of a digit in a number is the value it holds to be at the place in the number. We know about the place value and face value of a digit and we will learn about it in details. We know th… -
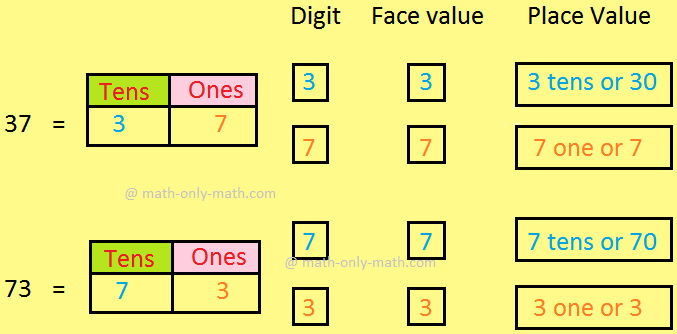
Face Value and Place Value|Difference Between Place Value & Face Value
Apr 13, 25 03:07 PM
What is the difference between face value and place value of digits? Before we proceed to face value and place value let us recall the expanded form of a number. The face value of a digit is the digit… -
Place Value and Face Value | Basic Concept on Place Value | Face Value
Apr 13, 25 02:59 PM
Learn the easiest way to understand the basic concept on place value and face value in the second grade. Suppose we write a number in figures 435 in words we write four hundred thirty five. -
Expressing Place Value and Face Value | International & Indian System
Apr 13, 25 02:35 PM
We will learn expressing place value and face value of a digit in any number in International and Indian system. Place value: We know how to find out the place value of a digit in any number. -
5th Grade Decimals | Word Problem on Decimals | Concept of Decimals
Apr 13, 25 02:16 PM
A fractional number whose denominator is 10 or multiple of 10 is called a decimal. Every decimal has two parts whole number part and decimal part. These two parts are separated by a dot or point. This…



New! Comments
Have your say about what you just read! Leave me a comment in the box below. Ask a Question or Answer a Question.