Parametric Equation of the Hyperbola

We will learn in the simplest way how to find the parametric equations of the hyperbola.

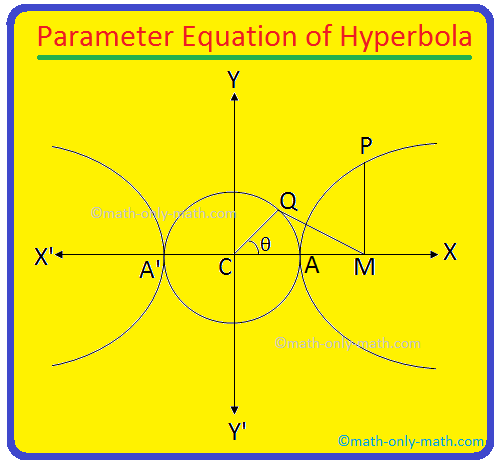
The circle described on the transverse axis of a hyperbola as diameter is called its Auxiliary Circle.

If \(\frac{x^{2}}{a^{2}}\) - \(\frac{y^{2}}{b^{2}}\) = 1 is a hyperbola, then its auxiliary circle is x\(^{2}\) + y\(^{2}\) = a\(^{2}\).

Let the equation of the hyperbola be, \(\frac{x^{2}}{a^{2}}\) - \(\frac{y^{2}}{b^{2}}\) = 1  

The transverse axis of the hyperbola \(\frac{x^{2}}{a^{2}}\) - \(\frac{y^{2}}{b^{2}}\) = 1 is AA’ and its length = 2a. Clearly, the equation of the circle described on AA’ as diameter is x\(^{2}\) + y\(^{2}\) = a\(^{2}\) (since the centre of the circle is the centre C (0, 0) of the hyperbola).

Therefore, the equation of the auxiliary circle of the hyperbola \(\frac{x^{2}}{a^{2}}\) - \(\frac{y^{2}}{b^{2}}\) = 1 is, x\(^{2}\) + y\(^{2}\) = a\(^{2}\)

Let P (x, y) be any point on the equation of the hyperbola be \(\frac{x^{2}}{a^{2}}\) -\(\frac{y^{2}}{b^{2}}\) = 1

Now from P draw PM perpendicular to the transverse axis of the hyperbola. Again take a point Q on the auxiliary circle x\(^{2}\) + y\(^{2}\) = a\(^{2}\) such that ∠CQM = 90°.

Join the point C and Q. The length of QC = a. Again, let ∠MCQ = θ. The angle ∠MCQ = θ is called the eccentric angle of the point P on the hyperbola.

Now from the right-angled  ∆CQM we get,

CQ/MC = cos θ          

or, a/MC  =   a/sec θ       

or, MC  = a sec θ

Therefore, the abscissa of P = MC = x = a sec θ

Since the point P (x, y) lies on the hyperbola \(\frac{x^{2}}{a^{2}}\) -\(\frac{y^{2}}{b^{2}}\) = 1 hence,

\(\frac{a^{2}sec^{2} θ }{a^{2}}\) - \(\frac{y^{2}}{b^{2}}\) = 1, (Since, x = a sec θ)

⇒ \(\frac{y^{2}}{b^{2}}\) = sec\(^{2}\) θ – 1

⇒ \(\frac{y^{2}}{b^{2}}\) = tan\(^{2}\) θ

⇒ y\(^{2}\) = b\(^{2}\) tan\(^{2}\) θ

⇒ y = b tan θ

Hence, the co-ordinates of P are (a sec θ, b tan θ).

Therefore, for all values of θ the point P (a sec θ, b tan θ) always lies on the hyperbola \(\frac{x^{2}}{a^{2}}\) - \(\frac{y^{2}}{b^{2}}\)  = 1  

Thus, the co-ordinates of the point having eccentric angle θ can be written as (a sec θ, b tan θ). Here (a sec θ, b tan θ) are known as the parametric co-ordinates of the point P.

The equations x = a sec θ, y = b tan θ taken together are called the parametric equations of the hyperbola \(\frac{x^{2}}{a^{2}}\) - \(\frac{y^{2}}{b^{2}}\) = 1; where θ is parameter (θ is called the eccentric angle of the point P).

Solved example to find the parametric equations of a hyperbola:

1. Find the parametric co-ordinates of the point (8, 3√3) on the hyperbola 9x\(^{2}\) - 16y\(^{2}\) = 144.

Solution:     

The given equation of the hyperbola is 9x2 - 16y2 = 144

⇒ \(\frac{x^{2}}{16}\) - \(\frac{y^{2}}{9}\) = 1

⇒ \(\frac{x^{2}}{4^{2}}\) - \(\frac{y^{2}}{3^{2}}\) = 1, which is the form of \(\frac{x^{2}}{a^{2}}\) - \(\frac{y^{2}}{b^{2}}\) = 1.  

Therefore,

a\(^{2}\) = 4\(^{2}\) 

⇒ a = 4 and   

b\(^{2}\) = 3\(^{2}\)     

⇒ b = 3.

Therefore, we can take the parametric co-ordinates of the point (8, 3√3) as (4 sec θ, 3 tan θ).

Thus we have, 4 sec θ = 8      

⇒ sec θ = 2        

⇒ θ = 60°

We know that for all values of θ the point (a sec θ, b tan θ) always lies on the hyperbola \(\frac{x^{2}}{a^{2}}\) - \(\frac{y^{2}}{b^{2}}\)  = 1  

Therefore, (a sec θ, b tan θ) are known as the parametric co-ordinates of the point.

Therefore, the parametric co-ordinates of the point (8, 3√3)   are (4 sec 60°, 3 tan 60°).

 

2. P (a sec θ, a tan θ) is a variable point on the hyperbola x\(^{2}\) - y\(^{2}\) = a\(^{2}\), and M (2a, 0) is a fixed point. Prove that the locus of the middle point of AP is a rectangular hyperbola.

Solution:        

Let (h, k) be the middle point of the line segment AM.

Therefore, h = \(\frac{a sec θ + 2a}{2}\)   

⇒ a sec θ = 2(h - a)

(a sec θ)\(^{2}\) = [2(h - a)]\(^{2}\) …………………. (i)

and k = \(\frac{a tan θ}{2}\)

⇒ a tan θ = 2k

(a tan θ)\(^{2}\) = (2k)\(^{2}\) …………………. (ii)

Now form (i) - (ii), we get,

(a sec θ)\(^{2}\) - (a tan θ)\(^{2}\) = [2(h - a)]\(^{2}\) - (2k)\(^{2}\)

⇒ a\(^{2}\)(sec\(^{2}\) θ - tan\(^{2}\) θ) = 4(h - a)\(^{2}\) - 4k\(^{2}\)

⇒ (h - a)\(^{2}\) - k\(^{2}\) = \(\frac{a^{2}}{4}\).

Therefore, the equation to the locus of (h, k) is (x - a)\(^{2}\) - y\(^{2}\) = \(\frac{a^{2}}{4}\), which is the equation of a rectangular hyperbola.

● The Hyperbola

• Definition of Hyperbola
• Standard Equation of an Hyperbola
  
• Vertex of the Hyperbola
• Centre of the Hyperbola
• Transverse and Conjugate Axis of the Hyperbola
• Two Foci and Two Directrices of the Hyperbola
• Latus Rectum of the Hyperbola
• Position of a Point with Respect to the Hyperbola
• Conjugate Hyperbola
• Rectangular Hyperbola
• Parametric Equation of the Hyperbola
• Hyperbola Formulae
• Problems on Hyperbola

11 and 12 Grade Math 

From Parametric Equation of the Hyperbola to HOME PAGE