Modulus of a Complex Number
Definition of Modulus of a Complex Number:
Let z = x + iy where x and y are real and i = √-1. Then the non negative square root of (x2+ y 2) is called the modulus or absolute value of z (or x + iy).
Modulus of a complex number z = x + iy, denoted by mod(z) or |z| or |x + iy|, is defined as |z|[or mod z or |x + iy|] = + √x2+y2 ,where a = Re(z), b = Im(z)
i.e., + √Re(z)2+Im(z)2
Sometimes, |z| is called absolute value of z. Clearly, |z| ≥ 0 for all zϵ C.
For example:
(i) If z = 6 + 8i then |z| = √62+82 = √100 = 10.
(ii) If z = -6 + 8i then |z| = √(−6)2+82 = √100 = 10.
(iii) If z = 6 - 8i then |z| = √62+(−8)2 =
√100 = 10.
(iv) If z = √2 - 3i then |z| = √(√2)2+(−3)2 = √11.
(v) If z = -√2 - 3i then |z| = √(−√2)2+(−3)2 = √11.
(vi) If z = -5 + 4i then |z| = √(−5)2+42 = √41
(vii) If z = 3 - √7i then |z| = √32+(−√7)2 =√9+7 = √16 = 4.
Note: (i) If z = x + iy and x = y = 0 then |z| = 0.
(ii) For any complex number z we have, |z| = |ˉz| = |-z|.
Properties of modulus of a complex number:
If z, z1 and z2 are complex numbers, then
(i) |-z| = |z|
Proof:
Let z = x + iy, then –z = -x – iy.
Therefore, |-z| = √(−x)2+(−y)2 = √x2+y2 = |z|
(ii) |z| = 0 if and only if z = 0
Proof:
Let z = x + iy, then |z| = √x2+y2.
Now |z| = 0 if and only if √x2+y2 = 0
⇒ if only if x2 + y2 = 0 i.e., a2 = 0and b2 = 0
⇒ if only if x = 0 and y = 0 i.e., z = 0 + i0
⇒ if only if z = 0.
(iii) |z1z2| = |z1||z2|
Proof:
Let z1 = j + ik and z2 = l + im, then
z1z2 =(jl - km) + i(jm + kl)
Therefore, |z1z2| = √(jl−km)2+(jm+kl)2
= √j2l2+k2m2–2jklm+j2m2+k2l2+2jklm
= √(j2+k2)(l2+m2
= √j2+k2 √l2+m2, [Since, j2 + k2 ≥0, l2 + m2 ≥0]
= |z1||z2|.
(iv) |z1z2| = |z1||z2|, provided z2 ≠ 0.
Proof:
According to the problem, z2 ≠ 0 ⇒ |z2| ≠ 0
Let z1z2 = z3
⇒ z1 = z2z3
⇒ |z1| = |z2z3|
⇒|z1| = |z2||z3|, [Since we know that |z1z2| = |z1||z2|]
⇒ |z1z2 = |z3|
⇒ |z1||z2| = |z1z2|, [Since, z3 = z1z2]
11 and 12 Grade Math
From Modulus of a Complex Number to HOME PAGE
Didn't find what you were looking for? Or want to know more information about Math Only Math. Use this Google Search to find what you need.
Recent Articles
-
Divisible by 10 | Test of Divisibility by 10 Video | Rules | Examples
Mar 29, 25 03:06 PM
Divisible by 10 is discussed below. A number is divisible by 10 if it has zero (0) in its units place. Consider the following numbers which are divisible by 10, using the test of divisibility by 10: -
Divisible by 9 | Test of Divisibility by 9 | Rules | Video | Examples
Mar 29, 25 02:55 PM
A number is divisible by 9, if the sum is a multiple of 9 or if the sum of its digits is divisible by 9. Consider the following numbers which are divisible by 9, using the test of divisibility by 9: -
Divisible by 6 | Rules for Test of Divisibility by 6 Video | Examples
Mar 29, 25 02:48 PM
Divisible by 6 is discussed below: A number is divisible by 6 if it is divisible by 2 and 3 both. Consider the following numbers which are divisible by 6, using the test of divisibility by 6: 42 -
Divisible by 5 | Rules for Test of divisibility by 5 | Video |Examples
Mar 29, 25 02:43 PM
Divisible by 5 is discussed below: A number is divisible by 5 if its units place is 0 or 5. Consider the following numbers which are divisible by 5, using the test of divisibility by -
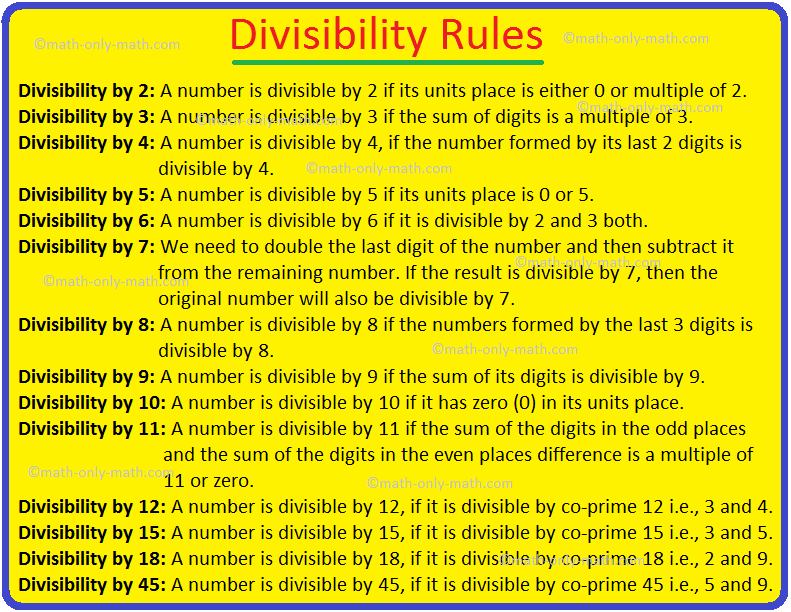
Divisibility Rules From 2 to 18 | Math Divisibility Test | Videos |
Mar 29, 25 02:17 PM
To find out factors of larger numbers quickly, we perform divisibility test. There are certain rules to check divisibility of numbers. Divisibility tests of a given number by any of the number 2, 3, 4…





New! Comments
Have your say about what you just read! Leave me a comment in the box below. Ask a Question or Answer a Question.