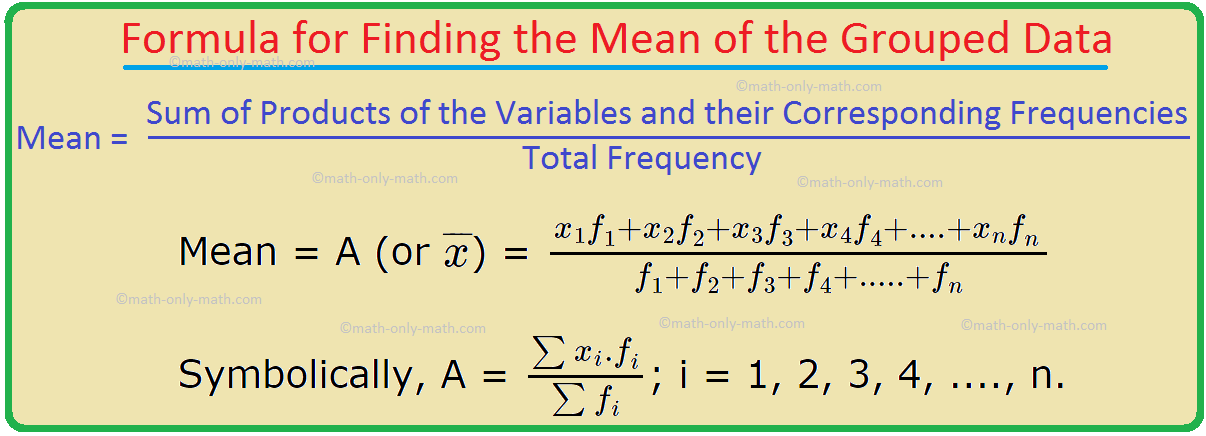
Mean of Grouped Data

If the values of the variable (i.e., observations or variates) be x\(_{1}\), x\(_{2}\), x\(_{3}\), x\(_{4}\), ....., x\(_{n}\) and their corresponding frequencies are f\(_{1}\), f\(_{2}\), f\(_{3}\), f\(_{4}\), ....., f\(_{n}\) then the mean of the data is given by

Mean = A (or \(\overline{x}\)) = \(\frac{x_{1}f_{1} + x_{2}f_{2} + x_{3}f_{3}  + x_{4}f_{4} + .... + x_{n}f_{n}}{ f_{1} +  f_{2} + f_{3} + f_{4} + ..... + f_{n}}\)

Symbolically, A = \(\frac{\sum{x_{i}. f_{i}}}{\sum f_{i}}\); i = 1, 2, 3, 4, ...., n.

In words,

Mean = \(\frac{\textbf{Sum of products of the Variables and their corresponding Frequencies}}{\textbf{Total Frequency}}\)

This is the formula for finding the mean of the grouped data by direct method. 

For Example:

The number of Mobile sold is given in the table below. Find the mean of the number of Mobile sold.

Solution:

Here, x\(_{1}\) = 2, x\(_{2}\) = 5, x\(_{3}\) = 6, x\(_{4}\) = 10, x\(_{5}\) = 12.

f\(_{1}\) = 6, f\(_{2}\) = 10, f\(_{3}\) = 8, f\(_{4}\) = 1, f\(_{5}\) = 5.

Therefore, mean = \(\frac{x_{1}f_{1} + x_{2}f_{2} + x_{3}f_{3} + x_{4}f_{4} + x_{5}f_{5}}{f_{1} + f_{2} + f_{3} + f_{4} + f_{5}}\)

                         = \(\frac{2 × 6 + 5 × 10 + 6 × 8 + 10 × 1 + 12 × 5}{6 + 10 + 8 + 1 + 5}\)

                         = \(\frac{12 + 50 + 48  10 + 60}{30}\)

                         = \(\frac{180}{30}\)

                         = 6.

Therefore, mean number of Mobile sold is 6.

Short-cut method for finding the mean of grouped data:

We know that the direct method of finding mean for grouped data gives

mean A =  \(\frac{\sum{x_{i}. f_{i}}}{\sum f_{i}}\)

where x\(_{1}\), x\(_{2}\), x\(_{3}\), x\(_{4}\), ....., x\(_{n}\) are variates and f\(_{1}\), f\(_{2}\), f\(_{3}\), f\(_{4}\), ....., f\(_{n}\)  are their corresponding frequencies.

Let a = a number taken as assumed mean from which the diviation of the variate is d_i = x_i - a.

Then, A =\(\frac{\sum{(a + d_{i})f_{i}}}{\sum f_{i}}\)

            = \(\frac{\sum{af_{i}} + \sum{d_{i}f_{i}}}{\sum f_{i}}\)

            =  \(\frac{a\sum{f_{i}} + \sum{d_{i}f_{i}}}{\sum f_{i}}\)

            = a + \(\frac{\sum{d_{i}f_{i}}}{\sum f_{i}}\)

Therefore, A = a + \(\frac{\sum{d_{i}f_{i}}}{\sum f_{i}}\), where d_i = x_i - a.

For Example: 

Find the mean of the following distribution using the short-cut method.

Solution:

Putting the calculated values in a tabular form, we have the following.

Therefore, mean A = a + \(\frac{\sum{d_{i}f_{i}}}{\sum f_{i}}\)

                            = 60 + \(\frac{200}{101}\)

                            = 61\(\frac{99}{101}\)

                            = 61.98.

Solved Examples on Mean of Grouped Data or Mean of the Arrayed Data:

1. A class has 20 students whose ages (in years) are as follows.

14, 13, 14, 15, 12, 13, 13, 14, 15, 12, 15, 14, 12, 16, 13, 14, 14, 15, 16, 12

Find the mean ago of the students of the class.

Solution:

In the data, only five different numbers appear respectively. So, we write the frequencies of the variates as below.

Therefore, mean A = \(\frac{x_{1}f_{1} + x_{2}f_{2} + x_{3}f_{3}  + x_{4}f_{4} + x_{5}f_{5}}{ f_{1} +  f_{2} + f_{3} + f_{4} + f_{5}}\)

= \(\frac{12 × 4 + 13 × 4 + 14 × 6 + 15 × 4 + 16 × 2}{4 + 4 + 6 + 4 + 2}\)

= \(\frac{48 + 52 + 84 + 60 + 32}{20}\)

= \(\frac{276}{20}\)

= 13.8

Therefore, the mean age of the students of the class = 13.8 years.

2. The weights (in kg) of 30 boxes are as given below.

40, 41, 41, 42, 44, 47, 49, 50, 48, 41, 43, 45, 46, 47, 49, 41, 40, 43, 46, 47, 48, 48, 50, 50, 40, 44, 44, 47, 48, 50.

Find the mean weight of the boxes by preparing a frequency table of the arrayed data.

Solution:

The frequency table for the given data is 

By formula, mean = \(\frac{\sum{x_{i}f_{i}}}{\sum f_{i}}\)

                           = \(\frac{1359}{30}\)

                           = 45.3.

Therefore, the mean weight of the boxes = 45.3 kg.

3. Four variates are 2, 4, 6 and 8. The frequencies of the first three variates are 3, 2 and 1 respectively. If the mean of the variates is 4 then find the frequency of the fourth variate. 

Solution:

Let the frequency of the fourth variate (8) be f. Then, 

mean A = \(\frac{x_{1}f_{1} + x_{2}f_{2} + x_{3}f_{3}  + x_{4}f_{4}}{ f_{1} +  f_{2} + f_{3} + f_{4}}\)

⟹ 4 = \(\frac{2 × 3 + 4 × 2 + 6 × 1 + 8 × f}{3 + 2 + 1 + f}\)

⟹ 4 = \(\frac{6 + 8 + 6 + 8f}{6 + f}\)

⟹ 24 + 4f = 20 + 8f

⟹ 4f = 4

⟹ f = 1

Therefore, the frequency of 8 is 1.

4. Find the mean of the following data.

Solution:

The frequency table and calculations involved in finding the mean are given below.

Therefore, mean = \(\frac{\sum{x_{i}f_{i}}}{\sum f_{i}}\)

                         = \(\frac{46}{15}\)

                         = 3.07.

5. Find the mean mark from the following frequency table by using the short-cut method.

Solution:

Taking the assumed mean a = 40, the calculations will be as follows.

Therefore, mean = a + \(\frac{\sum{d_{i}f_{i}}}{\sum f_{i}}\)

                         = 40 + \(\frac{-460}{100}\)

                         = 40 - 4.6

                         = 35.4.

Therefore, the mean mark is 35.4.

9th Grade Math

From Mean of Grouped Data to HOME PAGE