Geometric Progression
We will discuss here about the Geometric Progression along with examples.
A sequence of numbers is said to be Geometric Progression if the ratio of any term and its preceding term is always a constant quantity.
Definition of Geometric Progression:
A sequence of non-zero number is said to be in Geometric Progression (abbreviated as G.P.) if each term, after the first, is obtained by multiplying the preceding term by a constant quantity (positive or negative).
The constant ratio is said to be the common ratio of the Geometric Progression and is denoted by dividing any term by that which immediately precedes it.
In other words, the sequence {a1, a2, a3, a4, ..................., an, ................. } is said to be in Geometric Progression, if an+1an = constant for all n ϵ N i.e., for all integral values of a, the ratio an+1an is constant.
Examples on Geometric Progression
1. The sequence 3, 15, 75, 375, 1875, .................... is a Geometric Progression, because 155 = 7515 = 37575 = 1875375 = .................. = 5, which is constant.
Clearly, this sequence is a Geometric Progression with first term 3 and common ratio 5.
2. The sequence 13, -12, 34, -98, is a Geometric Progression with first term 13 and common ratio −1213 = -32
3. The sequence of numbers {4, 12, 36, 108, 324, ........... } forms a Geometric Progression whose common ratio is 3, because,
Second term (12) = 3 × First term (4),
Third term (36) = 3 × Second term (12),
Fourth term (108) = 3 × Third term (36),
Fifth term (324) = 3 × Fourth term (108) and so on.
In other words,
Secondterm(12)Firstterm(4) = Thirdterm(36)Secondterm(12) = Fourthterm(108)Thirdterm(36) = Fifthterm(324)Fourthterm(108) = ................. = 3 (a constant)
Solved example on Geometric Progression
Show that the sequence given by an = 3(2n), for all n ϵ N, is a Geometric Progression. Also, find its common ratio.
Solution:
The given sequence is an = 3(2n)
Now putting n = n +1 in the given sequence we get,
an+1 = 3(2n+1)
Now, an+1an = 3(2n+1)3(2n) = 2
Therefore, we clearly see that for all integral values of n, the an+1an = 2 (constant). Thus, the given sequence is an Geometric Progression with common ratio 2.
Geometric Series:
If a1, a2, a3, a4, ..............., an, .......... is a Geometric Progression, then the expression a1 + a2 + a3 + ......... + an + .................... is called a geometric series.
Notes:
(i) The geometric series is finite according as the corresponding Geometric Progression consists of finite number of terms.
(ii) The geometric series is infinite according as the corresponding Geometric Progression consists of infinite number of terms.
● Geometric Progression
- Definition of Geometric Progression
- General Form and General Term of a Geometric Progression
- Sum of n terms of a Geometric Progression
- Definition of Geometric Mean
- Position of a term in a Geometric Progression
- Selection of Terms in Geometric Progression
- Sum of an infinite Geometric Progression
- Geometric Progression Formulae
- Properties of Geometric Progression
- Relation between Arithmetic Means and Geometric Means
- Problems on Geometric Progression
11 and 12 Grade Math
From Geometric Progression to HOME PAGE
Didn't find what you were looking for? Or want to know more information about Math Only Math. Use this Google Search to find what you need.
Recent Articles
-
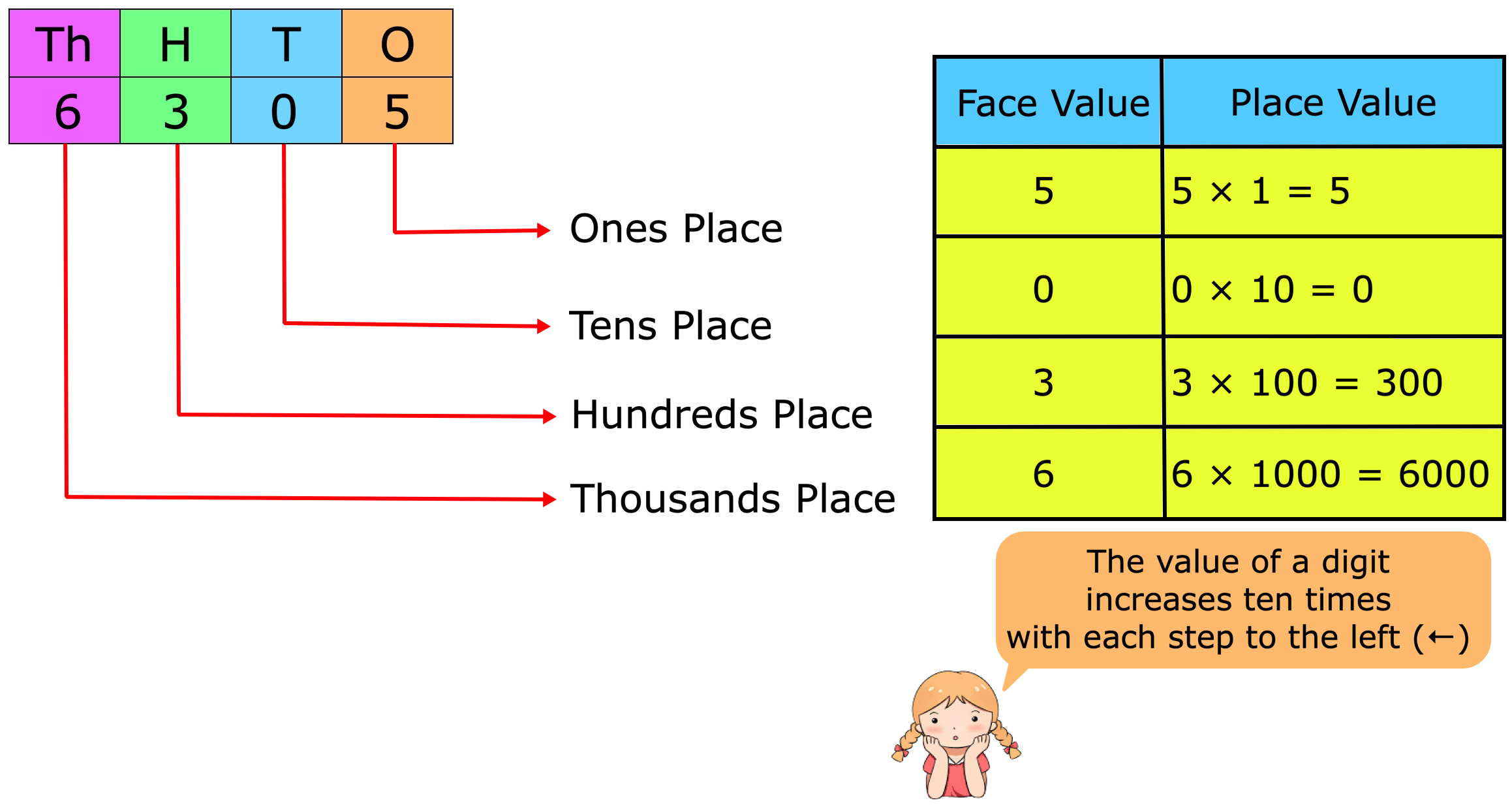
Place Value and Face Value | Place and Face Value of Larger Number
Apr 13, 25 03:12 PM
The place value of a digit in a number is the value it holds to be at the place in the number. We know about the place value and face value of a digit and we will learn about it in details. We know th… -
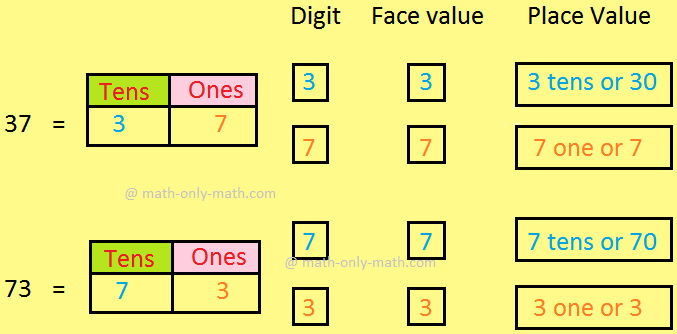
Face Value and Place Value|Difference Between Place Value & Face Value
Apr 13, 25 03:07 PM
What is the difference between face value and place value of digits? Before we proceed to face value and place value let us recall the expanded form of a number. The face value of a digit is the digit… -
Place Value and Face Value | Basic Concept on Place Value | Face Value
Apr 13, 25 02:59 PM
Learn the easiest way to understand the basic concept on place value and face value in the second grade. Suppose we write a number in figures 435 in words we write four hundred thirty five. -
Expressing Place Value and Face Value | International & Indian System
Apr 13, 25 02:35 PM
We will learn expressing place value and face value of a digit in any number in International and Indian system. Place value: We know how to find out the place value of a digit in any number. -
5th Grade Decimals | Word Problem on Decimals | Concept of Decimals
Apr 13, 25 02:16 PM
A fractional number whose denominator is 10 or multiple of 10 is called a decimal. Every decimal has two parts whole number part and decimal part. These two parts are separated by a dot or point. This…



New! Comments
Have your say about what you just read! Leave me a comment in the box below. Ask a Question or Answer a Question.