Conditions of Collinearity of Three Points

We will discuss here how to prove the conditions of collinearity of three points.

Definition of Collinear Points:

Three or more points in a plane are said to be collinear if they all he on the same line.

Working Rules to Draw Collinear Points:

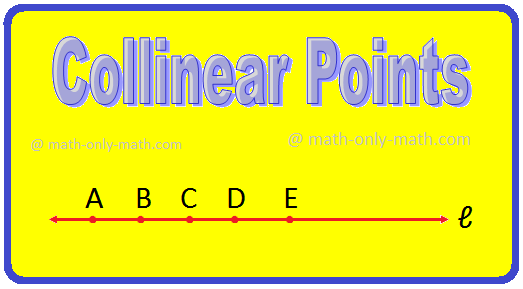
Step I: Draw a straight line 'ℓ'.

Step II: Mark points A, B, C, D, E on the straight line 'ℓ'.

Thus, we have drawn the collinear points A, B, C, D and E on the line 'ℓ'.

NOTE: If the points do not lie on the line, they are called non-collinear points.

Three points A, B and C are said to be collinear if they lie on the same straight line.

There points A, B and C will be collinear if AB + BC = AC as is clear from the above figure.

In general, three points A, B and C are collinear if the sum of the lengths of any two line segments among AB, BC and CA is equal to the length of the remaining line segment, that is,

either AB + BC = AC or AC + CB = AB or BA + AC = BC.

In other words,

There points A, B and C are collinear iff:

(i) AB + BC = AC i.e.,

Or, (ii) AB + AC = BC i.e. ,

Or, AC + BC = AB i.e.,

Solved examples to prove the collinearity of three points:

1. Prove that the points A (1, 1), B (-2, 7) and (3, -3) are collinear.

Solution:

Let A (1, 1), B (-2, 7) and C (3, -3) be the given points. Then,

AB = \(\sqrt{(-2 - 1)^{2} + (7 - 1)^{2}}\) = \(\sqrt{(-3)^{2} + 6^{2}}\) = \(\sqrt{9 + 36}\) = \(\sqrt{45}\) = 3\(\sqrt{5}\) units.

BC = \(\sqrt{(3 + 2)^{2} + (-3 - 7)^{2}}\) = \(\sqrt{5^{2} + (-10)^{2}}\) = \(\sqrt{25 + 100}\) = \(\sqrt{125}\) = 5\(\sqrt{5}\) units.

AC = \(\sqrt{(3 - 1)^{2} + (-3 - 1)^{2}}\) = \(\sqrt{2^{2} + (-4)^{2}}\) = \(\sqrt{4 + 16}\) = \(\sqrt{20}\) = 2\(\sqrt{5}\) units.

Therefore, AB + AC = 3\(\sqrt{5}\) + 2\(\sqrt{5}\) units = 5\(\sqrt{5}\) = BC

Thus, AB + AC = BC

Hence, the given points A, B, C are collinear.

2. Use the distance formula to show the points (1, -1), (6, 4) and (4, 2) are collinear.

Solution:

Let the points be A (1, -1), B (6, 4) and C (4, 2). Then,

AB = \(\sqrt{(6 - 1)^{2} + (4 + 1)^{2}}\) = \(\sqrt{5^{2} + 5^{2}}\) = \(\sqrt{25 + 25}\) = \(\sqrt{50}\) = 5\(\sqrt{2}\)

BC = \(\sqrt{(4 - 6)^{2} + (2 - 4)^{2}}\) = \(\sqrt{(-2)^{2} + (-2)^{2}}\) = \(\sqrt{4 + 4}\) = \(\sqrt{8}\) = 2\(\sqrt{2}\)

and

AC = \(\sqrt{(4 - 1)^{2} + (2 + 1)^{2}}\) = \(\sqrt{3^{2} + 3^{2}}\) = \(\sqrt{9 + 9}\) = \(\sqrt{18}\) = 3\(\sqrt{2}\)

⟹ BC + AC = 2\(\sqrt{2}\) + 3\(\sqrt{2}\) = 5\(\sqrt{2}\) = AB

So, the points A, B and C are collinear with C lying between A and B.

3. Use the distance formula to show the points (2, 3), (8, 11) and (-1, -1) are collinear.

Solution:

Let the points be A (2, 3), B (8, 11) and C (-1, -1). Then,

AB = \(\sqrt{(2 - 8)^{2} + (3 - 11)^{2}}\) = \(\sqrt{6^{2} + (-8)^{2}}\) = \(\sqrt{36 + 64}\) = \(\sqrt{100}\) = 10

BC = \(\sqrt{(8 - (-1))^{2} + (11 - (-1))^{2}}\) = \(\sqrt{9^{2} + 12^{2}}\) = \(\sqrt{81 + 144}\) = \(\sqrt{225}\) = 15

and

CA = \(\sqrt{((-1) - 2)^{2} + ((-1) + 3)^{2}}\) = \(\sqrt{(-3)^{2} + (-4)^{2}}\) = \(\sqrt{9 + 16}\) = \(\sqrt{25}\) = 5

⟹ AB + CA = 10 + 5 = 15 = BC

Hence, the given points A, B, C are collinear.

● Distance and Section Formulae

• Distance Formula
• Distance Properties in some Geometrical Figures
• Conditions of Collinearity of Three Points
• Problems on Distance Formula
• Distance of a Point from the Origin
• Distance Formula in Geometry
• Section Formula
• Midpoint Formula
• Centroid of a Triangle
• Worksheet on Distance Formula
• Worksheet on Collinearity of Three Points
• Worksheet on Finding the Centroid of a Triangle
• Worksheet on Section Formula

10th Grade Math

From Conditions of Collinearity of Three Points to HOME PAGE

New! Comments

Have your say about what you just read! Leave me a comment in the box below. Ask a Question or Answer a Question.

Share this page: What’s this?

FacebookXPinterestWhatsAppReddit

ShareFacebookXPinterestWhatsAppReddit