Circle
A circle is the set of all those point in a plane whose distance from a fixed point remains constant.
The fixed point is called the centre of the circle and the constant distance is known as the radius of the circle.
Full moon is the example of a circle.
Interior and Exterior of a Circle:
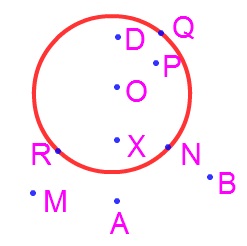
A Circle has an interior as well as an exterior region as shown in the below figure.
Here the points A, B and M lie in the exterior of the circle.
The points D, P and X lies in the interior of the circle.
The point R, Q, N lie on the circle.
The centre O of the circle always lies in the interior of the circle.
Consider a circle with centre O and radius r.
(i) The part of the plane consisting of the point A, for which OA < r, is called the interior of the circle.
(ii) The part of the plane consisting of the point B, for which OB = r, is the circle itself.
(iii) The part of the plane consisting of the point C, for which OC > r, is called the exterior of the circle.
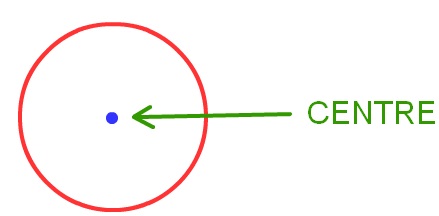
1. What is the centre of the circle?
Solution:
The point P at which we place the needle end of the compass and move the pencil around is the center of the circle.
The centre of a circle lies in its interior.
2. What is the circumference of the circle?
Solution:
The length of the boundary of the circle is its circumference.
In other words, it is the perimeter of the circle.
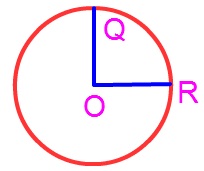
3. What is the radius of the circle?
Solution:
The line segment joining the centre to any point on the circle is called the radius of the circle.
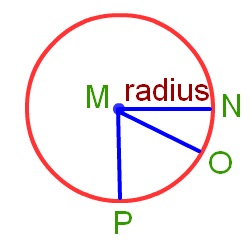
Take any point N on the circle and joint it with the centre M. The line segment MN is the radius of the circle.
Note:
MN = MO = MP → (Radii)
All the radii of a circle are equal in length. We can draw as many radii as we want. MN = MO = MP → (Radii)
All the radii of a circle are equal in length. We can draw as many radii as we want.
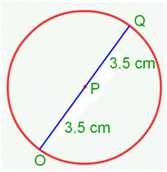
4. What is the diameter of the circle?
Solution:
Let us produce the radius PQ to meet another point O on the circle. We get a line segment OQ with its end points O & Q on the circle. It passes through the centre P.
Such a line segment is called a diameter.
The length of a diameter of a circle is twice the length of the radius of the circle.
OP = 3.5 cm
PQ = 3.5 cm
OQ = 3.5 cm + 3.5 cm
Therefore, OQ = 7.0 cm
5. What is the chord of the circle?
Solution:
The line segment joining any two points on the circle is the chord of the circle. The end points A and B of line segment AB lie on the circle.
So, AB is the chord of the circle.
Chords of a circle may or may not be equal in length. Diameter of a circle is the longest chord.
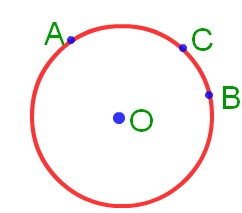
6. What is the arc of the circle?
Solution:
Any part of a circle is called an arc of the circle. An arc is usually named by 3 points.
ACB is an arc of the given circle.
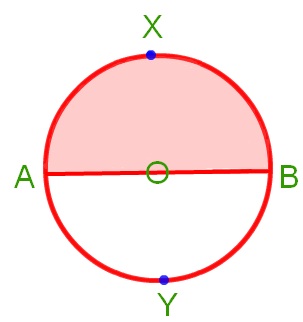
7. What is semi circle?
Solution:
The end points of a diameter of a circle divide the circle into two parts; each part is called a semi-circular region.
AXB and AYB are two semi circles.
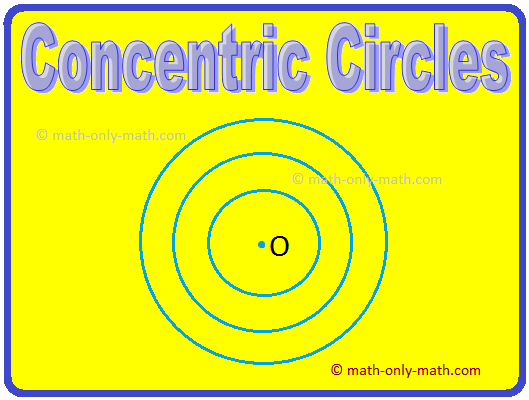
8. What are Concentric Circles?
Two or more circles with the same centre are called concentric circles.
In the above figure, three concentric circles with same centre O are drawn.
Math Only Math is based on the premise that children do not make a distinction between play and work and learn best when learning becomes play and play becomes learning.
However, suggestions for further improvement, from all quarters would be greatly appreciated.
● Circle
Relation between Diameter Radius and Circumference.
5th Grade Geometry Page
5th Grade Math Problems
From Circle to HOME PAGE
Didn't find what you were looking for? Or want to know more information about Math Only Math. Use this Google Search to find what you need.
Recent Articles
-
Counting Numbers from 1 to 50 | Match the Number | Missing Numbers
Apr 04, 25 03:46 PM
In counting numbers from 1 to 50, recognize the numbers, count and then join the numbers in the correct number order. Here we mainly need eye-hand coordination to draw the picture and maintain the num -
Counting Eleven to Twenty with Numbers and Words |Numbers from 11 - 20
Apr 04, 25 03:21 PM
Counting eleven to twenty with numbers and words are explained below. One ten and one more is eleven. Eleven comes after ten. One ten and two more is twelve. Twelve comes after eleven. -
5th Grade BODMAS Rule Worksheet | PEMDAS | Order of operations|Answers
Apr 03, 25 03:11 PM
In 5th Grade BODMAS Rule Worksheet you will get different types of problems on mathematical expressions involving different operations, mathematical expression with 'brackets' and 'of' and simplifying… -
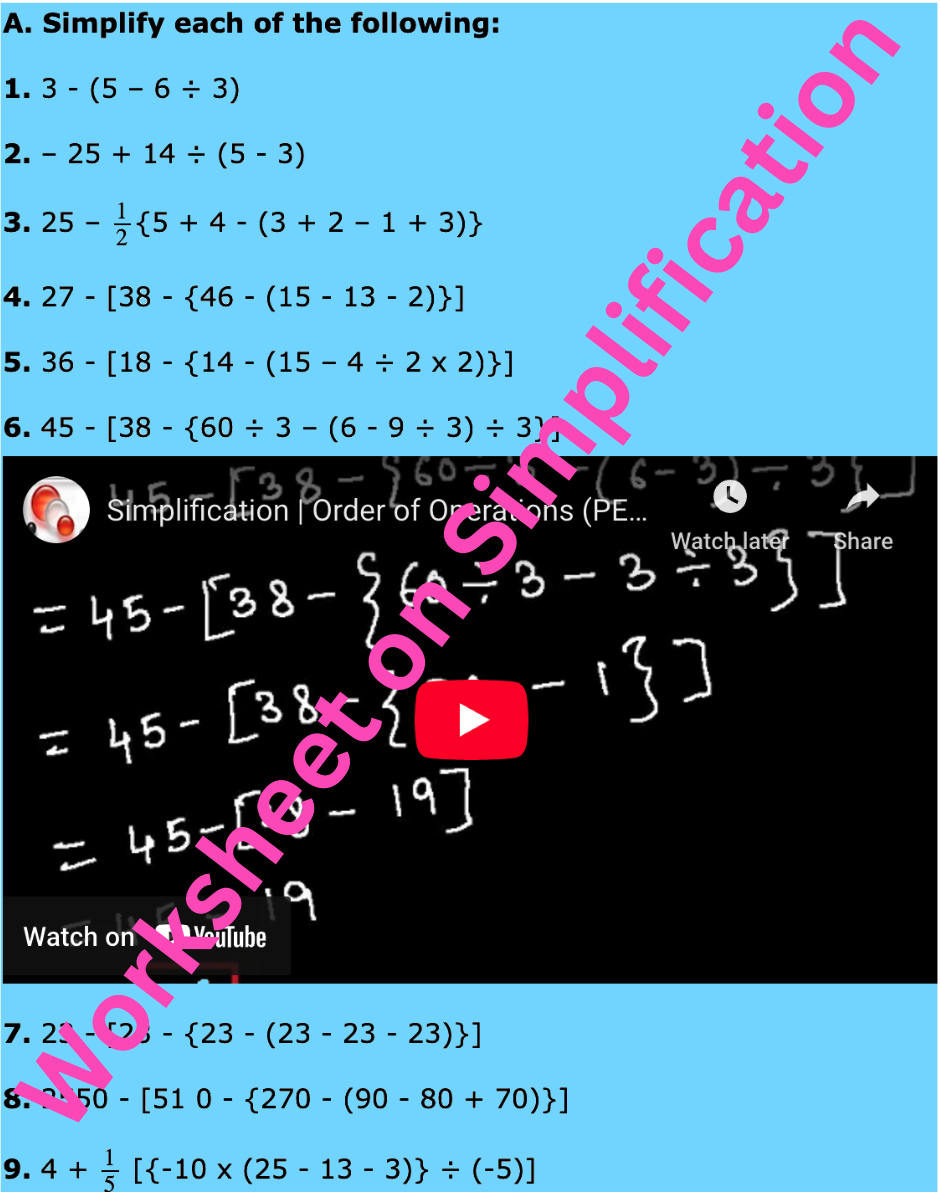
Worksheet on Simplification | Simplify Expressions | BODMAS Questions
Apr 03, 25 02:58 PM
In worksheet on simplification, the questions are based in order to simplify expressions involving more than one bracket by using the steps of removal of brackets. This exercise sheet -
Divisible by 2 Video |Test of Divisibility by 2 Trick| Rules| Examples
Apr 03, 25 10:25 AM
A number is divisible by 2 if the digit at unit place is either 0 or multiple of 2. So a number is divisible by 2 if digit at its units place is 0, 2, 4, 6 or 8.






New! Comments
Have your say about what you just read! Leave me a comment in the box below. Ask a Question or Answer a Question.