Processing math: 100%

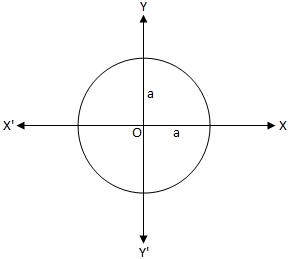
Centre of the Circle Coincides with the Origin

We will learn how to form the equation of a circle when the centre of the circle coincides with the origin.

The equation of a circle with centre at (h, k) and radius equal to a, is (x - h)2 + (y - k)2 = a2.

When the centre of the circle coincides with the origin i.e., h = k = 0.

Centre of the Circle Coincides with the OriginCentre of the Circle Coincides with the Origin

Then the equation (x - h)2 + (y - k)2 = a2 becomes x2 + y2 = a2

Solved examples on the central form of the equation of a circle whose centre coincides with the origin:

1. Find the equation of the circle whose centre coincides with the origin and radius is √5 units.

Solution:

The equation of the circle whose centre coincides with the origin and radius is √5 units is x2 + y2 = (√5)2

⇒ x2 + y2 = 5

⇒ x2 + y2 - 5 = 0.


2. Find the equation of the circle whose centre coincides with the origin and radius is 10 units.

Solution:

The equation of the circle whose centre coincides with the origin and radius is 10 units is x2 + y2 = (10)2

⇒ x2 + y2 = 100

⇒ x2 + y2 - 100 = 0.

 

3. Find the equation of the circle whose centre coincides with the origin and radius is 2√3 units.

Solution:

The equation of the circle whose centre coincides with the origin and radius is 2√3 units is x2 + y2 = (2√3)2

⇒ x2 + y2 = 12

⇒ x2 + y2 - 12 = 0.


4. Find the equation of the circle whose centre coincides with the origin and radius is 13 units.

Solution:

The equation of the circle whose centre coincides with the origin and radius is 13 units is x2 + y2 = (13)2

⇒ x2 + y2 = 169

⇒ x2 + y2 - 169 = 0


5. Find the equation of the circle whose centre coincides with the origin and radius is 1 unit.

Solution:

The equation of the circle whose centre coincides with the origin and radius is 1 unit is x2 + y2 = (1)2

⇒ x2 + y2 = 1

⇒ x2 + y2 - 1 = 0

● The Circle




11 and 12 Grade Math 

From Centre of the Circle Coincides with the Origin to HOME PAGE




Didn't find what you were looking for? Or want to know more information about Math Only Math. Use this Google Search to find what you need.



New! Comments

Have your say about what you just read! Leave me a comment in the box below. Ask a Question or Answer a Question.




Share this page: What’s this?

Recent Articles

RSS

  1. Math Place Value | Place Value | Place Value Chart | Ones and Tens

    Apr 16, 25 03:10 PM

    0, 1, 2, 3, 4, 5, 6, 7, 8 and 9 are one-digit numbers. Numbers from 10 to 99 are two-digit numbers. Let us look at the digit 6 in the number 64. It is in the tens place of the number. 6 tens = 60 So…

    Read More

  2. Place Value and Face Value | Place and Face Value of Larger Number

    Apr 16, 25 02:55 PM

    Place Value of 3-Digit Numbers
    The place value of a digit in a number is the value it holds to be at the place in the number. We know about the place value and face value of a digit and we will learn about it in details. We know th…

    Read More

  3. Face Value and Place Value|Difference Between Place Value & Face Value

    Apr 16, 25 02:50 PM

    Place Value and Face Value
    What is the difference between face value and place value of digits? Before we proceed to face value and place value let us recall the expanded form of a number. The face value of a digit is the digit…

    Read More

  4. Place Value and Face Value | Basic Concept on Place Value | Face Value

    Apr 16, 25 02:44 PM

    Face Value and Place Value of a Number
    Learn the easiest way to understand the basic concept on place value and face value in the second grade. Suppose we write a number in figures 435 in words we write four hundred thirty five.

    Read More

  5. Greater or Less than and Equal to | Bigger Number | Smaller Number | >

    Apr 16, 25 02:31 PM

    Greater or less than and equal to
    In math, greater or less than and equal to help the kids to understand how one number is different from the other number. Whether one number is bigger or smaller than the other number or both the numb

    Read More