Equation of a Circle
We will learn how to find the equation of a circle whose centre and radius are given.
Case I: If the centre and radius of a circle be given, we can determine its equation:
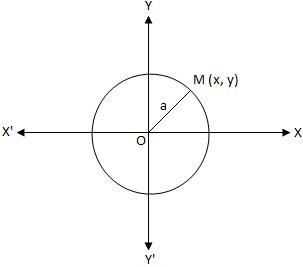
To find the equation of the circle whose centre is at the origin O and radius r units:
Let M (x, y) be any point on the circumference of the required circle.
Therefore, the locus of the moving point M = OM = radius of the circle = r
⇒ OM\(^{2}\) = r\(^{2}\)
⇒ x\(^{2}\) + y\(^{2}\) = r\(^{2}\), which is the required equation of the circle.
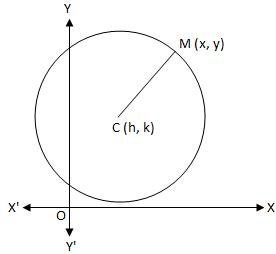
Case II: To find the equation of the circle whose centre is at C (h, k) and radius r units:
Let M (x, y) be any point on the circumference of the requited circle. Therefore, the locus of the moving point M = CM = radius of the circle = r
⇒ CM\(^{2}\) = r\(^{2}\)
⇒ (x - h)\(^{2}\) + (y - k)\(^{2}\) = r\(^{2}\), which is the required equation of the circle.
Note:
(i) The above equation is known as the central from of the equation of a circle.
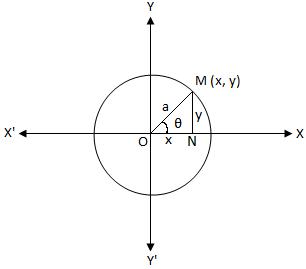
(ii) Referred to O as pole and OX as initial line of polar co-ordinate system, if the polar co-ordinates of M be (r, θ) then we shall have,
r = OM = radius of the circle = a and ∠MOX = θ.
Then, from the above figure we get,
x = ON = a cos θ and y = MN = a sin θ
Here, x = a cos θ and y = a sin θ represent the parametric equations of the circle x\(^{2}\) + y\(^{2}\) = r\(^{2}\).
Solved examples to find the equation of a circle:
1. Find the equation of a circle whose centre is (4, 7) and radius 5.
Solution:
The equation of the required circle is
(x - 4)\(^{2}\) + (y - 7)\(^{2}\) = 5\(^{2}\)
⇒ x\(^{2}\) - 16x + 16 + y\(^{2}\) - 14y + 49 = 25
⇒ x\(^{2}\) + y\(^{2}\) - 16x - 14y + 40 = 0
2. Find the equation of a circle whose radius is 13 and the centre is at the origin.
Solution:
The equation of the required circle is
x\(^{2}\) + y\(^{2}\) = 13\(^{2}\)
⇒ x\(^{2}\) + y\(^{2}\) = 169
● The Circle
- Definition of Circle
- Equation of a Circle
- General Form of the Equation of a Circle
- General Equation of Second Degree Represents a Circle
- Centre of the Circle Coincides with the Origin
- Circle Passes through the Origin
- Circle Touches x-axis
- Circle Touches y-axis
- Circle Touches both x-axis and y-axis
- Centre of the Circle on x-axis
- Centre of the Circle on y-axis
- Circle Passes through the Origin and Centre Lies on x-axis
- Circle Passes through the Origin and Centre Lies on y-axis
- Equation of a Circle when Line Segment Joining Two Given Points is a Diameter
- Equations of Concentric Circles
- Circle Passing Through Three Given Points
- Circle Through the Intersection of Two Circles
- Equation of the Common Chord of Two Circles
- Position of a Point with Respect to a Circle
- Intercepts on the Axes made by a Circle
- Circle Formulae
- Problems on Circle
11 and 12 Grade Math
From Equation of a Circle to HOME PAGE
Didn't find what you were looking for? Or want to know more information about Math Only Math. Use this Google Search to find what you need.
Recent Articles
-
Worksheet on Addition of 4-Digit Numbers | 4 Digit Addition Worksheets
Jan 10, 25 03:02 PM
Practice the questions given in the worksheet on addition of 4-digit numbers. Here we will add two 4-digit numbers (without carrying and with carrying) and three 4-digit numbers -
Word Problems on 4-Digit Numbers |Addition and Subtraction of 4-Digits
Jan 10, 25 02:49 PM
We will solve here some of the word problems on addition and subtraction of 4-digit numbers. We will apply the same method while adding and subtracting the word problems. 1. In a village, there are 25… -
Addition of 10, 100 and 1000 | Adding 10 | Adding 100 | Adding 1000
Jan 10, 25 01:20 AM
Here we will learn Addition of 10, 100 and 1000 with the help of different examples. -
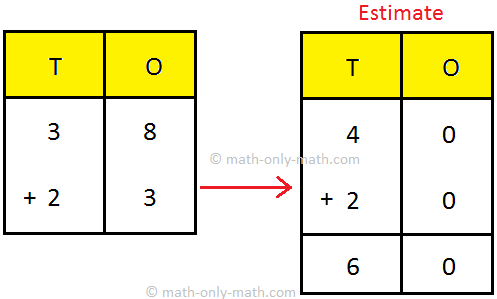
Estimating a Sum | Round the Number | Numbers by Rounding | Estimating
Jan 10, 25 12:10 AM
We will learn the basic knowledge for estimating a sum. Here we will learn an easy way to estimate a sum of two numbers by rounding. In case of two digit numbers we can only round the number -
Addition of 4-Digit Numbers | 4-Digit Addition |Adding 4-Digit Numbers
Jan 09, 25 10:07 PM
We will learn about the addition of 4-digit numbers (without carrying and with carrying). We know how to add 2 or 3, 3-digit numbers without carrying or with carrying.

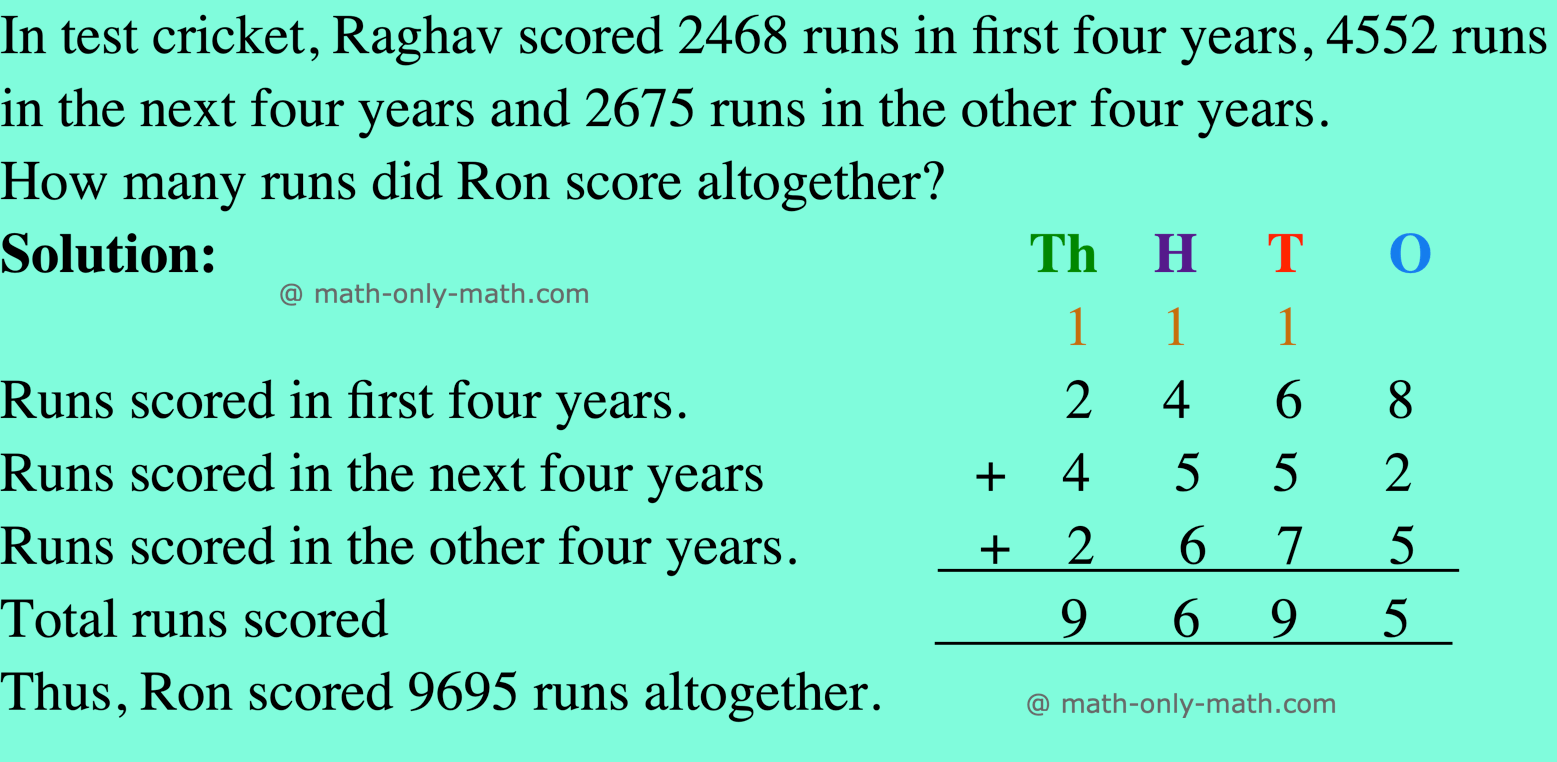


New! Comments
Have your say about what you just read! Leave me a comment in the box below. Ask a Question or Answer a Question.